Blue and white
Identical squares of side one unit contain some circles shaded blue. In which of the four examples is the shaded area greatest?
Problem
Blue and White printable sheet
In the examples below identical squares of side one unit contain some circles shaded blue.

In which of the four examples is the shaded area greatest?
This problem is also available in French: Bleu et Blanc
Student Solutions
We received correct solutions (that the areas were all the same) from:
- Charles Blackham (Shrewsbury House School)
- Michael Brooker (home educated),
- Andrei Lazanu (School number 205, Bucharest),
- Chong Ching Tong, Chen Wei Jian and Teo Seow Tian (River Valley High School, Singapore) and
- Chris Wells (Forres Academy).
Well done all of you.
I particularly liked Michael's solution because he generalised the result and I have used this as the basis of what follows. Although it was not intended to be a trick question Michael!
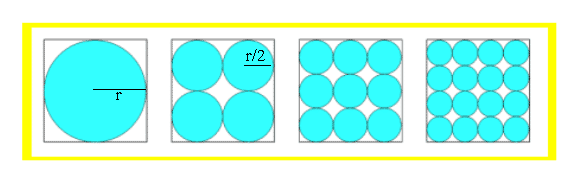
With one circle in the square the diameter is the length of one side of the square. The shaded area is ${\pi}{r^2}$ where $r$ = radius of the largest circle.
With four circles in the square, the diameter of one circle is half that of the large circle. The area of each small circle is ${\pi}({r/2}\: \times \:{r/2})$. The total shaded area is $4({\pi}{r^2}/4)$. This can be simplified to ${\pi}{r^2}$.
With nine circles in the square, the diameter of one circle is a third that of the large circle. The area of each small circle is $\pi(r/3\: \times\: r/3)$. The total shaded area is $9({\pi}{r^2}/9)$. This can be simplified to ${\pi}{r^2}$.
We can go one step further by saying that with $n$ circles the area is $n({\pi}{r^2}/n)$ - which can again be simplified to ${\pi}{r^2}$. Therefore the answer is that the shaded area is the same in each picture.
Teachers' Resources
This printable worksheet may be useful: Blue and White.
