Tiling into slanted rectangles
A follow-up activity to Tiles in the Garden.
Problem
This is seen as a possible follow on from Tiles in the Garden.
This activity takes "Tiles in the Garden", much further. We can keep the main ideas the same -
- Square tiles
- A corner of a tile at each corner of the rectangle
- The ability to slice a tile into parts so as to use each part
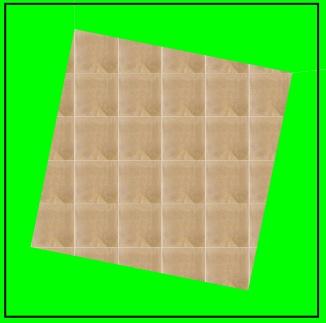
So this one used $26$ and the slope was generated by going along $1$ and up $5$.
This time let's put on a limit of using less than $100$ tiles.
What sizes of rectangles could be filled obeying the three rules?
How many tiles for each rectangle you find?
Are there any numbers of tiles between $10$ and $100$ for which there cannot be a rectangle?
Teachers' Resources
Why do this problem?
Possible approach
Key questions
Possible Extension
It would be good to handing over to the pupil and encouraging the curiosity question "I wonder what would happen if . . . ?"
Teacher Support
This task was created to help in the pursuance of curiosity within the Mathematics lessons.
Help may be found in the realm of curiosity in watching parts of these excellent videos.
Firstly "The Rise & Fall of Curiosity", particularly the extract [23.50 - 37.15] on "adult encouragement answering and teacher behaviour."
Secondly, "The Hungry Mind: The Origins of Curiosity", particularly the extract [8.22 - 12.29] on "Children asking questions"
First can also be found at - https://www.youtube.com/watch?v=X-0NOrIU67w
Second can also be found at https://www.youtube.com/watch?v=Wh4WAdw-oq8
