Walkabout
A walk is made up of diagonal steps from left to right, starting at
the origin and ending on the x-axis. How many paths are there for 4
steps, for 6 steps, for 8 steps?
Problem
Image
Image
Image
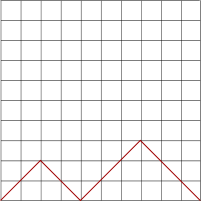
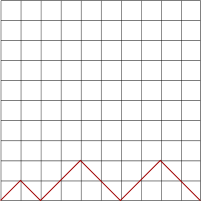
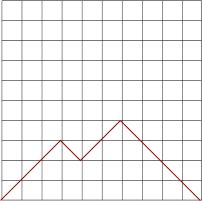
A walk is made up of diagonal steps, starting at the bottom left and ending up back on the bottom line (x-axis). You can move diagonally up and down towards the right but you cannot move towards the left.
A diagonal must go from a left-hand corner of a square to the opposite right-hand corner of the same square.
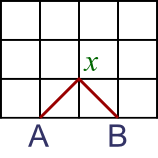
The examples above all show 10-step walks. So let's look at a 2-step walk in more detail:
Image
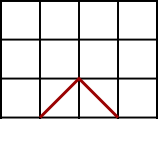
There is only one way to make a 2-step walk - from A up to x and down to B:
Image
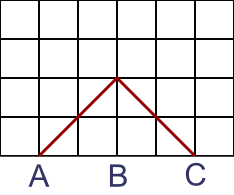
Here are two 4-step walks:
Image
Image
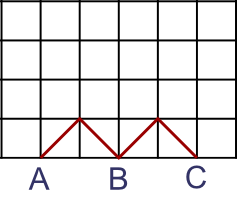
Are there any more 4-step walks?
How do you know you have them all?
How many 6-step, 8-step walks are there?
Can you find a general rule?
See also the problems One Basket and Counting Binary Ops
Getting Started
The 'trick' is finding a systematic way to record all possibilities.
Student Solutions
This one was a tough problem! Some of you started to spot patterns and suggested possible rules, but the patterns didn't continue. In fact, a general rule for this sequence is pretty hard to write down! One very useful way of recording the number of walks of up to eight steps was this:
Image
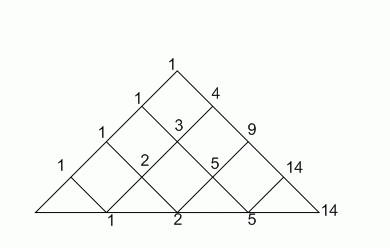
Can you see how the diagram works? Could you continue it to find the number of ten-step walks?
Teachers' Resources
Why do this problem
This
problem presents an investigation which does eventually require
a systematic approach. Although the generalisation is difficult for
Stage 4 some of the context's structure is discernible and
describable, and comparable to other similar situations. Do the
problem in conjunction with Group Photo and ask learners to
describe what is the same about the two situations that could
explain them resulting in the same sequence of Catalan numbers.An
apparent generalisation related to cubes of numbers breaks down and
so the problem offers an opportunity to discuss a danger of
applying inductive reasoning.
Possible approach
One approach is to do this in conjunction with Group
Photo , either following from one to the other, or dividing the
class so that groups work on different problems, or why not use two
classes working on the different problems. The aim would be to
bring the two sets of findings together to discuss why two
apparently quite different situations result in the same
mathematics.
Allow plenty of time to 'play' with the problem, making sense
of what is being counted and how it might be represented.
Encourage ideas that involve systematic approaches, and share
them so that all learners have access to a way into the
problem.
Use results from separate groups to check working.
Key Questions
- Can you describe what is the same about the two problems that might explain the similar mathematical structure?
- What is different about and what is similar to other examples, such as One Step Two Step and Room Doubling that result in a Fibonacci sequence?
Possible support
Group photo can be done with real people and you can start with small numbers. Spend plenty of time trying out, and considering the efficiency of, possible recording methods.Possible extension
Can students make connections between the structures of the two problems that may in part explain the mathematical connections?Notes
$ 1$, $ 1$, $ 2$, $ 5$, $ 14$, $ 42$, $ 132$, $ 429$, $ 1430$, $ 4862$ ,...
The Catalan numbers describe things such as:
- the number of ways a polygon with n+2 sides can be cut into n triangles
- the number of ways to use n rectangles to tile a stairstep shape (1, 2, ..., n-1, n)
- the number of ways in which parentheses can be placed in a sequence of numbers to be multiplied, two at a time
- the number of planar binary trees with n+1 leaves
- the number of paths of length 2n through an n-by-n grid that do not rise above the main diagonal
The Catalan numbers are also generated by the recurrence relation:
$ C_0=1, \qquad C_n=\sum_{i=0}^{n-1} C_i C_{n-1-i}.$
For example, $ C_3=1\cdot 2+ 1\cdot 1+2\cdot 1=5$, $ C_4 = 1\cdot 5 + 1\cdot 2 + 2\cdot 1 + 5\cdot 1 = 14$, etc.
