Follow the numbers
What happens when you add the digits of a number then multiply the result by 2 and you keep doing this? You could try for different numbers and different rules.
Problem
This is your chance to follow some numbers and see where they go!
A simple rule is all you need.
My first suggestion is to add the digits together then multiply (times) by $2$.
The first number that I chose happened to be $56$.
So, let's start:
We add the $5$ and $6$, $5+6=11$
We multiply the $11$ by $2$, $2 \times 11 = 22$, and that's the first part of the journey.
So, where now?
We carry on with this rule:
We add the $2$ and $2$, $2+2=4$
We multiply the $4$ by $2$, $4\times 2 = 8$, and that's the second part the journey.
Now, $8 + 0 = 8$ and $8\times 2 = 16$ and that was the third part.
And, $1 + 6= 7$, and $7\times 2 = 14$
Do the same to $14$ and we get $10$
$10$ leads to $2$, $2$ leads to $4$, $4$ leads to $8$ and we are back to where we got to in the second part of the journey.
If we went on and on and wrote down where we got to after each part we would see something like:
$56, 22, 8, 16, 14, 10, 2, 4, 8, 16, 14, 10, 2, 4, 8, ...$
After exploring that journey it's time to start somewhere new, for example $11$ which goes along like this;:
$11, 4, 8, 16, 14, 10, 2, 4, 8, ...$
Oh! So we are on the same bit as before, a circular bit that goes $2, 4, 8, 16, 14, 10$ and then back to the $2$ again.
Now a new starting place, $96$.
This goes like this:
$96, 30, 6, 12, 6, 12, 6, ...$
Oh! So we now have a smaller circular bit of the journey that goes $6, 12$ then back to the $6$.
I explored further trying to start with each number from $1$ to $99$.
Then I tried similar, but different rules.
I found I needed a big piece of plain paper and used arrows to show the journeys. Here are just some bits of them to tempt you to go further:
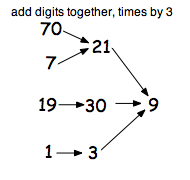
and
Getting Started
What number will you start with?
What could you try next?
What is the same and what is different about the 'journeys' you have tried?
Student Solutions
From Year $4$ at Queen Edith School, Cambridge we had the following rather good idea sent in.
After we had got the idea of following a number on its journey, we split up the work of checking out lots of numbers. Some of us began with numbers in the $30$s, some with numbers in the $40$s, and so on.
We found that all the numbers we tried ended up on one of three journeys:
$2, 4, 8, 16, 14, 10, 2, 4, 8,$ ... which we called the "red" journey
$6, 12, 6, 12,$ ... which we called the "green" journey
$18, 18, 18,$ ... which we called the "blue" journey
Next, we used a $100$ square on the Smartboard, and coloured the numbers to match their journeys. After we had coloured a few of the numbers, some of us spotted patterns beginning to show, like the blue diagonal from $81$ up to $9$. We predicted that other numbers on the diagonal would also be blue and checked them out. We also saw green squares along diagonals and made more predictions.
Finally, we made a display using the 100 square and some of our work to challenge other children to predict the journeys for some of the squares we had not coloured.
Can you predict a journey and then check if you were right?
From Krystof in Prague and Matthew from Hamworthy Middle School we had had similar results. From Karin in West Acton in London we had a clever further idea sent in.
My rule for "Follow the Numbers" is to work out the difference between the $2$ digits and add $5$ to the difference.
Here is some of my "Follow the Numbers"
Starting number:$24 24,07,12,06,11,05,10,06...$
Starting number:$39 39,11,05,10,06,11...$
Starting number:$83 83,10,06,11,05,10...$
Starting number:$63 63,08,13,07,12,06,11,05,10,06... $
On my "Follow the Numbers", most of my numbers had a pattern of $08,13,07,12,06,11,05,10,06.$
Well done Karin, I like this very much, others of you could try your own rules.
Teachers' Resources
Follow the Numbers
This is your chance to follow some numbers and see where they go!
A simple rule is all you need.
My first suggestion is to add the digits together then multiply (times) by $2$.
The first number that I chose happened to be $56$.
So, let's start:
We add the $5$ and $6$, $5+6=11$
We multiply the $11$ by $2$, $2 \times 11 = 22$, and that's the first part of the journey.
So, where now?
We carry on with this rule:
We add the $2$ and $2$, $2+2=4$
We multiply the $4$ by $2$, $4 \times 2 = 8$, and that's the second part the journey.
Now, $8 + 0 = 8$ and $8 \times 2 = 16$ and that was the third part.
And, $1 + 6= 7$, and $7 \times 2 = 14$
Do the same to $14$ and we get $10$
$10$ leads to $2$, $2$ leads to $4$, $4$ leads to $8$ and we are back to where we got to in the second part of the journey.
If we went on and on and wrote down where we got to after each part we would see something like:
$56, 22, 8, 16, 14, 10, 2, 4, 8, 16, 14, 10, 2, 4, 8, ...$
After exploring that journey it's time to start somewhere new, for example $11$ which goes along like this;:
$11, 4, 8, 16, 14, 10, 2, 4, 8, ...$
Oh! So we are on the same bit as before, a circular bit that goes $2, 4, 8, 16, 14, 10$ and then back to the $2$ again.
Now a new starting place, $96$.
This goes like this:
$96, 30, 6, 12, 6, 12, 6, ...$
Oh! So we now have a smaller circular bit of the journey that goes $6, 12$ then back to the $6$.
I explored further trying to start with each number from $1$ to $99$.
Then I tried similar, but different rules.
I found I needed a big piece of plain paper and used arrows to show the journeys. Here are just some bits of them to tempt you to go further:
and
Why do this problem?
Possible approach
Key questions
Possible extension
Possible support
For the highest-attaining