Holly
Problem
The ten arcs forming the edges of this "holly leaf" are all arcs of circles of radius 1 cm. At all the spiky points the circles touch each other tangentially. All the straight lines join the centres of the circles they pass through and the four triangles at the corners have angles of 45, 45 and 90 degrees.
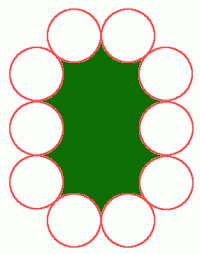
Find the length of the perimeter of the holly leaf and the area of its surface.
This is a 10-spike holly leaf. What is the perimeter of a 16-spike holly leaf of the same sort?
Examine some real holly leaves and you will find that they don't lie flat. This shape is different from a real holly leaf in so far as it does lie flat on a flat surface (or plane). See ' Giant Holly Leaf' for an extension of this problem to a more realistic holly leaf which has negative curvature.
Student Solutions
James Page of Hethersett High School, Norfolk solved the Holly problem, well done James.
Length $AB = 2 + 2\sqrt2$ cm and length $BC = 4 + 2\sqrt 2$ cm.

To find this answer I first took a diagram of the shape and put in the angles. I took 45 from 180 degrees to find the interior angle so I could find the inner curve. I then found that this angle is used 8 times in the diagram. Each circle has a radius of 1 cm and a circumference of $2\pi$ cm. To find the length of the edges of these pieces of the holly I did this:
$
Then there are the two middle ones of which I had to find the circumference and divide by 2 then multiply by 2 for the two of them, giving a total $2\pi$ cm. I then added the two answers to get the perimeter of the holly leaf which is $8\pi = 25.13$ cm (to 2 decimal places).
If you wished to do a 16 spike holly of the same sort the ends would be the same and it would have an extra 3 circles on each side making the perimeter an extra $6\pi$ cm making a total of $14\pi = 43.98$ cm (to 2 decimal places).
The rectangle $ABCD$ has area
From this we have to subtract the areas of the four triangles at the corners, a total area of 4 sq. cm., and also the areas of 8 sectors of circles with angles of 135 degrees and the areas of the two semicircles, in all a total area of $4\pi$ sq. cm. The area of the 10 spike holly leaf is
Teachers' Resources
Possible extension
Try the problem Giant Holly Leaf. It does not require any more mathematical knowledge than this problem but it introduces the concept of curvature.
See also the article Curvature of Surfaces.
