Tangled trig graphs
Can you work out the equations of the trig graphs I used to make my pattern?
Problem
Tangled Trig Graphs printable sheet
Here is a pattern I made with some graphs of trigonometric functions.You can find a copy to print here.
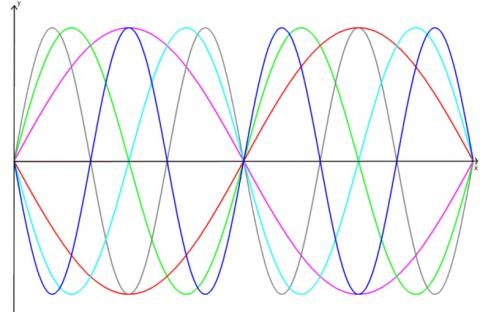
- The purple line is the graph $y=\sin x$. Can you identify the coordinates of the points where it crosses the axes and where it reaches its maximum and its minimum values?
- How could I make the red graph from the purple graph? Can you work out the equation of the red graph?
- The green graph has equation $y=\sin 2x$. Can you describe how to make the green graph from the purple graph? How does the transformation of the graph relate to the way the equation has changed?
- Using these ideas, can you work out the equations of the other graphs I have drawn?
Imagine you had a graphical calculator but the sine button is broken. Can you draw the same patterns using the cosine function instead? Explain how you can transform a cosine graph into a sine graph.
Why not create some trig patterns of your own using graphical calculators or graphing software, and send them to us.
This problem is also available in French: Trigo tricoté
Getting Started
All these graphs have the same maximum and minimum values. What are these? Want does this tell use about the equations of the graphs?
What effect does changing the constant $a$ have on the graph $y=\sin(ax)$?
Student Solutions
This anonymous solver correctly identified the remaining curves, and explained how to draw a graph of sin $x$ using the cosine function:
The red graph has equation $y=-\sin x$.
The green graph has equation $y =\sin 2x$.
The light blue graph has equation $y=-\sin 2x$.
The grey graph has equation $y=\sin 3x$.
The dark blue graph has equation $y=-\sin 3x$.
The graph $y=\cos x$ is the same shape as $y=\sin x$ but shifted along. I can make the shape of $y=\sin x$ by drawing the graph $y=\cos (x-90^{\circ})$.
Teachers' Resources
Why do this problem?
This problem provides a context to explore graphs of trigonometric functions and look at the effects on the graph when the equation is changed. Rather than asking learners to sketch the graphs from the equations, they have to figure out the equation from the graph. The problem gives the opportunity to investigate reflections, stretches and translations of curves, and the corresponding effects on equations, which then can start to generalise.
Possible approach
On plain paper or pupil whiteboards, start by sketching the purple curve $y=\sin x$. Ask learners to mark on the coordinates of any interesting points, such as intersections with the axes and turning points.
Now sketch the green curve and again mark on the key points. Ask pairs to discuss similarities and differences between the purple and green curves, and to consider how to transform one graph into the other.
Reveal the information that the green curve has equation $y=\sin 2x$, and set the challenge of working out the equations of the other curves. Through this, students may begin to conjecture and generalise about the transformations. Graphical calculators or graphing software could be used to experiment with changing the equation and looking at the effect on the graph. After some exploration, encourage students to state what they think the effect will be before they use the graphical calculator or graphing software to test it.
Key questions
What are the interesting features of the graph of $y=\sin x$?
Possible support
Start by investigating transformations of more familiar graphs in Parabolic Patterns and related problems.
Possible extension
Learners could create their own patterns using a combination of sin, cos and tan graphs, and challenge others to work out the equations. Sine problem gives another example of a pattern made from sine graphs which learners could try to recreate.
