Legs eleven
Take any four digit number. Move the first digit to the end and move the rest along. Now add your two numbers. Did you get a multiple of 11?
Problem
Take any four-digit number.
Create a second number by moving the first digit to the 'back of the queue' and moving the rest along.
Now add your two numbers.
I predict your answer will be a multiple of 11...
Try it a few times. Is the answer always a multiple of $11$?
Can you explain why?
Click below to see what Samira noticed:
"I started with 5 thousands, 2 hundreds, 3 tens and 8 units.
After I moved the digits along, my new number had 2 thousands, 3 hundreds, 8 tens and 5 units.
I wonder if this can help me explain what's happening?"
Click below to see what Jay noticed:
"I picked 1000 as my first number, so my second number was 0001 and the total was 1001.
I know 1001 is a multiple of 11 because it is 1100-99, and 1100 and 99 are both multiples of 11."
Do these observations help you to explain what's going on?
What if you start with a three-digit number?
Or a five-digit number?
Or a six-digit number?
Or a 38-digit numbers ... ?
Can you prove your findings?
You may be interested in this article on Divisibility Tests.
Getting Started
Imagine starting with the number $5238$.
We can write it as
$5000+200+30+8$
or
$5\times1000 + 2\times100 + 3\times10 + 8\times1$
Can you express the second number in the same way?
How many 5s, 2s, 3s and 8s are there when you combine the two numbers?

Student Solutions
Lots of people tried out numbers and found that the result was divisible by 11 for 4 digit numbers, and some people tried numbers of other lengths.
| Name | Number | Sum | 11 times table |
| Geon | 3412 | 3412 + 4123 | 7535 = 685$\times$11 |
| Jun | 1000 | 1000 + 0001 | 1001 = 91$\times$11 |
| Mahnoor | 3621 2163 | 3621 + 6213 2163 + 1632 | 9834 = 894$\times$11 3795 = 345$\times$11 |
| Yusuf, Shafi, Adam | 97685437 | 97685437 + 76854379 | 174539816 = 15867256$\times$11 |
| Helen | 34 826 | 34 + 43 826 + 268 | 77 = 7$\times$11 1094 is not divisible by 11 |
| Nabiha | 38 5463 832596 | 38 + 83 5463 + 4635 832596 + 325968 | 121 = 11$\times$11 10098 = 918$\times$11 1158564 = 105324$\times$11 |
| Aadit, Nicole, Jedi | 6953 3484 9876 3527 4567 1000 | 6953 + 9536 3483 + 4843 9876 + 8769 3527 + 5273 4567 + 5674 1000 + 1 | 16489 = 1499$\times$11 8827 = 757$\times$11 18645 = 1695$\times$11 8800 = 800$\times$11 10241 = 931$\times$11 1001 = 91$\times$11 |
As a result of this, Geon from British International School of Hanoi in Vietnam, Nabiha, Helen, Victor, Peter and Edric and Yusuf, Shafi and Adam from Greenacre Public School in Australia and Darcy and Travis from St Stephen's School thought that numbers with an even number of digits would give a sum that was divisible by 11, but numbers with an odd number of digits would not.
Jordan, Ryan, Stephen, Nathan, Juliana and Presley from Monarch Global Academy sent in a solution that gives an insight into why it works for 4 digit numbers:
We agree that all whole four-digit numbers will add to a sum that is divisible by 11. Decimals will not.
For example
1,234
+2,341
The way I found my answer was splitting my numbers into expanded form:
1$\times$1,000 + 2$\times$100 + 3$\times$10 + 4$\times$1
2$\times$1,000 + 3$\times$100 + 4$\times$10 + 1$\times$1
Then I combined the numbers with the same digit, to make multiples of 11:
1,000+1 = 1,001; 200+2,000 = 2,200; 300+30 = 330; 4+40 = 44
All of these sums are multiples of 11, so their sum will also be a multiple of 11. In this situation, the answer was 3,575, which in the end was a multiple of 11.
All of the other examples we tried were too.
You will always have at least two of the same digit in each problem because you are just moving the digits in the original number around.
Thomas, Nathan, Max from Longsands Academy used the same method, but with letters to represent the digits:
First of all, we tried to disprove the statement given. We found out that it was true. After we decided that that it was true we tried symbolising the digits as letters. Starting off with ABCD. We thought about place value that the numbers held and once the place value had been shifted. It looked like this:
ABCD
+BCDA
Place value of letters are:
A=1000's and 1's
B=100's and 1000's
C=10's and 100's
D=1's and 10's
Therefore,
The A will be a multiple of 1001
The B will be a multiple of 1100
The C will be a multiple of 110
The D will be a multiple of 11
Because 1001, 1100, 110 and 11 are all multiples of 11, it does not matter what numbers are being used, as the sum will always be divisible by 11.
Anas from England, Thiago from Unicamp in Brazil, Marcus from Richard Hale, Thomas from Hollinwood Academy, Por Pan from British International School Phuket, Aadit, Nicole and Jedi from Mr McDonagh's math group at Bangkok Patana School in Thailand, and Victoria used a more formal algebraic method.
Victoria made a very clear video, which you can watch here. This is Anas' written work, which also includes 3, 5 and 6 digit numbers:
Let '$abcd$' represent the four digit number. For a four digit number, there is a 'thousands' column, a 'hundreds' column, a 'tens' column and a 'units' column. As the '$a$' is in the 'thousands' column, its actual value it $1000a$.
The '$b$' is in the 'hundreds' column, therefore its actual value is $100b$.
Similarly, the '$c$' is in the 'tens' column and therefore its real value is $10c$.
As the '$d$' is in the 'units' column, its value is just $d$.
So, writing out '$abcd$' algebraically, we get: $1000a+100b+10c+d$
Now, as we add on the number '$bcda$' (as we have to move the numbers from '$abcd$' to the left and therefore the a comes to the 'units' column), we'll do the same as we did for '$abcd$' for '$bcda$': $1000b+100c+10d+a$
We can now add the two algebraic expressions for the integers '$abcd$' and '$bcda$' by collecting like-terms and we'll get:$$(1000a+100b+10c+d)+(1000b+100c+10d+a)\\
=1001a+1100b+110c+11d$$
We can now factor out '$1001a+1100b+110c+11d$' and we'll get:$$11(91a+100b+10c+d)$$
The sum of the two integers '$abcd$' and '$bcda$' is a multiple of $11$ always.
As for the three digit number case, let '$abc$' denote the three digit number. If we write '$abc$' algebraically, we get: $100a+10b+c$
Now writing an algebraic expression for '$bca$', we get: $100b+10c+a$
We now add the two algebraic expressions together to get:$$(100a+10b+c)+(100b+10c+a)
=101a+110b+11c$$
This, however, can't be factored out using $11$ and therefore the answer of the sum of '$abc$' and '$bca$' is not a multiple of eleven.
I will now do the same as has been done above for the previous two examples, except for a five digit number. Let '$abcde$' denote a five digit number. Writing '$abcde$' algebraically, we get: $10000a+1000b+100c+10d+e$
Now, writing out '$bcdea$' algebraically, we will get: $10000b+1000c+100d+10e+a$
Adding the two five-digit integers, we get:$$(10000a+1000b+100c+10d+e)+(10000b+1000c+100d+10e+a)\\
=10001a+11000b+1100c+110d+11e$$
The sum of the integers '$abcde$' and '$bcdea$' can't be factored using $11$ and therefore, the sum of the integers '$abcde$' and '$bcdea$' is not a multiple of eleven.
For the case of a six digit integer, we'll let '$abcdef$' denote the integer. Writing the integer '$abcdef$' algebraically, we get: $100000a+10000b+1000c+100d+10e+f$
Writing the integer '$bcdefa$' algebraically, we get: $100000b+10000c+1000d+100e+10f+a$
Adding '$abcdef$' and '$bcdefa$' together, we get: $$(100000a+10000b+1000c+100d+10e+f)+(100000b+10000c+1000d+100e+10f+a)\\
=100001a+110000b+11000c+1100d+110e+11f$$
This can be factorised to give us: $$11(9091a+10000b+1000c+100d+10e+f)$$
So, the sum of '$abcdef$' and '$bcdefa$' is a multiple of eleven.
I did some other digit-numbers in my own time and I came to conclude that if you're doing the sum for numbers with an even amount of digits, then the answer will be a multiple of eleven. If the numbers have an odd number of digits, then the answer will not be divisible by 11. Therefore, if you used a 38-digit-number, the sum would be divisible by 11.
To show that it is true that this works only for numbers with even numbers of digits, Anny from St Mary's School Cambridge, Oak and Tiffany from British International School Phuket, Tom from King Edward Five Ways and Jack from Ashmole Academy in England looked at the coefficient of $a$. Tom and Jack used formal algebra and a divisibility test for 11 to prove the result. This is Jack's work:
With regards to a general solution for an $n$ digit number, you can, using my previous logic, state that the digits of the number are $a, b, c, d, ..., y, z$ in order.
(Actually, since there are 26 letters in the alphabet, this suggests a 26 digit number, so most mathematicians use $a_1, a_1, a_1, ...a_n$ instead of $a, b, c, ..., z$. However, here we can imagine that there could be any number of letters in the alphabet, as Jack has)
You can also write the number as an expression, in the form: $$10^{n-1}\times a + 10^{n-2}\times b + 10^{n-3}\times c + 10^{n-4}\times d + ... + 10y + z$$
The permutation can also be written in this form as:$$10^{n-1}\times b + 10^{n-2}\times c + 10^{n-3}\times d + 10^{n-4}\times e + ... + 10z + a$$
(by just shifting all of the digits to the left)
By adding these two expressions together and then simplifying, we get the expression:$$a\left(10^{n-1} + 1\right) +b\left(10^{n-2} + 10^{n-1}\right) + c\left(10^{n-3} + 10^{n-2}\right) + ... +z(10 + 1)$$
Now, let us take the expression of $10^{n-2} + 10^{n-1}$. If we express this in a numerical form, we will get the number $11$ followed by $(n-2)$ zeros, as $10^{n-2}$ is just $1$ followed by $(n-2)$ zeros and $10^{n-1}$ is just $1$ followed by $(n-1)$ zeros. Thus we can express $10^{n-2} + 10^{n-1}$ as $11 \times 10^{n-2}.$
In fact, we can do this with every part of the expression above, and can simplify yet again to: $$a\left(10^{n-1} + 1\right) + b\left(11\times10^{n-2}\right) + c\left(11\times10^{n-3}\right) ... + z(11\times1)$$
Or, factoring out the 11: $$a\left(10^{n-1} + 1\right) + 11\left(10^{n-2}b + 10^{n-3}c + ... + z \right)$$
So, as we wish $11$ to go into all of the above expression, all we need to do is prove $10^{n-1} + 1$ is divisible by $11.$ If we were to write this number out, it would be in the form $1$ followed by $(n-2)$ zeros followed by a $1.$ The divisibility rule for $11$ is to take the alternating sum of the digits in the number, read from left to right. If that is divisible by $11$, so is the original number. There are $2$ potential cases here: the digits sum to $0$ or to $2,$ as we can either have the expression $1 - 0 + 0 - 0 ... + 0 - 1 = 0$ OR $1 - 0 + 0 - 0 ... - 0 + 1 = 2.$
As $0$ is a multiple of $11$ ($11\times0$) but $2$ is not, we need to only find cases where $0$ occurs. This ONLY happens when $n$ is even, as when $n$ is even, the last alternating sign HAS to be a $-$. Thus, $10^{n-1} + 1$ is divisible by $11$ iff (IF AND ONLY IF) $n$ is even!
Thus, as two multiples of $11$ sum to a multiple of $11$, we can prove that for an $n$ digit number, WHEN $n$ IS EVEN, if you add the original number to its permutation you will always get a multiple of $11.$ Finally, QED
Tom used a different divisibility test which is described in this article to prove the claim that $10^{n-1}$ is divisible by $11$ only when $n$ is even:
From the NRICH page on divisibility tests, odd powers of $10$ are one less than a multiple of $11$ and even powers of $10$ are one more than a multiple of $11$. So in order for $10^{n-1}+1$ to be a multiple of $11$, $10^{n-1}$ must be one less than a multiple of 11, ${n-1}$ must be odd and therefore $n$ must be even.
Jamie from Bournemouth School in the UK used proof by induction to prove that $10^{n-1}$ is divisible by $11$ when $n$ is even. Jamie almost proves that it is never divisible by $11$ when $n$ is odd - the missing part is explained below.
In fact, this only proves that for $n=1$, $10^{2n}+1$ is not divisible by $11$ - it doesn't prove that it is not divisible by $11$ for larger values of $n$. However, the work needed to prove this is included later in the proof.
Here, this same algebra would show that $\left(10^{2k+2}+1\right)-\left(10^{2k}+1\right)$ is a multiple of $11$, so for the even number case, $f(n+1)$ is divisible by $11$ if and only if $f(n)$ is - and given that, in this case, $f(1)$ is not, $f(2), f(3), ..., f(n)$ won't be either.
Navjot from Sherborne Qatar wanted to prove that $10^n+1$ is divisible by $11$ if and only if $n$ is odd, and began a very brave proof using logarithms. Kevin also bravely attempted to prove the 4 digit case (that rearranging the digits and adding gives a multiple of 11) by induction. Both proofs include very good work, but both have a flaw.
Click here to see Navjot's proof with the flaw explained
Click here to see Kevin's proof with the flaw explained
Well done to both of you for following your curiosity and applying different techniques to create a proof.
Another way to determine when $10^n+1$ (or any other number) is divisible by $11$ it is by using modular arithmetic, which is introduced in this article. To do this, you need to read up to (and including) the section on multiplication, and then try writing the powers of 10 in modulo 11. (You can also use this technique to find out how Jack's divisibility test works).
Teachers' Resources
Why do this problem?
The unexpected result is a great hook that can be used to engage curious students.
Challenging your students to justify and explain their findings will encourage them to develop their understanding of place value and their reasoning skills at the same time.
The problem may be suitable for students to work on in small groups.
Possible approach
Share the animation with students and ask them to describe what they notice.
"I think the answer is divisible by 11. How can we check?"
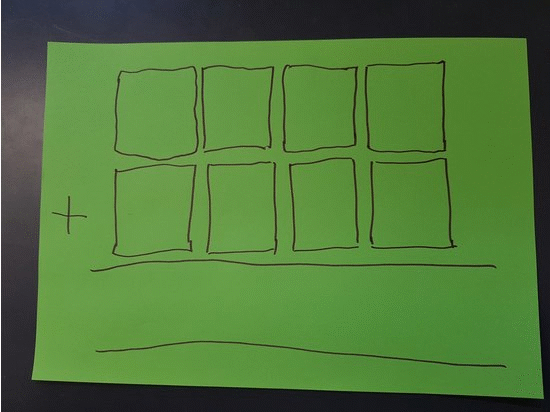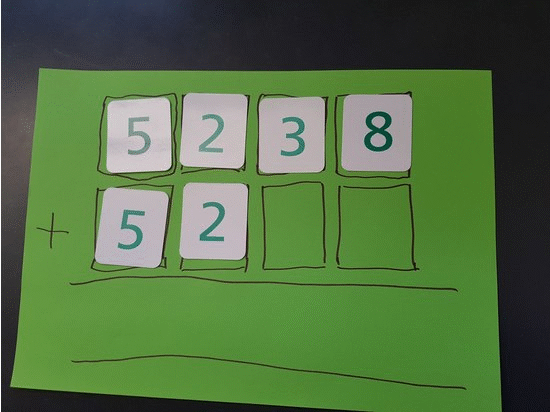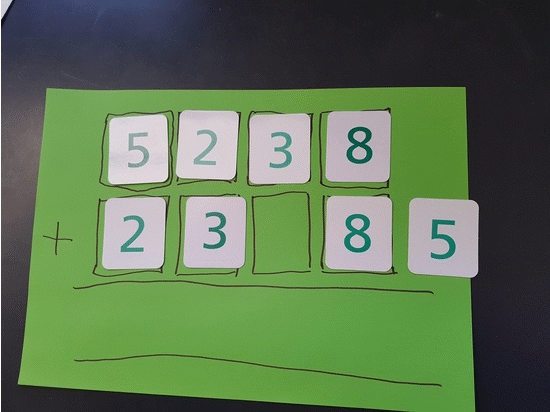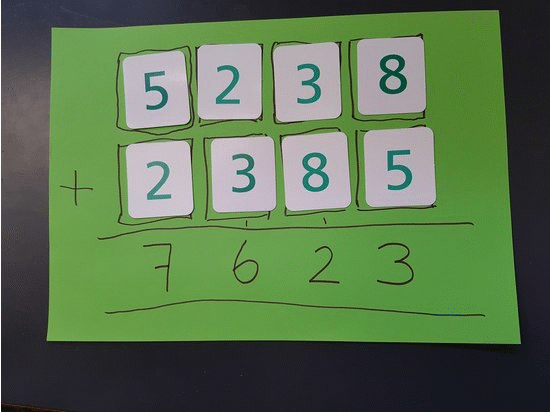
Your students may wish to use a division algorithm here but you may like to draw their attention to the fact that we only wish to confirm that there is no remainder, not find the answer to the division. One way to do this is to subtract known multiples of eleven for example:
7623 - 6600 = 1023
1023 - 22 = 1001
If we can confirm that 1001 is divisible by 11 then we know 7623 is divisible by 11.
"Let's try some more examples...
Choose your own 4 digit number, move the first digit to the back of the queue and add the result to your original number. Check whether your answer is divisible by 11."
This is a good moment for self checking as if anyone thinks they have a counter example you could do it on the board altogether and this can help to sort out any mistakes in their addition strategy or misconceptions about divisibility.
"Will it always work?"
Give students some time to think about this. It might be a good time to work in groups. You could share the prompt below.
"If I started with 5 thousands, 2 hundreds, 3 tens and 8 units.
We could write it as
$5\times1000 + 2\times100 + 3\times10 + 8\times1$
After I moved the digits along, my new number would have 2 thousands, 3 hundreds, 8 tens and 5 units, and could be written as
$2\times1000 + 3\times100 + 8\times10 + 5\times1$
How many 5s, 2s, 3s and 8s would there be when we add the two numbers?"

Your students may come up with a variety of explanations.
One way might be to decompose each number into its place value parts and recombine the parts from the two numbers.
If we can convince ourselves that these are all multiples of 11 we know the total must be a multiple of 11.
Key questions
Will this process always generate a multiple of 11?
How can we prove that it will always work?
Possible support
Possible extension
What if you start with a three-digit number?
Or a five-digit number?
Or a six-digit number?
Or a 38-digit numbers ... ?
After establishing a general proof, students might like to try some similar problems:
