Domino sorting
Try grouping the dominoes in the ways described. Are there any left over each time? Can you explain why?
Problem
Domino Sorting printable sheet
Here are some dominoes taken out of the full set:
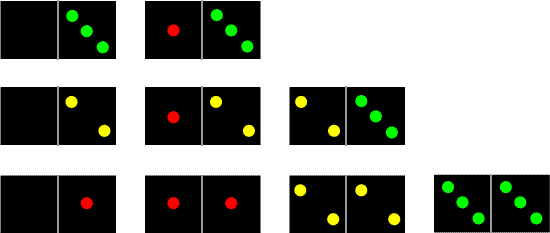
Sort them into two groups - one group with an odd number of spots and one group with an even number of spots.
Do you have any dominoes left over? Why or why not?
Now put the dominoes into pairs. The number of spots on each pair of dominoes must make a total of $5$.
How many pairs can you make?
Which dominoes are left over?
Can you pair them up in any different ways so that each pair adds to $5$?
Which dominoes are left over now?
Are there any dominoes which are always left over?
Can you explain why?
Getting Started
You might find it useful to find some real dominoes to use.
Have you added up or counted the spots on each domino?
Can you think of some pairs of numbers that add to five?
Student Solutions
Natasha and Izzy from Finney Primary School say:
Our solution was that we gave each domino a number going left to right (and top to bottom) . The first domino 1, second 2 and so on. The first solution was the odd numbers (in order 1, 2 so on) were:
So, in other words the dominoes which have an odd number of spots are:

And the rest have an even number of spots. Natasha and Izzy say that none of the dominoes was left over. Rachel from Brynteg School explains that:
All whole numbers are even or odd. The numbers on the dominoes are even or odd so there are no numbers left.
Jack and Peter answered the second part of the problem. They wrote:
Well done, Jack and Peter. There are other ways to make pairs which total 5 and Thomas from Reading explains why:
Teachers' Resources
Why do this problem?
This problem will help learners to become more familiar with odd and even numbers, and number bonds to five. It will also challenge them to justify findings.
Possible approach
If you have an interactive whiteboard, you may find our Dominoes Environment useful for this problem.
- How can you sort them?
- Can you make a pattern?
- Can you make a snake?
- What did you notice?
Key questions
Possible extension
You might want some children to find all the different ways of making pairs that add to $5$. This could be by picking two and then replacing them, or by finding all the different combinations which could be made at the same time (the problem as written focuses on the latter). Whichever way, part of their task should be to convince you that they have not missed any pairs out.
Domino Join Up is a similar problem which gives practice in number bonds to six, and could be used as an extension to this problem.
Possible support
Children would really benefit from having sets of dominoes to manipulate as this allows them to change their mind easily, so giving them more confidence to begin the task, and also prevents them from using any domino twice.
