Domino Join Up
Problem
You will need a double-six set of dominoes for this problem (a standard set of dominoes) but without the seven doubles (double one, double two etc). That is twenty-one domino pieces in all.
Can you arrange fifteen of the pieces round a track like this so that all the touching domino pieces add to $6$ and the ends join up?
You will have six pieces left over.
Using the same twenty-one pieces, can you now make all the joins add to $7$? Which six pieces will you have to leave out?
Can you now make all the joins add to $5$ using the twenty-one pieces? Which six pieces will you have to leave out now?
Now can you make all the joins add to make an even number? What about all the joins adding to make an odd number?
You may find our Dominoes Environment useful for working on this problem.
Printable NRICH Roadshow resources: Instructions and Domino Track.Getting Started
You might find these sheets with a picture of the track and twenty-one domino pieces useful if you don't have a set of dominoes to use.
Don't be afraid to just have a go and to see what happens. You may be able to make some final 'tweaks' later on.
Student Solutions
Sarah, Aleisha, Samuel and Jesse from Rutherglen Primary wrote to say:
We had fun doing this, and it was fairly easy the way we understood it. The hardest part was making sure we had the right twenty one pieces in the first place, because we used some old mixed sets from school. We worked out a great system to check we had what we needed though!Joining to $6$.
This was really easy, we all had lots of fun doing this we all liked it.
Joining to $7$.
This was a bit tricky but we all worked as a team and got it done. We also worked out that the blank ones had to be left out.
Joining to $5$.
This one was easy because we knew that we had to get the sixes out of the road so it can work.
Joining even.
This was easy because we just had to put two even numbers or two odd numbers together and it will make an even number.
Joining odd.
This was easy because we got help off the even one a bit. Then we just kept on working really hard as a team and got it done.
Joining even then odd.
We did this one for a bit of fun and we had lots of fun. We had to work out what will fit together and what won't.
Well done all of you. Tom from Crawley Down Village C of E School told us:
Dominoes adding up to $6$ (which also solves the even number problem):Leave out: $1/4, 5/6, 2/0, 4/3, 2/5, 5/1$
I just tried different combinations.
Dominoes adding up to $7$ (which also solves the odd number problem):
Leave out: $0/1, 0/2, 0/3, 0/4, 0/5, 0/6$
I worked out that you couldn't have dominoes with zeros because it wouldn't add up to $7$.
Dominoes adding up to $5$:
Leave out: $6/0, 6/1, 6/2, 6/3, 6/4, 6/5$
I worked out that you couldn't have dominoes with six because it would be too much.
Good reasoning, thank you Tom. Alex from Heathfield sent in some different solutions:
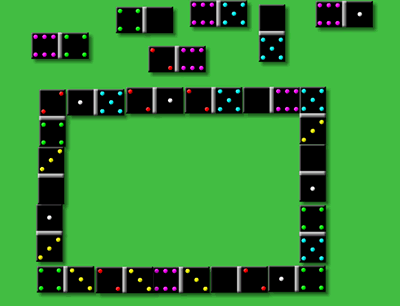
Adding to $6$: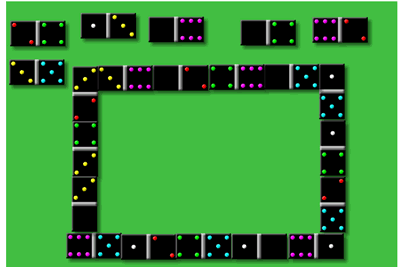
Adding to $7$:
Adding to $5$:
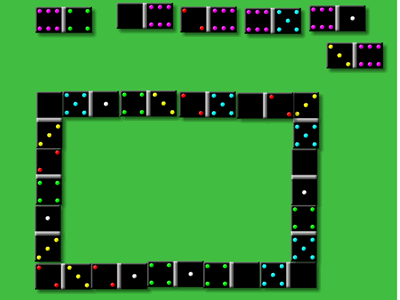
Adding to an even number:
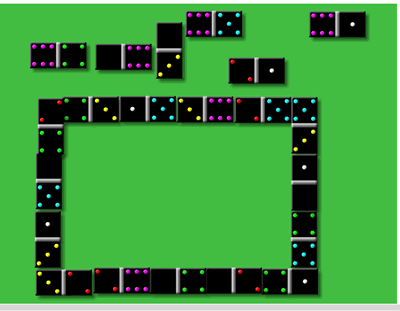
Adding to an odd number:
Teachers' Resources
Domino Join Up
You will need a double-six set of dominoes for this problem (a standard set of dominoes) but without the seven doubles (double one, double two etc). That is twenty-one domino pieces in all.
Can you arrange fifteen of the pieces round a track like this so that all the touching domino pieces add to $6$ and the ends join up?
You will have six pieces left over.
Using the same twenty-one pieces, can you now make all the joins add to $7$? Which six pieces will you have to leave out?
Can you now make all the joins add to $5$ using the twenty-one pieces? Which six pieces will you have to leave out now?
Now can you make all the joins add to make an even number? What about all the joins adding to make an odd number?
You may find our Dominoes Environment useful for working on this problem.