Warmsnug Double Glazing

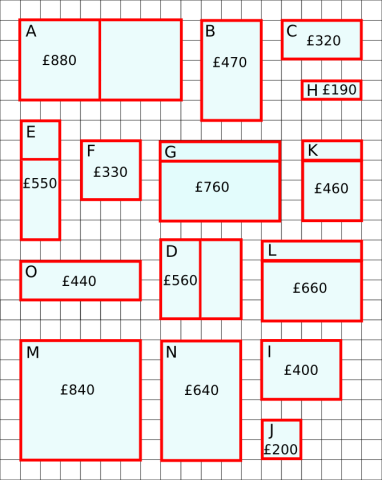
How have Warmsnug arrived at the prices shown on these windows?

Which window has been given an incorrect price?

Explain your reasoning clearly.


The information is available on a printable worksheet here
If you need to, you could look at the hints for ideas of variables that warmsnug might be using.

When you have finished, test your techniques for solving the problem on a new or a harder version. This spreadsheet generates more problems (and harder problems) using different ways of pricing the the warmsnug windows.

Can you find an efficient strategy for solving these problems?


This problem is based on the original Warmsnug Double Glazing problem which appeared in The Language of Functions and Graphs produced by the Shell Centre for Mathematical Education and the Joint Matriculation Board.