Pebbles
Place four pebbles on the sand in the form of a square. Keep adding as few pebbles as necessary to double the area. How many extra pebbles are added each time?
Problem
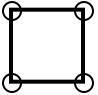
Imagine that you're walking along a sandy beach collecting pebbles. You start off with four pebbles and you place them on the sand as the corners of a square. The area inside your pebbles is just 1 square.
Image

| Image

|
Image

| Image

|
Image

| |
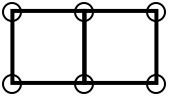
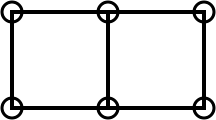
By adding another 2 pebbles you double the area to 2 squares, like this:
Image

| Image

| Image

|
Image

| Image

| Image

|
Image
| ||
The rule is that you keep the pebbles that you already have, not moving them to any new positions, and add as few new pebbles as necessary to double the previous area.
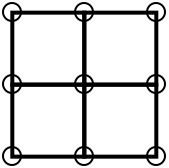
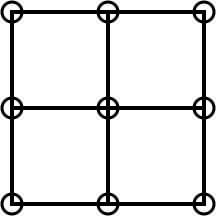
So, to continue, we add another three pebbles to get an area of 4:
Image

| Image

| Image

|
Image

| Image

| Image

|
Image

| Image

| Image

|
Image
| ||
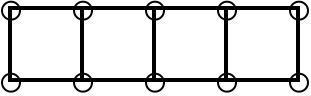
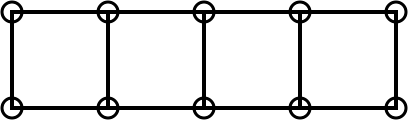
You could have doubled the area by doing this instead:
Image

| Image

| Image

| Image

| Image

|
Image

| Image

| Image

| Image

| Image

|
Image
| ||||
But this would not be allowed because we are adding as few pebbles as possible each time.
The sixth arrangement of pebbles would look like this:
Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

|
Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

|
Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

|
Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

|
Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

| Image

|
Image

| ||||||||
Now it's time for you to have a go.
What do you notice about the arrangements? What questions could you ask about them?
You might like to explore:
How many extra pebbles are added each time? This starts off as 2, 3, 6...
How many are there around the edges? This starts off as 4, 6, 8...
How big is the area? This starts off as 1, 2, 4...
How many pebbles are there inside each arrangement? This starts off as 0, 0, 1, 3, 9...
Don't forget the all-important question to ask - "I wonder what would happen if I ...?''
More Pebbles is a three-dimensional version of this task, which you might like to have a go at afterwards.
Getting Started
You could use cubes or counters for pebbles, with squared paper.
Can you make a rectangle with just one more pebble? Two more? Three more ...?
Have you checked that each rectangle is double the size of the last one?
How are you recording what you have done?
Student Solutions
A group of children from Manorfield Primary School, Stoney Stanton sent in lots of ideas:
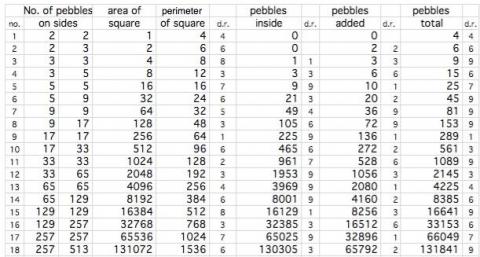
S.B. and N.L. produced the following table of results:
| Number | Shape | Number of pebbles on side | Area of shape | Perimeter of shape |
| 1 | Square | 2x2 | 1cm$^2$ | 4cm |
| 2 | Rectangle | 2x3 | 2cm$^2$ | 6cm |
| 3 | Square | 3x3 | 4cm$^2$ | 8cm |
| 4 | Rectangle | 3x5 | 8cm$^2$ | 12cm |
| 5 | Square | 5x5 | 16cm$^2$ | 16cm |
| 6 | Rectangle | 5x9 | 32cm$^2$ | 24cm |
| 7 | Square | 9x9 | 64cm$^2$ | 32cm |
| 8 | Rectangle | 9x17 | 128cm$^2$ | 48cm |
A.H and E.R also said:
Now, Alice from St Thomas' Church of England Primary School, wrote;
On B (how many pebbles there are round the edges), the pattern starts on 4 adds 2 which makes 6 adds another 2 which is eight then adds 4 which makes 12 adds 4 again (16) adds 8 (24) adds 8 adds 16, and so on.
So it adds one thing twice then doubles the adding number and starts to do that again.
Amelia from NLCS sent in her ideas;
First double forward then double sideways and so on
Pebbles added: 2,3,6,10,20,36,72
Pebbles Outside: 4,6,8,12,16,24,32
Squares: 1,2,4,8,16,32,64,128
Well done all of you - you obviously worked hard on this problem.
Teachers' Resources
Why do this problem?
Use this activity to introduce children to an investigation that mixes both shape and space work with number work. You could also introduce learners to this extended piece of work to help you look at perseverence and persistence.
Possible approach
A good introduction can be had with the whole class by making the first two or three arrangements all together. It is useful to have squared and dotted (squares) paper available whilst some pupils may benefit from using blocks (such as multilink) to represent the pebbles. You may also find it helpful to use a virtual geoboard for sharing ideas amongst the whole group.
Key questions
Possible extension
Some pupils may produce a table or a spreadsheet of their results which would enable them to explore further.
Alternatively, Making Squares is an extension activity.
For more extension work
Go to More Pebbles.
Possible support
Children may benefit from adult support in keeping track of where they are in their exploration. They could be helped to proceed as if it were a game.