Biggest bendy
Four rods are hinged at their ends to form a quadrilateral. How can you maximise its area?
Problem
You may wish to try the problem Bendy Quad first.
Image
Four rods are hinged at their ends to form a quadrilateral with fixed side lengths.
Show that the quadrilateral has a maximum area when it is cyclic.
Getting Started
How would the area change as one of the angles is changed?
You could try splitting the quadrilateral into two triangles. Can you find an expression for the length of the diagonal in two different ways?
Student Solutions
Four rods are hinged at their ends to form a convex
quadrilateral with fixed side lengths. Show that the quadrilateral
has a maximum area when it is cyclic. Sue Liu from Madras College,
St Andrews used two methods to solve this.
|
${\bf METHOD}$ ${\bf 1}$
First she applied Brahmagupta's formula for the area of a
quadrilateral:
$ \delta = \sqrt{(s - a)(s - b)(s
- c)(s - d) - abcd \cos^2 \beta}$
where $ s = \frac{1}{2}(a + b + c + d)$ and $\beta =
\frac{1}{2}(A + C)$ or $ \frac{1}{2}(B + D)$.
Hence the area is clearly the greatest when $abcd \cos^2
\beta$ is least. Since $\cos^2 \beta$ is always positive, this
value is least when $\beta $ is $90$ degrees as $\cos 90^{\circ} =
0$. Hence $\frac{1}{2}(A + C) = 90 $ and so $A + C = 180$ showing
that the opposite angles in the quadrilateral add up to
$180^{\circ}$ and so the area of a quadrilateral with fixed lengths
of sides is greatest when it is cyclic.
This method gives a proof of the required result but you have
to assume Brahmagupta's formula and Sue's second method uses only
the formula for the area of a triangle.
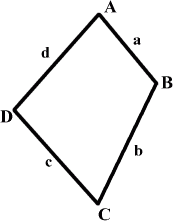
${\bf METHOD}$ ${\bf 2}$ The area of the quadrilateral $ABCD$ can be expressed as the
sum of the areas of triangle $ABD$ and $BCD$. Let the area of
$ABCD$ be $\Delta$. Then
From the Cosine Rule,
Hence, (differentiating both sides with respect to $A$),
From (1) and (2),
We can show that this gives the maximum and not the minimum
value of $\Delta$ by finding the second derivative.
|
Image
|
