You may also like

problem icon

Cushion Ball

The shortest path between any two points on a snooker table is the straight line between them but what if the ball must bounce off one wall, or 2 walls, or 3 walls?

problem icon

Grid Points on Hyperbolas

Find a condition which determines whether the hyperbola y^2 - x^2 = k contains any points with integer coordinates.

problem icon

Parabella

This is a beautiful result involving a parabola and parallels.

Napoleon's Hat

Stage: 5 Challenge Level: Challenge Level:1

hat
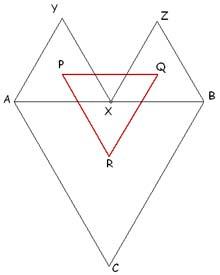
The diagram shows three equilateral triangles $ABC, AYX$ and $XZB$. The point $X$ is a moveable point on $AB$. The points $P$, $Q$ and $R$ are the centres of the three triangles.

Experiment with the dynamic diagram.

What can you say about triangle $PQR$?

Can you prove your conjecture?

Created with GeoGebra

You can download your own free copy of Geogebra from the above link and draw your own dynamic diagrams.
The NRICH Project aims to enrich the mathematical experiences of all learners. To support this aim, members of the NRICH team work in a wide range of capacities, including providing professional development for teachers wishing to embed rich mathematical tasks into everyday classroom practice. More information on many of our other activities can be found here.
Copyright © 1997 - 2012. University of Cambridge. All rights reserved.
NRICH is part of the family of activities in the Millennium Mathematics Project.