Property Chart

Stage: 3 Challenge Level: Challenge Level:2 Challenge Level:2

For this problem, you will need to print off a set of quadrilateral cards . You'll also need a copy of the game grid

Shuffle eight cards, and lay them on the grid in the spaces marked "property card". Your challenge is to draw a quadrilateral in each square, so that the quadrilateral has both the properties at the top of the column and at the start of the row. There might be some that aren't possible! If you like, you could play this with a friend, taking turns to draw shapes. If you can't draw a shape, pass and see whether your partner can, the winner is the one who draws the last shape.

You might find it helpful to draw the shapes on spotty paper; there are squares here and isometric paper here (this might help you find areas and angles).

Picture of board and some cards



You could play the game using triangle cards instead.


Now you've had a go at the challenge, here are some questions you could think about. Use the quadrilateral cards for these.

Can you select 8 cards and arrange them so that you can fill in all of the squares? What cards did you use? What about none of the squares?

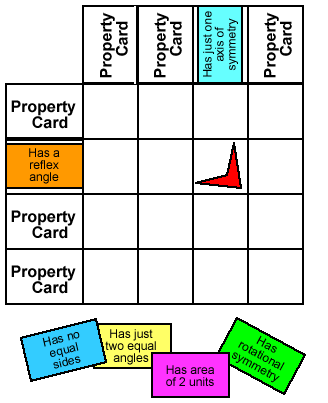
What's the smallest number of different shapes you need to fill in the grid? What shapes are these, and what cards did you use?

Quadilateral cards
This problem is based on the game Nine Pin Shape Draw from "Geometry Games", a photocopiable resource produced by Gillian Hatch and available from the Association of Teachers of Mathematics
search engine page

Published September 2005.