Giant holly leaf
Problem
|
|
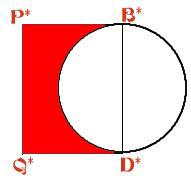
This model holly leaf is made in sections and joined together. Like a real holly leaf, it will not lie flat. It has negative curvature. To make the holly leaf, a circle centre $C$ of radius 5 cm and radii $CA$ and $CB$ with $\angle ACB = 125$ degrees are drawn. The tangents to the circle at $A$ and $B$ meet at the point $P$. Eight identical 3 sided shapes are made by cutting along $PA$ and $PB$ and around the arc $AB$ to make a 3 sided shape with 2 straight edges and one edge along the minor arc of the circle (the circles are thrown away). Two identical 4-sided shapes are made by drawing a circle with radius 5 cm, a diameter $B$*$D$* and tangents $B$*$P$* and $D$*$Q$*equal in length to $PB$. These shapes have edges $B$*$P$*, $P$*$Q$*, $Q$*$D$* and the semicircular arc (inside the rectangle) from $B$* to $D$*. The sketch shows (on a smaller scale) how the ten pieces are joined together to make the "holly leaf". Find the length of the boundary of the yellow area around $P$ which is bounded by six arcs centred at $P$, each of radius $r$ cm. All points on the boundary of the yellow region are equidistant from the point $P$. If the surface at $P$ were flat, the boundary of the region would be a circle and its length would be $2\pi r$. In this case the length of the boundary is greater than $2\pi r$ and the surface of the "holly leaf" has negative curvature at $P$. Compare the perimeter and area of this "holly leaf" with the similar flat leaf for which $\angle ACB = 135$ degrees. See the problem "Holly" for the flat version of this problem. What happens to the holly leaves as the angle $\angle ACB$changes? [For positive curvature the boundary is less than $2 \pi r$ in length.] |
See the article Curvature of Surfaces to find out more about this subject.
Getting Started
First find the angle $\angle APB$.
Student Solutions
The following solution is based on the work of Ben and Sarah. Well done to both of you.
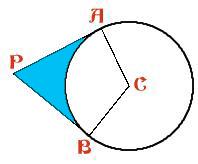
The boundary of the holly leaf can be divided into sections. There are two sections made up from arcs of a circle like the first diagram (above) and there are eight arcs like the second diagram above. So that boundary can be calculated by finding the sum of the lengths of these arcs
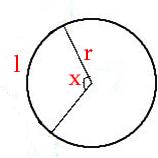
The length of an arc can be calculated by the following formula:
$l$ = $rx$ ($x$ measured in radian)
The parameter of the holy leaf is formed by 8 arcs subtended by angle ACB and 2 semi-cirles .
If $\angle ACB$ = 125 degrees, the parameter of the holy leaf is:
8 $\times$ (5 $\times$125$\pi$/180) + 2 $\times$ 5 $\times$ $\pi$ = 340$\pi$/9 = 118.68 cm
If$\angle ACB$ = 135 degrees, the parameter of the holy leaf is:
8 $\times$ (5 $\times$ 135$\pi$/180) + 2 $\times$ 5 $\times$ $\pi$ = 40$\pi$ = 125.66 cm > 118.68cm
The area of the holy leaf is formed by 8 blue and 2 red areas.
Blue area = 2 $\times$ area of APC - area of the sector corresponding to the angle ACB
Area of APC = $\frac{1}{2}$ AP.AC
Because $\tan$ ($\angle ACP$) = AP/AC, AP = AC. $tan \angle ACP$
Also$\angle ACP$ =$\angle ACB$/2
Hence area of APC = $\frac{1}{2}$ (AC. $tan (\angle ACB$/2)).AC =$\frac{1}{2}$ ($r^2$ $tan (\angle ACB$/2))
Area of the sector subtended by x degrees = $\pi$ $r^2$ $\times$ ($x/360$)
So the area of the sector subtended by angle ACB = $\pi$ $r^2$ $\times$ ($\angle ACB$ $/360$)
So the blue area = 2$\times$ $\frac{1}{2}$ ($r^2$ $tan (\angle ACB$/2)) -$\pi$ $r^2$ $\times$ ($\angle ACB$ $/360$)
Red area = area of rectangle P*B*D*Q* - area of semi-circle = AP.(2r) -$\pi$ $r^2$/2 =
AC. $tan (\angle ACB$/2)$\times$(2r) - $\pi$ $r^2$/2 = $r^2$ $tan (\angle ACB$/2) -$\pi$ $r^2$/2
So the area of the holy leaf is:
8 $\times$ ($r^2$ $tan (\angle ACB$/2) - $\pi$ $r^2$ $\times$ ($\angle ACB$ $/360$)) + 2 $\times$ ($r^2$ $tan (\angle ACB$/2) - $\pi$ $r^2$/2)
= 12$r^2$ $tan (\angle ACB$/2) - $\pi$ $r^2$ ($\angle ACB/45$ + 1)
If$\angle ACB$ = 125 degrees, the area of the holy leaf is:
12 $\times$ $5^2$ $tan (125$/2) - $\pi$ $5^2$ ( 125/45 + 1) =279.59 $cm^2$
If$\angle ACB$ = 135 degrees, the area of the holy leaf is:
12 $\times$ $5^2$ $tan (135$/2) - $\pi$ $5^2$ ( 135/45 + 1) = 410.10 $cm^2$ > 279.59 $cm^2$
From our findings, some conclusions can be drawn as follows:
The boundary of the yellow area is greater than 2r$\pi$, which means the surface of the holy leaf has negative curvature.
As the angle ACB increases ($\angle ACB$ < 180 degrees), the curvature of the surface will increase; both parameters and areas of the holy leaf will also increase.
Teachers' Resources
Why do this problem?
The problem offers practice in calculating arc length and areas of sectors of circles. It introduces the concept of negative curvature and what better way to meet a new mathematical concept than finding out about it for yourself through a practical experiment and investigation? Curvature is a very important concept in mathematics and cosmology and this problem uses very simple school mathematics to introduce the key idea about curvature though curvature is not on school syllabuses. The negative curvature of say a banana as opposed to the positive curvature of an orange is easy to understand and, though more abstract, students may already have heard about curved space.
Possible approach
You could have the class do a 'thought experiment' or actually take a banana and a satsuma into class, and peel them doing as little damage to the peel as possible, then get one of the students to try to flatten the peel. They will find that the satsuma splits because there is less than a 'circumference' of $2\pi r$ for any circle of radius $r$, while it is impossible to flatten the banana skin because circles of radius $r$ have circumferences of more than $2\pi r$. [A 'circle' is defined here as a set of points equidistant from the centre.]
