Kissing

Congratulations Tom Davie, Mike Gray and Ella Ryan of Madras College for your excellent team work on this problem. This is their solution; Tom wrote up the first part, Mike solved the equation and showed that there are two possible circles, and Ella described the construction of the smallest circle. Work like this is a real pleasure to read.

Let the radius of the small circle be $r$ and the radius of the larger circles be $R$. From the two triangles in the diagram formulae for $h$ can be found: $$h = r + \sqrt{(R + r)^2 - (R - r)^2} = r + 2\sqrt{rR}$$ and $$h = R + R\sqrt 2.$$ Hence This is a quadratic equation giving $r$ in terms of $R$. Using the quadratic formula: $$r = {1 \over 2}\big((3 + \sqrt 2)R \pm \sqrt{(-2(3 + \sqrt 2)R)^2 -4 \times 1 \times (3 +\sqrt 2)R^2}\big )$$ Simplifying this expression gives: $$r = R(3 + \sqrt 2 \pm 2\sqrt{2 + \sqrt2)})$$ The two solutions give radii, $r_1$ and $r_2$, of two circles, $C_1$ and $C_2$, which touch both circles of radius $R$ and also touch the tangent lines as shown in the diagram.



$$r_1 = R(3 + \sqrt 2 - 2\sqrt{2 + \sqrt2)})$$ $$r_2 = R(3 + \sqrt 2 + 2\sqrt{2 + \sqrt2)}).$$ Constructing the small circle. The small circle has radius $r$ (given in terms of $R$). $$r = R(3 + \sqrt 2 - 2\sqrt{2 + \sqrt2)})$$ To construct it you will need a pencil, compasses and straight edge.

1. Set your compasses to the size of the radius of the larger circles (length $R$) ).
   
   
   
2. Find $\sqrt 2 R$:
   
   
   • take 2 lengths of $R$
   • construct the perpendicular bisector of this line
   • mark a length $R$ on the bisector
   • the resulting diagonal has length $\sqrt 2 R$
   
   
   
   
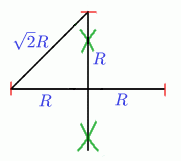
3. Find $3R$ :
   • draw 3 lengths of $R$
   
   
   
   
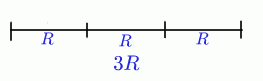
4. Find$(\sqrt{2 + \sqrt 2})R$
   Ella's method uses the intersecting chord theorem: $x^2 = PA\times PB$
   
   
   
   • draw line $AB$ of length$R +2R + \sqrt 2 R$
   • mark the point$P$ such that$PA = R$
   • draw the circle on $AB$ as diameter
   • draw the chord perpendicular to $AB$ through $P$
   • if this chord has length $2x$ then, by the intersecting chord theorem, $$x^2 = R \times (2R + \sqrt2 R)$$ hence $$x = \sqrt{(2 + \sqrt2)R}.$$
     
     
5. Find $2(\sqrt {2 + \sqrt2})R$
   • draw two lengths of $x$
   • 
     
6. Find $r$
   • subtract$2(\sqrt {2 + \sqrt 2})R$ from$\sqrt 2 R + 3R$
   
   
   
   
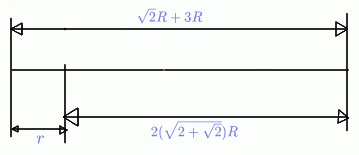
7. Find the centre, $S$ of the small circle
   
   
   
   • set the compass to length to$r$
   • mark off the length$r$ from the origin on both axes, giving the points where the circle touches the axes
   • draw two arcs of radius $r$from these points, then the point where the arcs intersect is the centre$S$ of the small circle
   • finally draw the circle centre $S$ radius $r$

APPENDIX 1 - DRAWING PERPENDICULAR LINES AND FINDING THE MIDPOINT OF A LINE

• set your compass to any length, retaining it throughout
• draw arcs above and below the line from each end of the line
• where the arcs intersect are two points on the perpendicular line
• where the perpendicular line and the original line cross is the midpoint.