Squareness
The family of graphs of x^n + y^n =1 (for even n) includes the
circle. Why do the graphs look more and more square as n increases?
Problem
Image
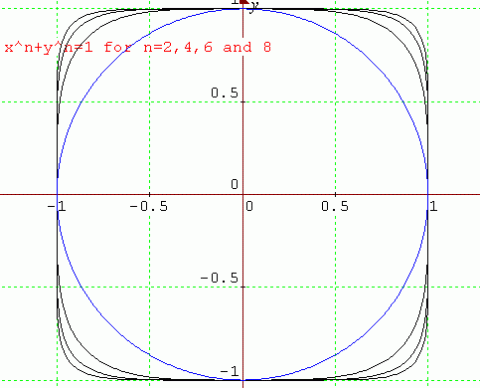
This question is about the family of relations given by $x^n+y^n=1$
(1) Explain the features of the graph of the relation $|x|+|y|=1$.
(2) Prove that
(3) Consider the family of relations $x^n+y^n=1$ in the first quadrant.
Choose one particular value of $n$ and show that $y$ decreases as $x$ increases.
Show that, for each value of $n$, the graph lies entirely outside the square bounded by the lines
(4) Sketch some graphs in all four quadrants of the family of relations $|x|^n+|y|^n=1$ for even values of $n$ and explain why the graphs get closer to a square shape as $n\to \infty$.
(5) Plot the graph of $x^3+y^3=1$ in all four quadrants. Why do the graphs of the relations $x^n+y^n=1$ differ according to whether $n$ is odd or even?
Getting Started
(1) Consider each quadrant separately or use a symmetry argument.
(2) Write $2^{1/n} = 1 + \delta$ and use the Binomial Theorem.
(3) Consider the symmetry of the graph and the point at which it cuts the line $y=x$.
(4) Use the result of (3) and a symmetry argument.
(5) Consider each quadrant separately.
Student Solutions
Image
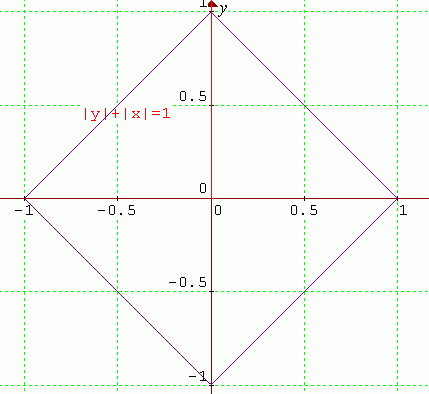
This solution was submitted by Curt from Reigate College. Well done Curt.
(1) Explain the features of the graph of the relation $|x|+|y|=1.$
If the above equation is true then neither $|x|$ nor $|y|$ can be greater than 1. If say $1+h =|x| > 1$, we have: $1+h + |y| =1$ and so $|y| = -h < 0$, a contradiction, as $|a|\geq 0$ for any $a$. Therefore, this function is not defined for values of $x$ and $y$ greater than 1.
I now quote without proof the fact that if $|a| = t$ then $a =t$ or $a= -t$. This is useful when applied in this problem.
If $|y| = 1- |x|$ then $y = 1 - |x|$ or $y = -1 + |x|$. If $y = 1- |x|$ then $y= 1 + x$ or $y= 1-x$. Similarly, if $y = -1 + |x|$, then $y=x-1$ or $y=-x-1$.
The graph is the line segments (where $-1< x< 1$ and $-1< y< 1$) given by: $y=1-x$ in the first quadrant; $y=1+x$ in the second quadrant; $y=-1-x$ in the third quadrant and $y=x-1$ in the fourth quadrant.
Editor's note: By symmetry if $(a,b)$ satisfies this relation then so does
The line segments are perpendicular and intersect at (0,1), (-1,0) (0,-1) and (1,0). The square shape with vertices at the above co-ordinates, has symmetry in $y=x$, $y= -x$, $y=0$ and $x=0$. I refer to this as a graph, not a function, due to one-to-many relation.
2) As $n/(n+1)$ can be written as $1/(1+1/n)$, by dividing both numerator and denominator by $n$ we consider:
(3) Taking the simple case $x^2 + y^2 = 1$ (the circle), and considering the first quadrant, it will be shown that $y$ decreases as $x$ increases. As this is in the first quadrant, the positive root alone is considered: $y = (1-x^2)^{1/2}.$ As $x$ increases to a maximum value of 1 on the interval $0 \leq x \leq 1$ so $x^2$ increases monotonically and $f(x)=1-x^2$ decreases monotonically (to confirm, check f'(x)=-2x).
If we have $f(x_1)> f(x_2)$ (with $x_1< x_2$), then taking the positive square root of both sides, we have ${f(x_1)}^{1/2} > {f(x_2)}^{1/2}$. So as $x$ increases, $y$ decreases.
This same method could be extended to all values of $n$. For $g(x) = 1-x^n$ then $g(x+h)< g(x)$ for $h> 0$ because $x^n$ increases monotonically on the interval $0< x< 1$. Clearly, if $x_1< x_2 $ then $x_1^n< x_2^n$ so $1-x_1^n> 1-x_2^n$ and hence $g(x_1)> g(x_2)$. Taking the positive nth root of both sides, we get: $y_1 = {g(x_1)}^{1/n}> {g(x_2)}^{1/n}=y_2$. So $y_1 > y_2$ for $x_1 < x_2$ ; hence as $x$ increases in the first quadrant, $y$ decreases.
Editor's note: Alternatively we can use calculus to prove this. Fixing one value of $n$ in $x^n+y^n=1$:
When $x=y$, on the general graph $x^n + y^n = 1$ we get $2x^n=1$ so $x=y=1/(2^{1/n})$. From earlier in this question, we have for $n> 1$,
Image

(4) As $n \to \infty$, $1/(1+1/n) = n/(1+n) \to 1$.
The square which the curve must lie within is fixed (as above). However, as $n$ increases, the square which the curve must lie outside of gets increasingly large. As the curve is squeezed between two squares, its shape becomes increasingly more squared.
For curves where $n$ is even, the curve becomes squeezed in all four quadrants because, by symmetry (exactly as in part (1) of this question) the graph is symmetric about $y=x$, $y=-x$, $y=0$ and about $x=0$.
Image
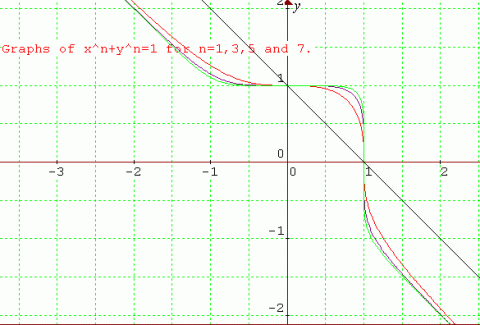
(5) In the first quadrant the graph of the relation $x^3 + y^3
= 1$ behaves similarly to other graphs where $n$ is even. However,
the graph cannot be defined in the third quadrant for $x< 0$ and
$y < 0$, for that would lead to the sum of two negative numbers
being 1, which is not possible. The function is also defined for
$x> 1$, for negative numbers do have real cube roots. If $x^3 =
1+a$ then $y^3 = -a$ and so $y=-a^{1/3}$ and $x = (1+a)^{1/3}$. For
large $x$ (large $a$) this shows that $x \approx -y$, therefore the
graph reaches an asymptote with $y= -x$. The same thing happens as
$x \to -\infty$ in the second quadrant.
The same features occur for the whole family of graphs of $x^n
+ y^n=1$ for odd $n$.
The graphs of $|x^n| + |y^n| = 1$ for odd $n$ would have the
symmetrical squarish shape, reflecting the family of graphs in the
first quadrant into the remaining quadrants (see part 1).
Teachers' Resources
This problem calls for you to describe and explain the features of the graphs and symmetry arguments will be very useful. First think about the features of the graphs and try to sketch them for yourself, then it may help to use a graphic calculator or graphing software.
