Cubestick
Stick some cubes together to make a cuboid. Find two of the angles
by as many different methods as you can devise.
Problem
Glue three cubes together to make a cuboid of dimensions $3$ by $1$ by $1$. Label $4$ points on the cuboid with coordinates $A = (0,0,0)$, $B=(1,1,1)$, $C=(2,1,0)$ and $D=(3,1,0)$. Now the challenge is to find at least two different ways to calculate the angles $\angle ABC$ and $\angle ABD$.
Getting Started
Pythagoras Theorem will give you all the important lengths here.
If you know the lengths of 3 sides of a triangle you can find the angles!
Or you could use vectors and scalar products.
If you know the lengths of 3 sides of a triangle you can find the angles!
Or you could use vectors and scalar products.
Student Solutions
The solution to this tough nut was submitted by Ray who does not reveal his school.
By Pythagoras' Theorem:
Using the cosine rule: $|AD|^2 = |AB|^2 + |BD|^2 - 2|AB||BD|\cos B.$ So
The challenge was to find more than one method so the second method uses geometry and vectors.
Image
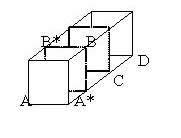
|
Triangle $A^*BC$ is a right angled isosceles triangle and $A^*B$ is perpendicular to $BC$.The line $B^*B$ is perpendicular to the plane $A^*BDC$ so it is perpendicular to $BC$. As the two line $A^*B$ and $B^*B$ are perpendicular to $BC$ the whole plane $AB^*BA^*$ is perpendicular to $BC$. Hence $AB$ is perpendicular to $BC$. |
Using vectors :
Teachers' Resources
Once you have solved a problem it's instructive to try to think of another (perhaps better?) method of solution. On one famous occasion I did not expect different methods but two school students sent in not 2 but 8 different solutions of the same problem. Not content with that these two students then generalised the problem.
See Eight Methods for Three by One and also Why stop at Three by One ?
Can you beat that? I expect their teacher at Madras College will remember it. Perhaps that school can score again, but what about other schools? This is a challenge calling for the combined efforts of a group of students or a whole class.
