Consecutive seven
Can you arrange these numbers into 7 subsets, each of three numbers, so that when the numbers in each are added together, they make seven consecutive numbers?
Problem
Consecutive Seven printable worksheet
Start with the numbers from $0$ - $20$:
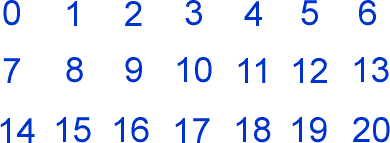
Can you arrange these numbers into seven sets of three numbers, so that the totals of the sets are consecutive?
For example, one set might be $\{2, 7, 16\}$
$2 + 7 + 16 = 25$
another might be $\{4, 5, 17\}$
$4 + 5 + 17 = 26$
As $25$ and $26$ are consecutive numbers these sets might be part of your solution.
Once you've found a solution, here are some questions you might like to consider:
- Is there more than one possible set of seven consecutive totals? How do you know?
- Is there more than one way to make the seven totals?
- Could you make seven sets that all had the same total?
- Could you make seven sets whose totals went up in twos? Or threes? Or...
Getting Started
Consecutive numbers are numbers which follow each other when you are counting, for example, $4$, $5$, $6$, $7$ or $19$, $20$, $21$, $22$, $23$.
What is the total of all the numbers from $0$ to $20$?
Student Solutions
Many of you approached this problem in the same way to start with. Maddie and Alex from The Mount School wrote:
We found out that 1+2+3+4+5+6...+20=210. Because we needed 7 different subsets that when added together made 7 consecutive numbers, we divided 210 by 7. 210/7=30
This told us that the seven numbers had to be around 30. The numbers turned out to be 27, 28, 29, 30, 31, 32 & 33.
We tried a variation of numbers but ended up using:
$$
\begin{align}
0+7+20&=27\\
6+10+12&=28\\
2+8+19&=29\\
5+9+16&=30\\
3+11+17&=31\\
1+13+18&=32\\
4+14+15&=33
\end{align}
$$
This is one of many solutions!
Well done, Maddie and Alex. In fact, not many of you mentioned that there are lots of solutions to this problem. Boris from Gresham's Preparatory School found the seven consecutive numbers in a similar way and then said:
So now we check which numbers from 0 to 20 can make them in groups of 3:
27 - 7,3,17
28 - 8,5,15
29 - 19,4,6
30 - 9,10,11
31 - 1,18,12
32 - 2,16,14
33 - 0,13,20
To find these it's easier to make two of the numbers end with a zero (e.g. 3+17=20, 5+15=20, 4+6=10, 9+11=20, 18+12=30, 16+14=30, 0+20=20) and then add the other number to finish it.
Thank you, Boris. Many of you suggested ways to make pairs of numbers and then add a third to total one of the consecutive numbers like Boris' method.
Ivo from Gresham's Prep School used a different method to work out which consecutive numbers to aim to make:
Thank you, Ivo! Zoe, Andrew, Nikita and Ben from Aqueduct Primary School went about the problem in a slightly different way:
2+7+16 = 25
| A | B | C | Answer |
| 2 | 7 | 16 | 25 |
| 4 | 5 | 17 | 26 |
We noticed that the pattern in A = adding 2 each time
We noticed that the pattern in B = subtract 2 each time
We noticed that the pattern in C = adding 1 each time
We followed the pattern on to see if we could come up with 7 sets however, we only managed to achieve 5.
Here are our results:
0 + 9 + 15 = 24
2 + 7+ 16 = 25
4 + 5 + 17 = 26
6 + 3 + 18 = 27
| 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| 7 | 8 | 9 | 10 | 11 | 12 | 13 |
| 14 | 15 | 16 | 17 | 18 | 19 | 20 |
| A | B | C | Answer |
| 0 | 7 | 14 | 21 |
| 1 | 8 | 15 | 24 |
| 2 | 9 | 16 | 27 |
| 3 | 10 | 17 | 30 |
| 4 | 11 | 18 | 33 |
| 5 | 12 | 19 | 36 |
| 6 | 13 | 20 | 39 |
This did not give us consecutive answers. The answers increased by 3 each time. We looked more closely at our lists of numbers and realised that although the numbers in each list were consecutive, the lists were not consecutive to each other. We rearranged the lists:
| A | B | C | Answer |
| 0 | 13 | 14 | 27 |
| 1 | 12 | 15 | 28 |
| 2 | 11 | 16 | 29 |
| 3 | 10 | 17 | 30 |
| 4 | 9 | 18 | 31 |
| 5 | 8 | 19 | 32 |
| 6 | 7 | 20 | 33 |
This did give us consecutive answers.
We noticed that the numbers in columns A and B when added together all give the same answer, for example:
0 + 13 = 13
1 + 12 = 13
2 + 11 = 13
3 + 10 = 13
4 + 9 = 13
5 + 8 = 13
6 + 7 = 13
Thank you Zoe, Andrew, Nikita and Ben. It is always good to receive solutions which take us all the way through the process that you followed to solve the problem. Your solution shows us that "playing" with a problem can be a very good way to start and will often lead to us finding something out that helps us go about a solution more systematically (in other words more logically).
Rhiannon was able to give a set where the totals were all the same, as well as ones where the totals went up in twos and threes.
The numbers have a total of $0+1+2+...+19+20=210$, so, if there are seven sets of three numbers with the same total, these must each have a total of $210 \div 7 = 30$.
If there is one number from the bottom row in each set, these are $14$, $15$, $16$, $17$, $18$, $19$ and $20$. This means the other numbers in the sets need to have totals of $16$, $15$, $14$, $13$, $12$, $11$ and $10$.
If $0$ is used to make the smallest of these totals, this means $0+10=10$.
To make $11$, this total needs to be increased by $1$. Increasing either of the numbers by $1$ is impossible, as the other number would be used twice. Therefore I tried increasing one of the numbers by $2$ and decreasing the other number by $1$. Since $-1$ is not available, the next pair becomes $2+9=11$.
This can be repeated to give $4+8=12$ and $6+7=13$.
Repeating this would give $8+6=14$, but $6$ and $8$ have already been used. The unused numbers are $1$, $3$, $5$, $11$, $12$ and $13$, so using the same idea gives $1+13=14$, $3+12=15$ and $5+11=16$.
Recombining these with the other numbers in order gives:
$0+10+20=30$
$2+9+19=30$
$4+8+18=30$
$6+7+17=30$
$1+13+16=30$
$3+12+15=30$
$5+11+14=30$
If instead the totals are going up in twos, then these must be $24$, $26$, $28$, $30$, $32$, $34$ and $36$, since the average value must still be $30$.
Again using one of the bottom row of numbers in each case and in the same order, the remaining totals needed are $24-14=10$, $26-15=11$, $28-16=12$, $30-17=13$, $32-18=14$, $34-19=15$ and $34-16=20$. These are the same totals that were used above with the numbers from the top two rows, so the sets become:
$0+10+14=24$
$2+9+15=26$
$4+8+16=28$
$6+7+17=30$
$1+13+18=32$
$3+12+19=34$
$5+11+20=36$
To make the totals increase by threes the columns of the grid can be used, as each of the three numbers is increased by $1$ for each set, so the total increases by $3$ each time. This means the sets are:
$0+7+14=21$
$1+8+15=24$
$2+9+16=27$
$3+10+17=30$
$4+11+18=33$
$5+12+19=36$
$6+13+20=39$
Thank you and well done to everyone who submitted solutions!
Teachers' Resources
Why do this problem?
This problem has several different solutions. The problem can be solved using an experimental / trial and error approach but some consideration of the structure can lead to more efficient solution techniques. There will be no need for students to feel 'stuck' on this problem: they will always be able to experiment with new combinations.
Possible approach
This printable worksheet may be useful: Consecutive Seven
Students could all write down the numbers $0$ to $20$. One student could be asked to select a first triple and everyone writes that down. All students search for a second triple, whose sum is one more than the first's sum. One such triple is chosen, and everyone writes it down and starts to search for the next - until the task of finding triples whose sums are consecutive is fully understood by the group, at which point, they can work alone or in pairs to find a solution.
With the whole group, ask students to describe what problems occur and how they are dealing with them. Ask them to share any observations, or inspirations they have had. Check that the points in the key questions have been covered in the students comments.
Students who wish to continue to work experimentally could be encouraged to devise a clear recording system for the combinations they are trying. For example, starting with the 20 and 19, what are the possibilities for the other two cards. Students who want to work analytically may choose to use algebra to determine the smallest consecutive number.
Key questions
- Why have you run out of possibilities? Can you change anything to avoid that problem?
- What are the biggest and smallest sums we could get from a triple?
- Can you work out what the sevenconsecutive numbers will have to add up to?
- Can you select your triples in a logical/symmetrical way?
Possible support
It might be helpful to provide students with cards labelled $0$ to $20$ to allow them to make their arrangements. You could also provide calculators so that students can focus on the structure of the problem, rather than getting stuck with the additions.
Alternative questions include:
Possible extension
- How many different solutions do you think that there might be? Can you work out how many might be possible?
- If the numbers $0$ to $20$ were changed to different sets of $20$ numbers, would solutions still be possible? (specifically designed sets e.g. $\{10, 11, .., 30\}$ or $\{1000, 1001, ...1020\}$ or $\{0, n, 2n, 3n, 4n, ..., 20n\}$, or totally random sets)
