Triangles and petals
An equilateral triangle rotates around regular polygons and produces an outline like a flower. What are the perimeters of the different flowers?
Problem
Triangles and Petals printable sheet
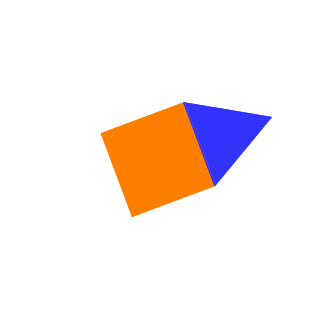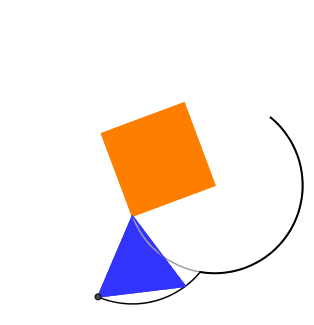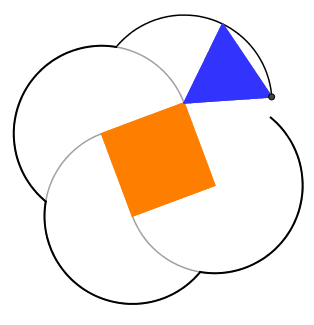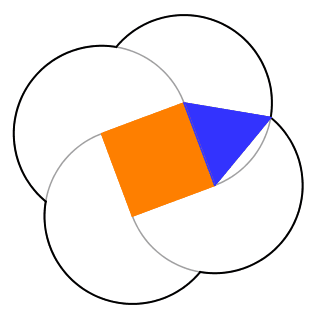
Look at the equilateral triangle rotating around the equilateral triangle. It produces a flower with three petals:
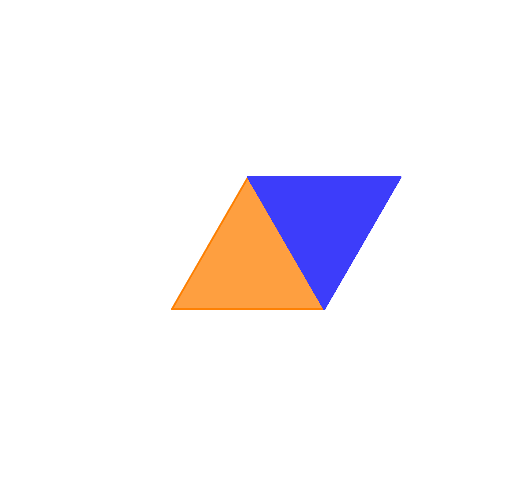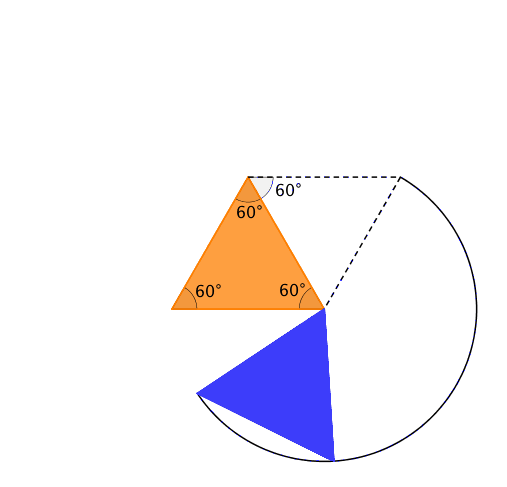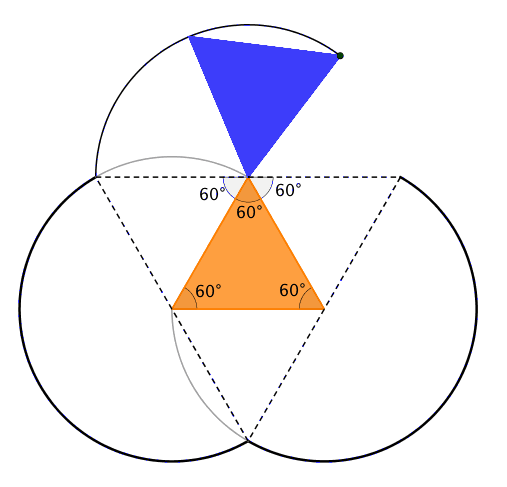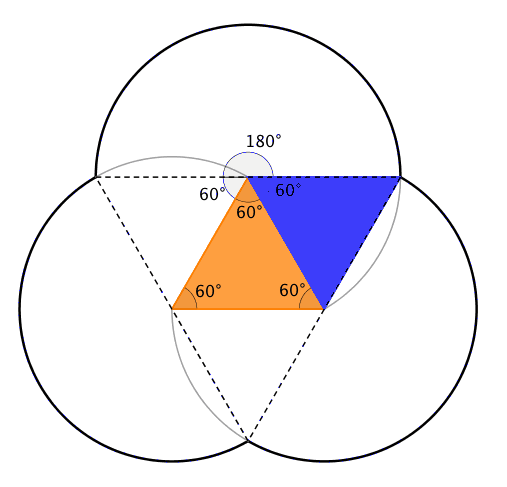
If each equilateral triangle has side length $r$, can you work out the perimeter of the flower's petals?
Now consider a flower made by the triangle rotating about a square with side length $r$ - what is the perimeter of the petals now?
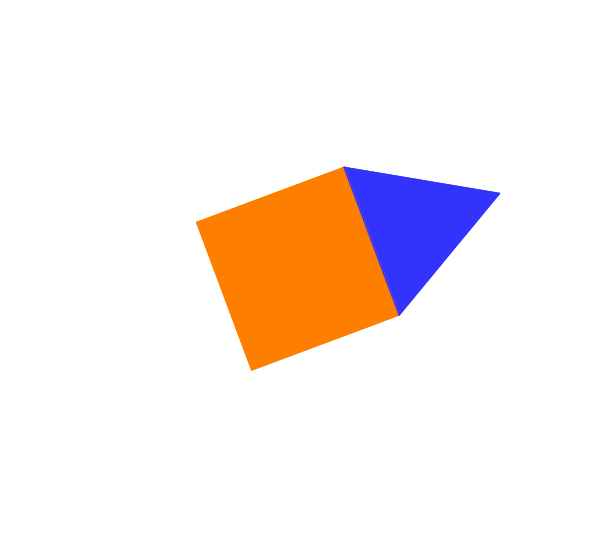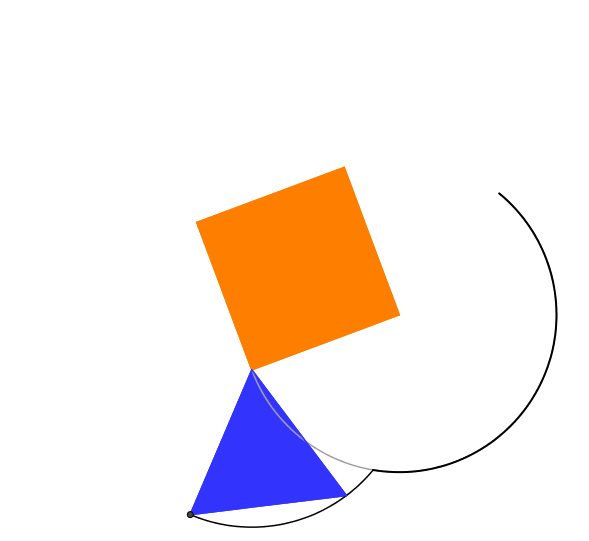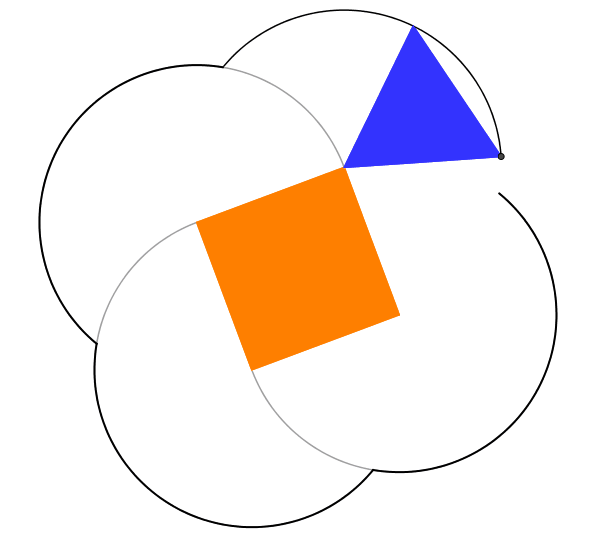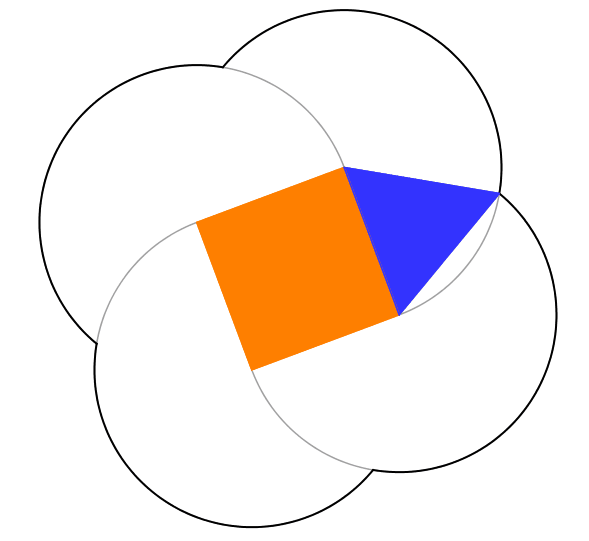
What is the perimeter when the centre of the flower is a regular pentagon, hexagon, heptagon...?
What can you say about the increase in perimeter as the number of sides of the centre shape increases?
Can you explain this increase?
What would be the perimeter of a flower whose centre is a regular $100$-sided polygon with side length $r$?
It may help to work in terms of $\pi$ throughout this problem.
Getting Started
Sketch a diagram and write on all the angles you know, and work out any angles you can find. Once you know the angle at the centre of the sectors, you can work out the proportion of the circle's circumference for each petal.


Student Solutions
Herschel from the European School of Varese sent us this solution:
The first flower has 3 petals corresponding to the 3 corners of the triangle. The completed animation shows that each petal is a semicircle, so the perimeter of the flower is $3\times \pi \times \text{ radius } = 3 \times \pi \times \text{ (side of triangle) }= 3\pi r$.
The second flower has 4 petals. This time, each petal is a sector of a circle rather than a simple semicircle. The angle of this sector is 360 - (2 triangle corners) - (1 square corner) = $360 - 2 \times 60 - 90 = 150^\circ$.
Therefore, the total perimeter of this petal is $4 \times \frac{150}{360}\times (2\times \pi \times \text{ radius }) =\frac{10}{3} \times \pi \times \text{ (side of the square) }= \frac{10}{3} \pi r$.
In general, we need to know 3 key bits of data to work out the perimeter of the flower.
They are:
- The number of sides of the central shape; we'll call this $n$.
- The length of each side in the central shape; we'll call this $r$. (Note that this is equal to the radius of the petals).
- The angle at the centre of each petal. This can be derived from $n$:
$\text{Angle } = 360 - 2 \times 60 - \text{( Corner of shape)}$
$\text{Angle } = 360 - 120 - \frac{180(n-2)}{n}$
$\text{Angle } = 240 - 180 - \frac{360}{n}$
$\text{Angle } = 60 + \frac{360}{n}$
Well done to Saif from Havering Sixth Form College, Nina, Jure and Kristjan from Elementary school Loka Crnomelj, Slovenia, Chi from Raynes Park, Rajeev from Haberdashers' Aske's Boys' School, Yun Seok Kang, and Cameron, who also sent in correct solutions. Click here to read Nina, Jure and Kristjan's thoughts.
Teachers' Resources
Why do this problem?
This problem offers the opportunity to practise calculating arc lengths, working in terms of $\pi$, and calculating interior angles of regular polygons. It also provides an opportunity to generalise from simple examples, and to explain patterns in terms of the underlying structure of the problem.
Possible approach
This printable worksheet may be useful: Triangles and Petals
Start by showing the animation of the triangle rotating around another triangle. Then hide the animation and ask learners to sketch what they saw. Show the animation once more so they can confirm what they saw.
Key questions
What angles do you know? What angles can you work out?
Possible support
An Unusual Shape offers opportunities to visualise sectors of circles and to consider simple loci.
Possible extension
Construct a proof for the formula for finding the perimeter of a flower with a regular $n$-sided polygon at its centre.
