Tangrams
Can you make five differently sized squares from the interactive tangram pieces?
Problem
| Image

| ||
Getting Started
Are any of the pieces square?
How might you use one of the red triangles to make a square?
Can you find another way?
How about using one of the green triangles?
Student Solutions
Well done to everybody who had a go at this task. As you might be able to tell from the way the question is worded, we thought there were five solutions - but there are actually some more if we allow squares that aren't completely filled!
Yiwei from The Scots College Preparatory School in Sydney, Australia sent in this picture of his solutions:
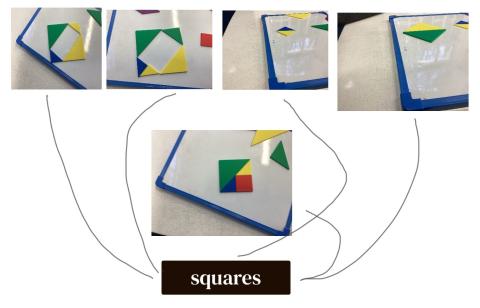
Good ideas, Yiwei. I like the way your sescond solution manages to use all five triangles!
Sam only looked at squares that were filled in, and sent these pictures of four different squares to us:




Horatio at St Johns College School, Cambridge found five ways of making filled-in squares, and also found the first solution that Yiwei sent in:

Thank you for these submitted solutions, well done!
Teachers' Resources
Why do this problem?
Not only will children be using their knowledge of properties of squares as they try this activity, but they will also be putting into practice their visualising skills. Tangrams can be great to work on in pairs and this will encourage the pupils to talk together about what they are doing - a great opportunity for you to listen!
Possible approach
You could display the interactivity on the board and start by asking children to look at the image on the left (of the tangram pieces). Invite them to consider what they see and what they might like to ask. Give them time to think on their own, then talk to a partner about their 'noticings'. Encourage some pairs to share their observations and questions with the whole group. Try to take a step back and allow the class to respond to the comments and questions themselves, rather than you validating all contributions. Some children might talk about the different shapes they can see (and colours), some might describe the outline shape of the square. Build on their thoughts to introduce the idea of a tangram.
You can then set the class off in pairs on the challenges. After some time, invite them to offer 'hints' for the rest of the group, without giving away a solution. Perhaps they can offer advice about what they are finding helpful to focus on as they tackle the challenges, perhaps they can suggest a starting point for one of the squares... These challenges are not straightforward and learners are likely to get stuck. Talk about this with the class. How does it feel to be stuck? How are they trying to get unstuck? How does it feel when they make a breakthrough? You can stop the class as appropriate to share experiences.
The completed squares could make a lovely classroom display, accompanied by some brief notes written by the class, focusing on how they went about finding solutions.
Key questions
How many pieces have you got altogether?
What could you put with this piece to make a square?
Are all the pieces different?
What's the smallest square you can make?
Possible extension
To find more tangrams on the site (many of which have interactivities), enter 'tangram' in the top right-hand search box. Our interactive Tangram Browser contains a whole collection of tangrams.
Possible support
You may have to encourage some children to experiment and 'have a go'.
