Hexy-metry
Problem
Hexy-metry printable sheets: main problem , idea cards
Image
|
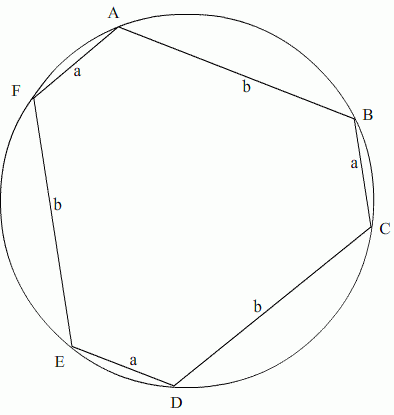
A hexagon is inscribed inside a circle.
The sides of the hexagon are alternately $a$ and $b$ units in length.
What is the radius of the circle?
|
Getting Started
Make cards from the images in this document .
Do they help you to make connections?
Can you arrange them in ways that help you to see some of the properties of the problem image?
Student Solutions
This very good solution came from The Maths Club at Wilson's School :
The interior angles of the hexagon are all equal to 120 degrees by symmetry.
Let O be the centre of the circle, with radius $r$. Considering triangle BCD, by the cosine rule,
Considering triangle BOD, by the cosine rule,
As $ \cos 120 = -\frac {1}{2}$, we have $a^2 + b^2 + ab = 3r^2$ so
Image
|
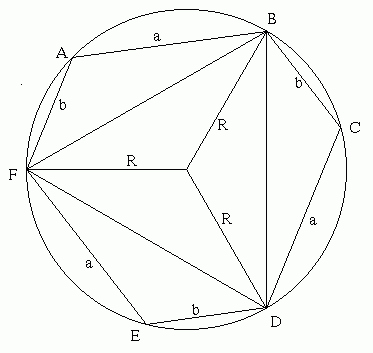
Ruth from the Manchester High School
for Girls proves the figure has rotational symmetry of order 3 and
the angles are 120 degrees as follows, and then goes on to find the
solution using the Cosine Rule as above:
Let O be the centre of the circle and $R$ be the radius.
Construct the radii OA, OB, OC, OD, OE and OF. This creates 6
isoceles triangles, 3 with sides $R, R$ and $a$ and 3 with sides
$R, R$ and $b$, therefore by SSS congruence, angles
FAO=AFO=CBO=BCO=DEO=EDO and BAO=ABO=CDO=DCO=FEO=EFO therefore the
angles of the hexagon are all 120 degrees. ACE and FBD are therfore
equilateral triangles.
|
|
Matt, who did not reveal his school,
used a different method not involving the Cosine Rule.
This hexagon is formed by drawing an equilateral triangle over
a circle where the centre of the circle coincides with the centre
of the triangle and joining the points at which the triangle
crosses the circle. The shorter edge of the hexagon has length $FB=
a$ and the longer has length $b$. Therefore line AB has length
$\frac {a}{2}$ and line BD has length $b$.
Constructing the equilateral triangle BCD defines line BC as
having length $b$. So line AC has length $\frac{a}{2}+b$.
|
Image

|
Teachers' Resources
Why do this problem?
This problem requires the solver to reason geometrically and make use of symmetry. By re-presenting the information in a different way, for example by adding additional lines (a useful technique in geometrical problems) more structure can be revealed. It is an interesting idea that adding something, and therefore apparently making it more complex, can sometimes make a problem more accessible. Then of course there is an opportunity to use the cosine rule in a non-standard context.
Possible approach
Use the images in this document to make cards.
Display the problem and ask learners to work in groups rearranging the cards in ways which help to make connections for them. Members of the group should explain their ideas to their group, and the group should agree on cards that go together, or complement each other.
Share ideas and relationships that groups notice before going on to solve the problem. Encourage careful reasoning and convincing arguments. For example:
- "Why can you say those two angles are equal?
There are several ways of solving the problem so share different approaches and discuss what helped to move thinking forward.
Two observations may be worth drawing attention to :
- the reflection symmetry between $a$ followed by $b$ and $b$ followed by $a$,
- the angle at the circumference as half the angle subtended at the centre onto the same arc (in this case an arc greater than a semicircle) .
Key questions
- How can you use the symmetry of the figure to determine some of the angles ?
- What things in the images helped to make connections for you?
- What do we need to find, and can that be found directly ?
Possible support
Ask the students if they can explain why these should all be equal .
Possible extension
