Colourful cube
A colourful cube is made from little red and yellow cubes. But can you work out how many of each?
Problem
Colourful Cube printable sheet
This cube is made up from $3\times 3\times 3$ little cubes whose faces are either all red or all yellow.
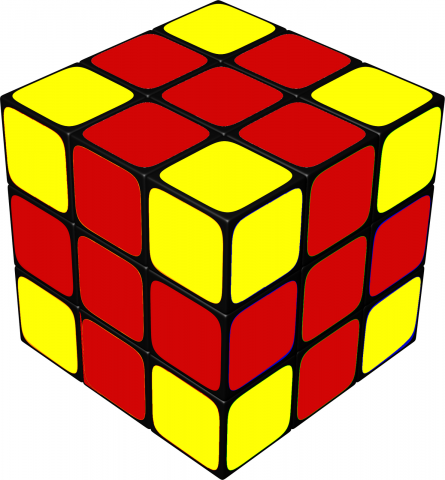
The views from all sides of the cube look like this, and the little cube in the centre is red.
How many little red cubes are used in total? How many little yellow cubes are used?
Suppose the other views of the cube do not necessarily look like this, and the little cube in the centre is not necessarily red.
What is the most and least number of little red cubes that could be used?
With thanks to Don Steward, whose ideas formed the basis of this problem.
Student Solutions
If all the sides look the same and the centre cube is red, then the corner cubes are the only yellow ones. Since a cube has $8$ corners, there are $8$ yellow little cubes. Also, the drawn cube has $3^3=27$ little cubes and any of them is either yellow or red, so there are $27-8=19$ red little cubes.
If we know only the colour of the little cubes we see, then, by counting them, we know that there are $7$ yellow cubes and $12$ red ones. Therefore, there are $27-(7+12)=8$ cubes of unknown colour. The maximum number of red cubes would therefore be achieved if all the unknown colour cubes will be red, so the maximum number of red cubes is $20$, while the minimum number is achieved if all the unknown colour cubes are yellow and the number of red ones is $12$.
Teachers' Resources
This printable worksheet may be useful: Colourful Cube.
This problem has not yet got Teachers' Notes.
If you have used this problem in your classroom, and would like to share your approach and your experiences with other teachers, please email us at secondary.nrich@maths.org
