Three Way Mix Up
Jack has nine tiles. He put them together to make a square so that two tiles of the same colour were not beside each other. Can you find another way to do it?
Age
5 to 11
Challenge level
Problem
Jack has three blue tiles, three yellow tiles and three red tiles.
He put them together like this to make a square.
Image
He made the rule that you could not put two tiles of the same colour beside each other.
Can you find another way to do it?
Can you find ALL the ways to do it?
You could try out some ideas using this interactive:
Printable NRICH Roadshow resource.
Click here for a poster of this problem.
Getting Started
It might help to have some coloured counters to move around, or you could use some squared paper and three coloured pencils/pens.
Have you checked to see if your arrangements obey the rule?
How do you know your arrangement is different from the others you've made?
Student Solutions
We received several different arrangements of tiles - thank you to those who sent in your suggestions. You had to remember that you didn't necessarily need one tile of each colour in every row and column - it was just that tiles of the same colour weren't allowed to touch. Not many of you looked for more than one other arrangement but Kesavan from Latymer All Saints C of E Primary sent in these solutions:
Image
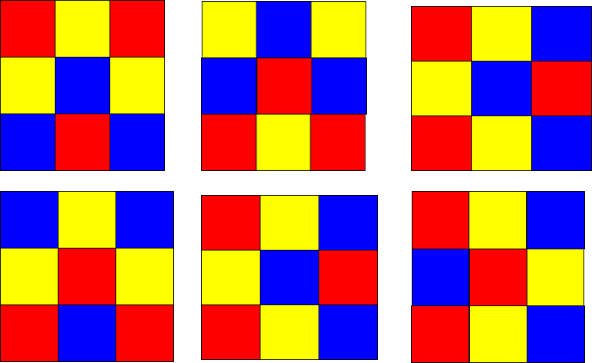
Louise from Farnborough Hill sent these arrangements:
Image
Trish and James sent in their solutions:
Image
We put all the blues on the diagonal, so none of them were touching, and then we put a red in one corner and a yellow in the other so they weren't touching and then we had to put the other colours where they are. Then we did the same with a red diagonal and a yellow diagonal:
Image
Image
A great way of working out some possible results!
Finding all the possible arrangements of tiles is quite a challenge and some of you began to have a go at this. Danny told us how he worked out how many possible combinations there were:
First I worked it out starting with blue in the top left corner and red next to it in the top middle.There are six different ways of starting (blue then red, blue then yellow, red then blue, red then yellow, yellow then red, yellow then blue) so there will be six times as many answers as I got starting with blue then red. This is because if I take one answer and swap all the blues for yellows and yellows for blues I get another answer, and there are six different ways to swap the colours.
The two squares below the starting two can be yellow then blue, red then blue or red then yellow. That way I had three different ways the top left two by two squares could be coloured.
Then I worked out how many different ways you can colour in the top right and middle right squares.
There are three ways for each of the different starting two by two squares.
For instance for the starting two by two squares with yellow then blue on the second row, here are the first and second rows of the whole arrangement:
Image
Then I worked out how many ways the bottom squares can be coloured and I think there are a total of three ways which work with this arrangement of the top left two by two square.
Then I looked at the arrangements of still having blue and red in the top left and top middle, but this time with red and blue underneath them, but none of these worked.
Finally, I looked at the arrangements of blue and red in the top left and top middle with red and yellow underneath them and I found another three ways.
So, I think there are six different ways of starting with blue then red, so there must be thirty six different ways altogether.
Thank you, Danny!
However, Suzanne, working with her son Lawrence at home, wrote to us to say:
We have just been doing 'Three Way Mix Up' and our solution is a little at odds with the solution published. Danny suggests that there are 6 ways of starting namely blue, red; blue, yellow; red, blue; red, yellow; yellow, blue and yellow red. So far so good. However he then works out that there are 6 possible permutations for each starting pair. Not so - there are only 4. If you look at the illustration of his solution, the middle one i.e. blue, red, blue then yellow, blue, red, has no possible third row without 2 yellows being next to each other. Therefore there are only 4 possible permutations for each of his starting duos.
Thank you for pointing this out, Lawrence and Suzanne!
We used a different approach. Using coloured milk carton lids and moving them round, we looked at the different patterns possible and discovered that there were two basic patterns - chevrons and diagonals. We then worked out all the possible colour combinations for each pattern. This does indeed give you 36 possible combinations but we worked them all out to be sure.
Here is a picture of some of their possible combinations:
Image
They sent the following explanation about the pictures:
Diagonals yellow, red then blue going both ways with both different permutations of the other two colours (3 x 4).
Then the chevrons red/yellow and yellow/red going L to R, R to L, top to bottom and bottom to top (2 x 4 = 8).
Then for the other two chevron colour combinations blue/red and red/blue then blue/yellow and yellow/blue we have only included the basic pattern (L to R), each of which is multiplied by 4 for the four different directions.
So 3 x 8 chevron combinations.
Hope this makes sense!
I like the chevron and diagonal idea - thank you to you both for sending in your thoughts.
Teachers' Resources
Three Way Mix Up
Jack has three blue tiles, three yellow tiles and three red tiles.
He put them together like this to make a square.
Image
He made the rule that you could not put two tiles of the same colour beside each other.
Can you find another way to do it?
Can you find ALL the ways to do it?
Why do this problem?
This activity allows pupils to explore in a non-threatening 'game-type' way, and begin to talk about what they are doing.
Possible approach
Start off with some tiles in a line and ask the children to talk in pairs about what they see. Invite them to share their observations - this may result in them counting the ones that are the same colour and talking about those numbers. Encourage them to use vocabulary such as 'more than', 'less than', 'same as', 'to the right of', 'to the left of', 'next to' etc.
Then move on to the three by three arrangement and ask them what they notice now. As they share their thoughts, you may need to introduce new vocabulary such as 'rows' and 'columns'. Set up the challenge as stated and ask learners to work in pairs. It would be good to have a space on the wall or the board for a pair to add a new solution once they have found one.
Bring the children together to look at all the solutions they have found so far. At this point, some may notice that in fact they are not all different and there may be some discussion needed as to what 'same' and 'different' mean in this context. Do rotations count as the same?
You may like to keep this as a 'simmering' activity over a number of weeks, then come back to it. How do the children know that they have found all the different arrangements? Did anyone have a good way of finding them all?
Key questions
What coloured tiles have you got here?
Have you checked it to see if it obeys the rule?
How do you know your arrangement is different from the others you've made?
Possible extension
Look at using different rectangles rather than a square arrangement.
Possible support
For youngsters who have difficulty discerning colours, this alternative may be useful:Image
(The file for this alternative black and white version can be found here.)
Help may be necessary for some by way of an adult to assist the pupil with focusing on both the columns and the rows. Having counters to move around or a three by three grid to colour will also help.