Gaudi's design
Eight lines are drawn in a regular octagon to form a pattern. What fraction of the octagon is shaded?
Age
11 to 16
Challenge level
Problem
Image
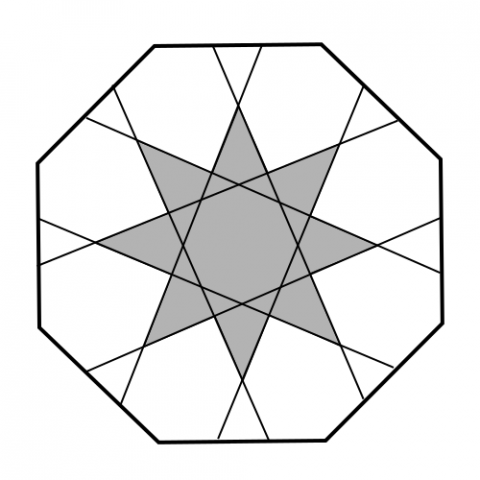
The diagram shows a ceramic design by the Catalan architect Antoni Gaudi. It is formed by drawing eight lines connecting points which divide the edges of the outer regular octagon into three equal parts, as shown.
What fraction of the octagon is shaded?
Student Solutions
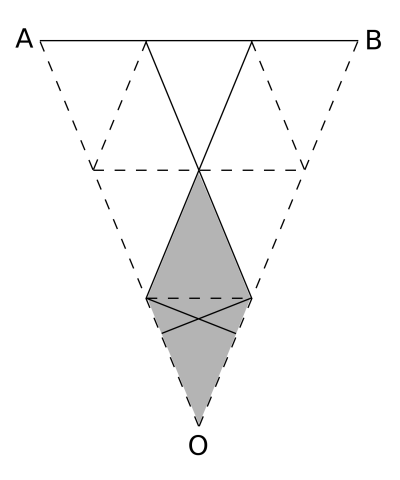
The diagram shows part of the ceramic. $A$ and $B$ are vertices of the outer octagon, which has $O$ at its centre. The lines $OA$, $OB$, two lines which are parallel to $AB$ and lines parallel to $OA$ and $OB$ respectively have been added. As can be seen, these lines divide $\triangle OAB$ into nine congruent triangles.
The shaded portion of triangle has area equal to that of two of the smaller triangles. So $\frac{2}{9}$ of the area of $\triangle OAB$ has been shaded. Now, the area of the outer octagon is eight times the area of $\triangle OAB$ and the area of shaded portion of the design is eight times the area of the shaded portion of $\triangle OAB$. Therefore, the fraction of the octagon which is shaded is also $\frac{2}{9}$.
Image
