Family tree
Problem
You have to use the clues given in the question to find out who's who in the family, to fill in the family tree and to find out which of the family members are mathematicians and which are not. This question does not require any mathematical knowledge but it does call for logical thinking which is needed to do mathematics.
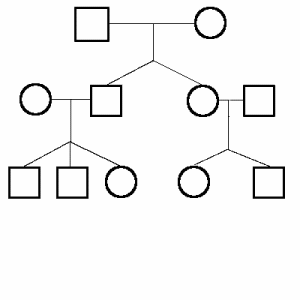
In the family tree square boxes indicate males and round boxes females.
Each set of siblings (brothers and sisters) is in descending order of age from left to right across the page.
Exactly five of the people represented in the tree are mathematicians.
Unusually for this sort of problem, everyone always tells the truth.
Last Christmas they were assembled together (and only people on this family tree were there). They made the following statements and, of course, none of them would be so rude as to mention anyone who was not present.
It may help you to know that E is the youngest male in the family, and that he and K are both mathematicians.
Image
|
A. My father is a mathematician. B. So is mine. C. And mine. D. And mine. E. Mine too. F. Neither of my parents is a mathematician but my one and only brother is. G. Only one of us females is a mathematician but it's not me. H. I've given birth to two mathematicians, no more and no less. I. I am the oldest mathematician in the family. J. My brother-in-law is a mathematician. C. My husband isn't. D. Exactly one of my cousins is. G. B is my granddaughter. |
Printable NRICH Roadshow resource.
Getting Started
It would be useful to talk to a friend as well!
Student Solutions
We had several solutions to this problem. Michael explained his reasoning as below. You will probably find it easiest to follow the solution if you fill in the people on a copy of the tree as you read it.
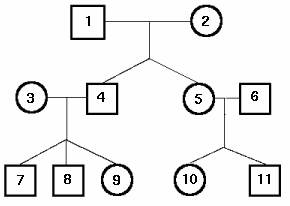
G must be $1$ or $2$ as she mentions a granddaughter; and she is
female so she is number $2$. We also know that she is a
non-mathematician. Her granddaughter, B, must be $9$ or $10$.
E, as the youngest male, must be $8$ or $11$.
J is $6$ or $3$ (if we allow 'brother-in-law' to describe her
husband's sister's husband).
F has exactly one brother, so must be $5$, $7$, $8$ or $10$.
C has a husband, and says her father is a mathematician, so C is
$5$. Therefore $1$ is a mathematician, and as the oldest
mathematician in the family can be identified as I. F can no longer
be $5$.
D, having cousins in the tree, must be one of the third
generation.
There is now no option but to assume that J is $6$. [Michael
hasn't quite explained why: he probably noticed that $6$ is C's
husband, and therefore not a mathematician, and so J can't be
$3$.] From here we can deduce that $4$ is a mathematician, and
that E and B are his children, since they have each said that their
father is a mathematician.
We can now rule out F being $7$ or $8$, and so F is $10$. F tells
us that her parents (C and J) are not mathematicians, and her
brother ($11$) is.
H is a mother of two mathematicians, so she is $3$, and either $7$
or $9$ is a mathematician (we were told that E is). This means that
for D to have just one mathematician cousin, D must be $7$ and F
must be a non-mathematician.
Finally, A must be $11$, as his father is a mathematician, so K is
$11$. We still haven't said which of $7$ and $9$ is the
mathematician, but since we haven't yet got a female mathematician,
it must be B.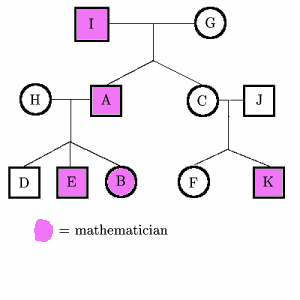
Other people who sent in correct solutions (but mostly without such detailed explanation) were Claire , Sian and Fiona of Stamford High School; Ankaru; Samantha, Jacqui, Sandy and Claire of The Mount School, York; Chong Ching, Clement Goh, Chen Wei and Ng Yan; Emma and Monica of Hethersett High School, Norwich.
