Equation matcher
Can you match these equations to these graphs?
Problem
Fitting algebraic curves through experimental data points is an important scientific process which allows us to make predictions of the behaviour of a system away from the observed points. But which equations are sensible choices for a particular set of data? This question draws us into the general process of curve fitting.
In four different sorts of experiments, two values of $x$ and $y$ are successively measured and plotted on charts below:
Image
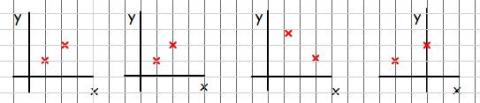
For each chart, which of the following equations are possible descriptions of the underlying process?
What restrictions would the data place on the ranges of the numbers $a$ and $b$ in each case? For example, do the data points imply that the constants will be positive?
Another point is subsequently measured and plotted on the graphs in each case.
Image
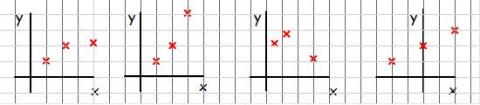
How do the possibilities now change?
Can you give examples of physical systems which are modelled by equations of these types?
Extension: Which curves can be distinguished by three points? i. e., could you pick three points which would rule out all but one of the possibilities in each case?
Teachers' Resources
Why do this problem?
This
problem encourages students to get into the real meaning of
graphical representation without getting bogged down in algebraic
calculations or falling back into blind computation. It will also
encourage them to think about error in measurement.
Possible approach
There are two levels at which the graphical data can be
interpreted. At a basic level, the students can easily see if a
measurement increases or decreases from point to point or whether
the measurement is positive or negative. At a more advanced level,
they can suggest some rate of change of the measurements from point
to point: although there are no units on the charts, there are
certain key points (the grid lines) which allow some 'indirect'
by-eye measurement. Students will need to realise this more subtle
point to make full progress. (Note that the points have been
carefully placed by the question setter!)
The question of the accuracy of the 'by-eye' measurements can
raise interesting discussion about the accuracy of the
measurements. Since no context is given in the question it is
natural to assume total accuracy, but would this be the case in
practice?
Key questions
- We only have two measurements, but what information can we deduce from these?
- How can we relate this information to the equations?
Possible extension
Think of other equations which might match the points on the
graphs. How many could you think of?
Possible support
Let students leaf through a science textbook searching for
graphs and charts. Do they notice that the same shapes of charts
appear frequently? For each chart they find, which measurements
would be a possibility?
You might also first try Real-life
equations.
