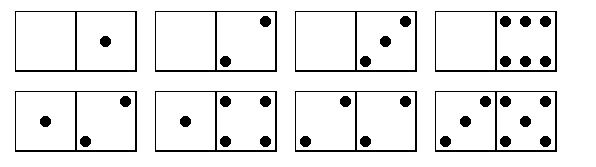
Congratulations to Katherine, from Maidstone
Girls' Grammar School who reasoned that there are the 24 unique
domino solutions given below, where each arrangement has the 5-3
domino horizontally. Katherine gave the answer 384. She explained
that there are 3 columns of dominoes which can be arranged in six
different ways. Each of these arrangements can be varied by
swopping the rows giving 6 x 4 = 24 arrangements. Katherine then
explained that from each of these 24 arrangements you can find 8
others: 4 which are mirror images and 4 which are rotations. This
gives 192 (24x8) patterns altogether. Then if you take the 2-2 you
can also turn it round 180 degrees to form twice as many solutions.
This gives 384 (192x2) domino square solutions.
James of Hethersett High School
explained that ``I have found that the domino 5-3 is the key
domino, as wherever it goes it has to be followed by two blanks.''
He explained that, from one solution, he
found different patterns by swapping the rows or columns. Daniel
and Michael of Necton Middle School, Norfolk found one of the
solutions. Camilla of Maidstone Girls' Grammar School discovered
that in her solutions certain blocks stayed next to one another and
concluded that having found one solution all the others are
different ways of re-arranging it.
| 5-3 |
B |
B |
| 1-2 |
3 |
2 |
| B-1 |
1 |
6 |
| 2-2 |
4 |
B |
|
| 5-3 |
B |
B |
| 1-2 |
2 |
3 |
| B-1 |
6 |
1 |
| 2-2 |
B |
4 |
|
| B |
5-3 |
B |
| 3 |
1-2 |
2 |
| 1 |
B-1 |
6 |
| 4 |
2-2 |
B |
|
| B |
5-3 |
B |
| 2 |
1-2 |
3 |
| 6 |
B-1 |
1 |
| B |
2-2 |
4 |
|
| B |
B |
5-3 |
| 3 |
2 |
1-2 |
| 1 |
6 |
B-1 |
| 4 |
B |
2-2 |
|
| B |
B |
5-3 |
| 2 |
3 |
1-2 |
| 6 |
1 |
B-1 |
| B |
4 |
2-2 |
|
| 5-3 |
B |
B |
| 1-2 |
3 |
2 |
| 2-2 |
4 |
B |
| B-1 |
1 |
6 |
|
| 5-3 |
B |
B |
| 1-2 |
2 |
3 |
| 2-2 |
B |
4 |
| B-1 |
6 |
1 |
|
| B |
5-3 |
B |
| 3 |
1-2 |
2 |
| 4 |
2-2 |
B |
| 1 |
B-1 |
6 |
|
| B |
5-3 |
B |
| 2 |
1-2 |
3 |
| B |
2-2 |
4 |
| 6 |
B-1 |
1 |
|
| B |
B |
5-3 |
| 3 |
2 |
1-2 |
| 4 |
B |
2-2 |
| 1 |
6 |
B-1 |
|
| B |
B |
5-3 |
| 2 |
3 |
1-2 |
| B |
4 |
2-2 |
| 6 |
1 |
B-1 |
|
| 1-2 |
3 |
2 |
| 5-3 |
B |
B |
| B-1 |
1 |
6 |
| 2-2 |
4 |
B |
|
| 1-2 |
2 |
3 |
| 5-3 |
B |
B |
| B-1 |
6 |
1 |
| 2-2 |
B |
4 |
|
| 3 |
1-2 |
2 |
| B |
5-3 |
B |
| 1 |
B-1 |
6 |
| 4 |
2-2 |
B |
|
| 2 |
1-2 |
3 |
| B |
5-3 |
B |
| 6 |
B-1 |
1 |
| B |
2-2 |
4 |
|
| 3 |
2 |
1-2 |
| B |
B |
5-3 |
| 1 |
6 |
B-1 |
| 4 |
B |
2-2 |
|
| 2 |
3 |
1-2 |
| B |
B |
5-3 |
| 6 |
1 |
B-1 |
| B |
4 |
2-2 |
|
| 1-2 |
3 |
2 |
| 5-3 |
B |
B |
| 2-2 |
4 |
B |
| B-1 |
1 |
6 |
|
| 1-2 |
2 |
3 |
| 5-3 |
B |
B |
| 2-2 |
B |
4 |
| B-1 |
6 |
1 |
|
| 3 |
1-2 |
2 |
| B |
5-3 |
B |
| 4 |
2-2 |
B |
| 1 |
B-1 |
6 |
|
| 2 |
1-2 |
3 |
| B |
5-3 |
B |
| B |
2-2 |
4 |
| 6 |
B-1 |
1 |
|
| 3 |
2 |
1-2 |
| B |
B |
5-3 |
| 4 |
B |
2-2 |
| 1 |
6 |
B-1 |
|
| 2 |
3 |
1-2 |
| B |
B |
5-3 |
| B |
4 |
2-2 |
| 6 |
1 |
B-1 |
|
Reflections in the diagonals give patterns with the 5-3 domino
placed vertically and reflections in the vertical mirror line give
patterns with this domino placed as 3-5. Reflections in the
horizontal mirror line turn the vertically placed dominoes upside
down.
Carrie who goes to Saint Thomas More School
has found another solution, based on this pattern of dominoes:
The numbers are:
| 1 |
2 |
3 |
2 |
| B |
2 |
5 |
1 |
| 1 |
4 |
B |
3 |
| 6 |
B |
B |
2 |
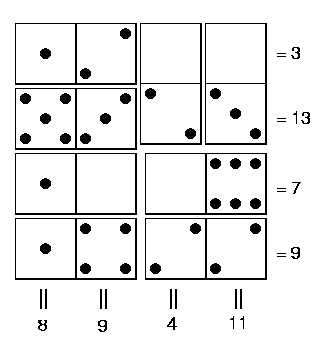
No-one else has found a different solution
that is not derived from the first basic pattern. You may like to
investigate patterns such as the one below, or investigate Carrie's
pattern further.