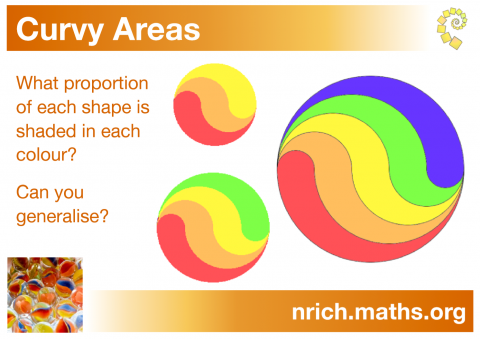
Curvy areas poster

3 pieces: each piece is $\frac13$ of the total area

4 pieces: each piece is $\frac14$ of the total area

5 pieces: each piece is $\frac15$ of the total area

$m$ pieces: each piece is $\frac1m$ of the total area

Why?

Red, orange and yellow

The top half is made of 3 semicircles on top of each other:

Image

Say the smallest semicircle has radius 1, the medium semicircle has radius 2 and the largest has radius 3

Smallest semicircle has area $\frac12\pi\times1^2 = \frac{\pi}2$

Medium semicircle has area $\frac12\pi\times2^2 = 2\pi$

Largest semicircle has area $\frac12\pi\times3^2 = \frac{9\pi}2$

Whole circle has area $9\pi$

Red area: smallest + (largest $-$ medium) $=\frac{\pi}2 + \frac{9\pi}2 - 2\pi = 3\pi$ which is $\frac13$ of $9\pi$

Yellow area is equal to red area so the orange area must also be $\frac13$ of the total.

Red, orange, yellow and green

Include also extra large semicircle (XL), area $\frac12\pi\times4^2 = 8\pi$

Whole circle has area $16\pi$

Red area: smallest + (XL $-$ large) $= \frac{\pi}2 + 8\pi - \frac{9\pi}2 = 4\pi$ which is $\frac14$ of $16\pi$

Orange area: (medium $-$ smallest) + (large $-$ medium) $=$ large $-$ smallest $= \frac{9\pi}2 - \frac{\pi}2 = 4\pi$

So each piece is $\frac14$ of the total area.

Red, orange, yellow, green and blue

XXL semicircle area $\frac12\pi\times5^2 = \frac{25\pi}2$

Whole circle has area $25\pi$

Red area: smallest + (XXL $-$ XL) $=\frac{\pi}2 + \frac{25\pi}2 - 8\pi = 5\pi$ which is $\frac15$ of the total area

Orange area: (medium $-$ smallest) + (XL - large) $= 2\pi - \frac{\pi}2 + 8\pi - \frac{9\pi}2 = 5\pi,$ also $\frac15$ of the total area

Yellow area: (large $-$ medium)$\times2 = \left(\frac{9\pi}2 - 2\pi\right)\times2 = 5\pi$

So each piece is $\frac15$ of the total area.

$m$ pieces

Largest piece has area $\frac{m^2\pi}2$ and whole circle has area $m^2\pi$

'Red' piece: 

$\begin{align}\frac{\pi}2 + \frac{m^2\pi}2 -  \frac{(m-1)^2\pi}2 &= \tfrac{\pi}2\left(1+m^2 -(m^1-2m+1\right) \\ &= m\pi\end{align}$

'Orange' piece:

$\begin{align} \left(2\pi - \frac{\pi}2\right) + \left(\frac{(m-1)^2\pi}2 - \frac{(m-2)^2\pi}2\right) &= \frac{\pi}2\left(4-1+\left(m^2-2m+1\right)-\left(m^2-4m+4\right)\right)\\ &= \tfrac{\pi}2\left(4m-2m\right)\\&=m\pi\end{align}$

General piece:

$\left(\frac{(r+1)^2\pi}2 - \frac{r^2\pi}2\right) + \left(\frac{(m-r)^2\pi}2 - \frac{(m-(r+1))^2\pi}2\right) \\

\begin{align} &= \tfrac{\pi}2\left((r+1)^2-r^2+\left(m^2-2mr+r^2\right)-\left(m^2-2m(r+1)+(r+1)^2\right)\right)\\ 

&= \tfrac{\pi}2\left(2m(r+1)-2mr\right)\\&=m\pi\end{align}$

$\therefore$ all of the pieces have the same area, $\frac1m$ of the total.