Cuboid faces
Can you find the volume of a cuboid, given the areas of its faces?
Problem
The faces of a cuboid have areas of 12, 18 and 24 square centimetres.
What is the volume of the cuboid?
This problem is adapted from the World Mathematics Championships
Student Solutions
Working out the lengths of the edges
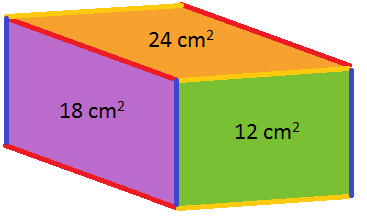
From this diagram, we can see that:
If the yellow edge is 6 cm long and the blue edge is 2 cm long, then the red edge must be 9 cm long, because 2$\times$9 = 18. However, then the orange face would have area 6$\times$9 = 54 square centimetres, which is not right.
If the yellow edge is 2 cm long and the blue edge is 6 cm long, then the red edge must be 3 cm long, because 6$\times$3 = 18. However, then the orange face would have area 2$\times$3 = 6 square centimetres, which is not right.
If the yellow edge is 4 cm long and the blue edge is 3 cm long, then the red edge must be 6 cm long, because 3$\times$6 = 18. Then the orange face would have area 4$\times$6 = 24 square centimetres, which is right.
So the edges of the cuboid are 3 cm, 4 cm and 6 cm long, which means the volume of the cuboid is 3$\times$4$\times$6 = 72 cm$^3$.
Using algebra to find the lengths of the edges
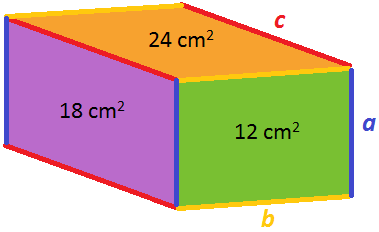
If we call the lengths of the edges $a$, $b$ and $c$, then $ab=12$, $ac=18$ and $bc=24$.
From here, we can use a trial approach to work out $a$, $b$ and $c$ similar to the method described above, or we can solve these equations, as shown below.
Since $ab=12$, we can say that $a=\dfrac{12}{b}$.
Substituting into $ac=18$, we get $\dfrac{12}{b}\times c=18$. Rearranging, $12c=18b$, so $c=\dfrac{18b}{12}=\dfrac{3b}{2}$.
Substituting into $bc=24$ now gives $b\times\dfrac{3b}{2}=24$, so $\dfrac{3b^{2}}{2}=24$, so $b^{2}=16$, so $b=4$.
So $a=\dfrac{12}{4}=3$ and $c=\dfrac{3\times4}{2}=6$.
So the volume, given by $abc$, is equal to $3\times4\times6=72$ cm$^3$.
Using algebra to find the volume directly
As in the picture above, we can call the lengths of the edges $a$, $b$ and $c$. So $ab=12$, $ac=18$ and $bc=24$.
We want the volume of the cuboid, $V=abc$.
Notice that $ab\times ac\times bc=a^2b^2c^2=(abc)^2=V^2$.
So $12\times18\times24=V^2$.
So $V=\sqrt{12\times18\times24}=72$.
So the volume is $72$ cm$^3$.
Image

From this diagram, we can see that:
- the length of the yellow edge multiplied by the length of the blue edge must be 12
- the length of the red edge multiplied by the length of the blue edge must be 18
- the length of the yellow edge multiplied by the length of the red edge must be 24.
If the yellow edge is 6 cm long and the blue edge is 2 cm long, then the red edge must be 9 cm long, because 2$\times$9 = 18. However, then the orange face would have area 6$\times$9 = 54 square centimetres, which is not right.
If the yellow edge is 2 cm long and the blue edge is 6 cm long, then the red edge must be 3 cm long, because 6$\times$3 = 18. However, then the orange face would have area 2$\times$3 = 6 square centimetres, which is not right.
If the yellow edge is 4 cm long and the blue edge is 3 cm long, then the red edge must be 6 cm long, because 3$\times$6 = 18. Then the orange face would have area 4$\times$6 = 24 square centimetres, which is right.
So the edges of the cuboid are 3 cm, 4 cm and 6 cm long, which means the volume of the cuboid is 3$\times$4$\times$6 = 72 cm$^3$.
Using algebra to find the lengths of the edges
Image

If we call the lengths of the edges $a$, $b$ and $c$, then $ab=12$, $ac=18$ and $bc=24$.
From here, we can use a trial approach to work out $a$, $b$ and $c$ similar to the method described above, or we can solve these equations, as shown below.
Since $ab=12$, we can say that $a=\dfrac{12}{b}$.
Substituting into $ac=18$, we get $\dfrac{12}{b}\times c=18$. Rearranging, $12c=18b$, so $c=\dfrac{18b}{12}=\dfrac{3b}{2}$.
Substituting into $bc=24$ now gives $b\times\dfrac{3b}{2}=24$, so $\dfrac{3b^{2}}{2}=24$, so $b^{2}=16$, so $b=4$.
So $a=\dfrac{12}{4}=3$ and $c=\dfrac{3\times4}{2}=6$.
So the volume, given by $abc$, is equal to $3\times4\times6=72$ cm$^3$.
Using algebra to find the volume directly
As in the picture above, we can call the lengths of the edges $a$, $b$ and $c$. So $ab=12$, $ac=18$ and $bc=24$.
We want the volume of the cuboid, $V=abc$.
Notice that $ab\times ac\times bc=a^2b^2c^2=(abc)^2=V^2$.
So $12\times18\times24=V^2$.
So $V=\sqrt{12\times18\times24}=72$.
So the volume is $72$ cm$^3$.
