Caterpillars
These caterpillars have 16 parts. What different shapes do they make if each part lies in the small squares of a 4 by 4 square?
Problem

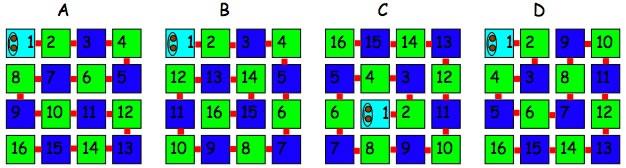
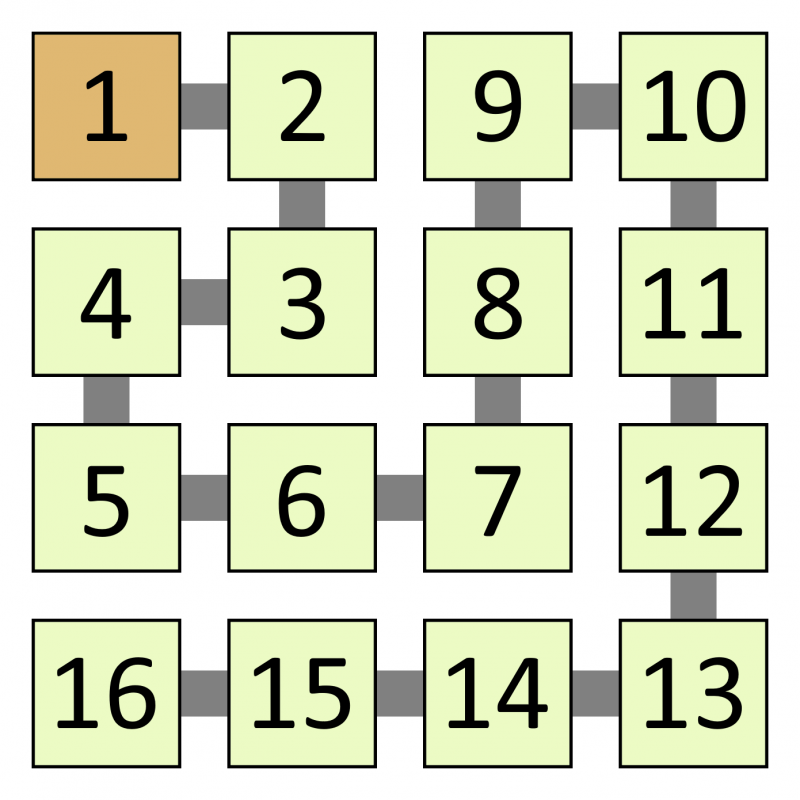
Here are some caterpillars! Our caterpillars have numbers on each little part - numbers $1, 2, 3, 4, \ldots$ up to $16$.
A Image
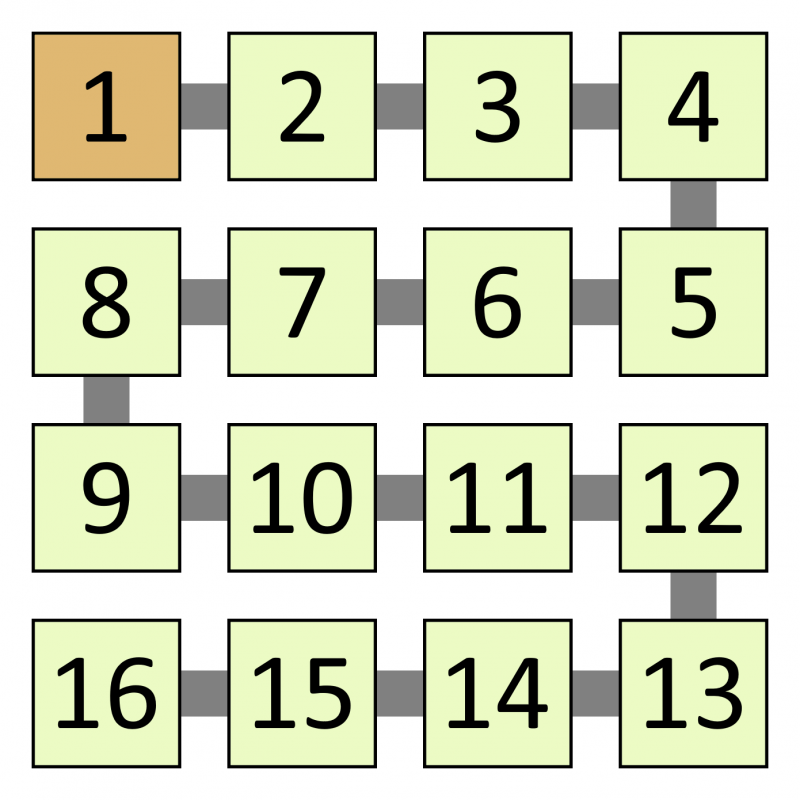
| B Image
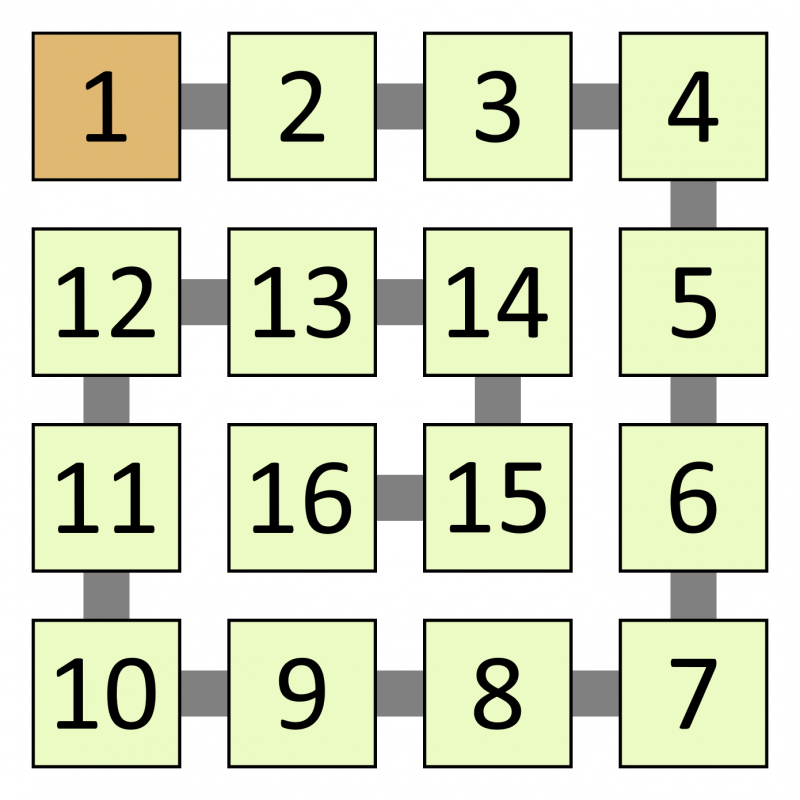
| C Image
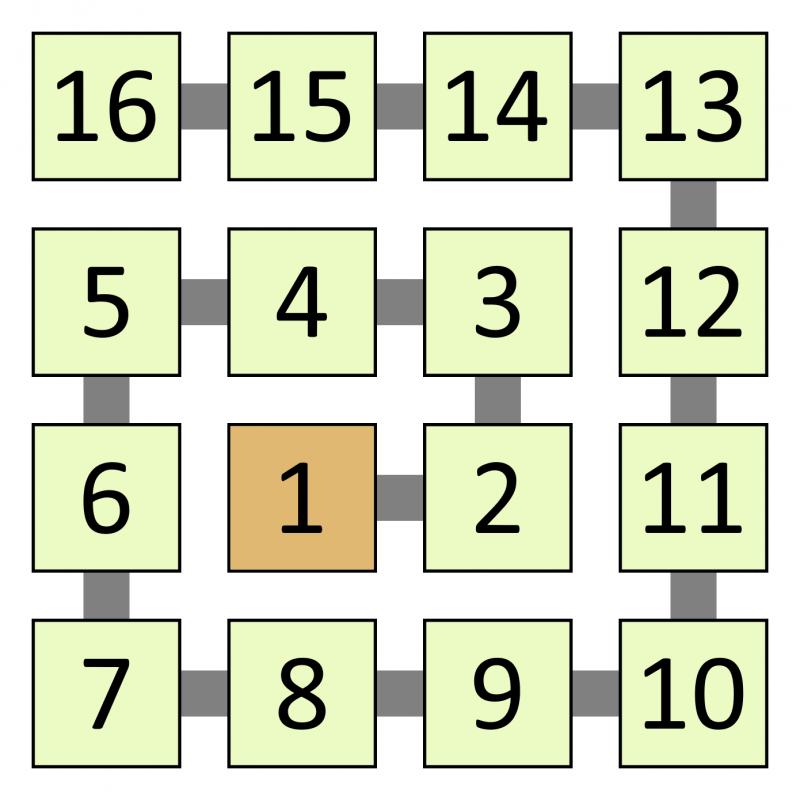
| D Image
|
You can see their orange heads, and their bodies bending at right angles so that each part is lying in a square.
There are two explorations to go with these caterpillars:
1. You could look carefully at the shapes of the caterpillars and let them turn in other ways. Here are a few to start you off:

When you've discovered many new ones, with the shapes - and therefore the numbers - all showing differently, you could compare them. What is the same and what is different? Can you explain why?
You could try to put the caterpillars in shapes that are not squares!
OR
2.
a) Choose one of the caterpillars and, using the numbers and the way that they are arranged, explore the patterns and relationships you can find.
b) Then let that caterpillar grow nine more parts so that it becomes a $25$ caterpillar with the shape bending in just the same way. Explore those patterns and relations.
c) Finally, compare the two different groups of things you've discovered in a) and in b).
Do tell us about all the things you find out.
Getting Started
Why not start with the caterpillar's head in one square and find different ways to lay out its body?
What is the same about all the different ways you have found? Why?
What differences do you notice? Can you explain them?
How will you know which ones you have found? How will you know you haven't got the same layout twice?
You could look at sums and differences in the numbers of one of your caterpillars.
Student Solutions
There are many different things to explore in this problem. Rebecca, Bethany, Ben and James from Gateway Primary School looked at finding the totals of the each row and column in their caterpillar arrangements. What a good idea!
Yajat from Hewson Public School in Canada said:
I was able to make three more patterns of caterpillar
and sent in the following picture:

Daniel wrote to say:
We did some caterpillars, and in all the different ones we did each medium square (made up of four squares inside our big square) added up to an even number, and all the rows and all the columns did too.
All the 9-square squares in our big square added up to odd numbers. We think that is because there was an odd number of squares in those squares, and because of the caterpillars being odd then even then odd then even.
Thank you for these contributions.
Teachers' Resources
Why do this problem?
Possible approach
Key questions
Possible extension
- If a caterpillar has some segments which are black then you won't be able to see their numbers, so you can offer problems with missing numbers. You can have a surprisingly large number of missing numbers and still be able to fill in every one. For example, in caterpillar A you only need to know the $1, 5, 10,$ and $11$ and you can place all the other numbers. You could challenge children to fill in the rest of the numbers, but also invite them to make their own so that they give as few numbers as possible in order for a friend to be able to complete the caterpillar. You could also extend this to larger caterpillars!
- For a tougher challenge, you could change the caterpillars so that you can't see the individual segments, only four mega-sections each of which covers four segments in a $2 \times 2$ square. Each mega-section shows you the product of the four segments of the caterpillar. For example, the top-left quarter shows $24$, top-right $7920$, bottom-right $15288$, bottom-left $7200$. Can learners identify the number of each segment? (Alan attributes this idea to Mike Taylor - thank you!) You could also set this up in a "battleships-style" game where each player records a caterpillar in a square grid and player $1$ tells player $2$ the products of $2\times2$ blocks he/she asks about, and vice versa.
Possible support