Arsenal collection: stadium sightline
Problem

Imagine that you were one of the design team when the Emirates stadium was being built. Your task is to design the tiered seating for the stadium.The back of each seat is 80cm behind the back of the seat in front, but raised up so that each row can see over the row in front.
Make some sketches showing what the cross-section (side view) of the seats might look like. Will the seats lie on a straight line, or a curve? How steep?
What factors do you think you will need to take into account when working out how high each seat needs to be?
Arsenal FC have stipulated that all spectators must be able to see clearly a point 10m in front of the front row of seating:
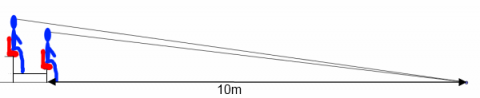
The spectator in the second row needs to have line of sight to the same point as the spectator in the first row, as seen in the diagram above. Notice that the spectator in the second row needs some extra clearance in order to see comfortably over the first spectator's head.
Here is a zoomed-in diagram:
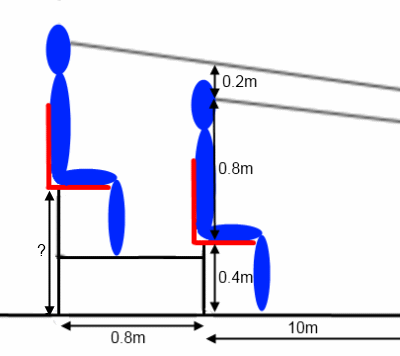
The first spectator's eye-level is 1.2m above the ground.
There is an extra 0.2m of clearance from his eye-level to the second spectator's line-of-sight. The second spectator is a further 0.8m away from the point on the pitch.
How high above ground level does spectator 2's seat need to be?
Now draw a similar diagram with the dimensions and unknowns for spectators 2 and 3.
How high above ground level does spectator 3's seat need to be?
Finally, imagine there were 40 rows of seating in the stadium.
Can you work out the heights above ground level of each of the 40 rows, and hence plot a side view of the seating?
It is very helpful to use a spreadsheet to perform the repeated calculations and plot the results.
Getting Started
What lengths do you know?
Look for similar triangles.
Teachers' Resources
Why do this problem?
This problem encourages students to model a real-life situation mathematically, that of tiered seating design in sports stadia. The problem begins with some straightforward geometry but leads into some spreadsheet modelling. Although the problem can be solved using trigonometry, the most elegant solution simply uses similar triangles.
Possible approach
"Your task is to design the seating layout for the stadium. The seats will be tiered so that the back of each seat is 80cm behind the back of the seat in front, but raised up so that each row can see over the row in front. Start by making some sketches of how you think the seats might need to be arranged."
Give students time to try out some ideas. Perhaps sketch on the board (or invite students to sketch) a variety of possibilities - straight lines of different gradients, and convex and concave curves. Then allow some time for students to share their intuitions about which will best model a successful tiered seating design, together with any reasons they have to support it.
Next, introduce the diagram:

Set the next task, to work out the missing height. Students may wish to draw or plot accurate diagrams before making calculations.
Move on to working out the height above ground level of the third seat, and allow some time to discuss whether students are surprised that the extra height is not constant.
Finally, invite students to explore what happens for subsequent steps, perhaps using spreadsheets to iterate and calculate the next step height from the previous.
Key questions
What sort of a view would spectators have if each seat was higher than the one before by the same distance?
What sort of a view would spectators have if the angle between their line of sight and the line of sight of the spectator in front was constant?
Possible extension
Invite students to consider other factors that might affect stadium seating and perhaps adapt their models accordingly.
Possible support
Track Design looks at a simpler design context at the Olympics.
