How odd

This problem challenges you to find out how many odd numbers there are between pairs of numbers. Can you find a pair of numbers that has four odds between them?

Age

5 to 7

Challenge level

Primary

Number - Multiplication, Division and Ratio

Multiplication and Division Facts

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

How Odd printable sheet

How many odd numbers are there between 3 and 11?

How many odd numbers are there between 4 and 11?

What do you notice? Can you explain your observation?

Can you find any other pairs of numbers which have this same number of odds between them?

Can you find a pair of numbers which have four odd numbers between them?

Can you find another pair of numbers which have four odds between them? And another pair?

How would you find a pair of numbers that have five odds between them? Six odds?

How would you explain to someone else how to find a pair of numbers that have a certain number of odds between them?

Student Solutions

We did not receive any satisfactory submissions this time, so here are some from past years.

Many of you were careful to say that you only counted numbers BETWEEN the two numbers given, so you didn't include the numbers themselves. That's important to get clear, I think.

Matthew and Luca from Dunchurch Boughton Junior School described what they noticed:

If the two numbers are consecutive (I think here they mean the starting numbers of each set of numbers) and the last numbers are the same, the answer will always be exactly the same.

Here are some examples:

1-9: 3, 5, 7. = three odds

2-9; 3, 5, 7. = three odds

3-15: 5, 7, 9, 11, 13. = five odds

4-15: 5, 7, 9, 11, 13. = five odds

123-131: 125, 127, 129. = three odds

124-131: 125, 127, 129. = three odds

Moana from the Canadian Academy explained what Matthew and Luca noticed by saying:

We figured out that after an odd number, it's an even number and since we don't count even numbers it doesn't change. (I think by 'it' in the second sentence Moana means the number of odd numbers.)

James from Edenlode wrote:

3 odd numbers between 3-11!

3 odd numbers between 4-11!!!

The same number of odd numbers in between!!!!!

Because the next odd number after 3 is 5!!!!!!!

Some numbers with 3 odds in between are 0 and 6 or 0 and 7!

Some numbers with 4 odds in between are 0 and 8 or 0 and 9!!!

Some numbers with 5 odds in betwen are 0 and 10 or 0 and 11!!!!!

Some numbers with 6 odds in between are 0 and 12 or 0 and 13!!!!!!!

If I was explaining to someone else how to work out how many odds between two numbers I would say 'If you start on an even number and land on an even number then the number of odds is half the number you count on. If you start on an even number and land on an odd number, then the number of odds is half the number you count on, plus one more'.

I love maths.

I wonder what happens when you start on an odd number and land on an even number? Or if you start on an odd number and land on an odd number? James also asked "Do you notice anything about my exclamation marks?".

Perhaps some of you thought about it in a slightly different way? Let us know if you did!

Teachers' Resources

How Odd

How Odd printable sheet

How many odd numbers are there between 3 and 11?

How many odd numbers are there between 4 and 11?

What do you notice? Can you explain your observation?

Can you find any other pairs of numbers which have this same number of odds between them?

Can you find a pair of numbers which have four odd numbers between them?

Can you find another pair of numbers which have four odds between them? And another pair?

How would you find a pair of numbers that have five odds between them? Six odds?

How would you explain to someone else how to find a pair of numbers that have a certain number of odds between them?

Why do this problem?

This problem will help consolidate children's understanding of, and familiarity with, odd and even numbers. It also provides an opportunity for learners to explain and generalise.

Possible approach

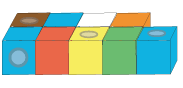
Depending on your pupils' past experience, you may want to begin with some models of some numbers made from multilink cubes. You could put the cubes together so that they are paired, for example this would be the model for 9:

Put your multilink models out for the children to see and invite them to talk about the models. You could lead on to ordering the models numerically and pupils could make their own models to fill in some of the missing numbers.

Having made, for example, models of the numbers from 1 to 12 and placed them in numerical order, you could pose a few questions to focus on odds and evens, if this has not already come up in conversation. For instance, you could ask whether there is anything the same about any of the models. Alternatively, you could group the models into two sets, one of odds and one of evens and invite the group to talk about what you've done.

You could then pose the first challenge in the problem and step back for learners to have a go in pairs. You may need to have a conversation about what 'between' means, so that, for example, the whole numbers between 3 and 11 would not include 3 or 11. Allow them to choose how they tackle it - this will be a great assessment opportunity for you. Once they have had sufficient time, talk together about their methods and conclusions. You can then go on to the other parts of the problem, again leaving it up to the children to decide how they approach it and how they record their solutions. If possible, give the group plenty of time to find lots of examples at each stage.

You could leave the final part of the problem to the plenary. Talk together about ways of finding two numbers which have, for example, ten odds between them. You may be able to encourage some children to articulate how they would find a pair with any number of odds between them.

Key questions

How will you find out how many odd numbers there are between 3 and 11, 4 and 11 etc.?

Tell me about what you're doing.

How will you remember what you've found so far?

Possible extension

You could challenge some children to find good ways of predicting the number of odd numbers between any two numbers. How about investigating the number of even numbers between a pair of numbers?

Possible support

It would be good to have multilink and number lines available along with plenty of blank paper and pencils and small whiteboards. Of course some children may want to use particular equipment which you hadn't thought of, so do allow them to choose whatever suits them best.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners