Arrow arithmetic 1
The first part of an investigation into how to represent numbers
using geometric transformations that ultimately leads us to
discover numbers not on the number line.
Problem
This problem forms the first part of an investigation into how to represent numbers using geometric transformations that will ultimately lead us to discover numbers that are not on the number line.
I'm going to assume that you are happy with the idea of numbers that lie on the number line. Just to remind you, here is a picture of a small part of the number line.
Image
The plan of action is to develop pictures or geometric representations of the numbers we know about already. We'll also develop ways to add, subtract, multiply and divide using just those pictures. Then we'll change the picture very slightly...
Here's my first attempt at a picture of the number 1. It's simply a blue arrow.
Image
I don't think this is a good enough picture yet, but it's helpful to think about its shortcomings.
Let's try to do arithmetic with these arrows. Try three simple additions.
Image
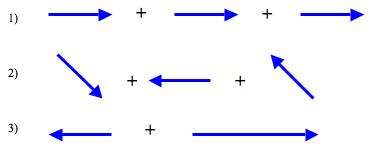
I tried these with three friends and here are the answers they gave me
| Sam | Hannah | Tim | |
| 1) | 3 | 3 | 3 |
| 2) | -1 | 3 | 3 |
| 3) | 1 | 3 | 2 |
They all had good explanations for their answers. Can you
guess what they were?
My arrow picture obviously has some shortcomings! How would
you improve it?
Getting Started
Think about what features of the arrow are representing the number
1.
Is the length important?
Is the direction important?
Sam, Hannah and Tim might have different ideas on what features mattered.
Is the length important?
Is the direction important?
Sam, Hannah and Tim might have different ideas on what features mattered.
Student Solutions
Emanuel has yet another set of solutions to these 3 problems. He ignores the length of the arrows but uses the direction! I suspect that completes the set of plausible solutions here.
We will ignore the length of an arrow in order to simplify the problem. Basing on traditional number line an arrow pointing upwards or to the right can represent a plus (+), and an arrow pointing downwards or the left can represent a minus (-).
From above the answer for question 1 is 3.
The first arrow of question 2 can represent 0 because it is halfway between +1 and 1.
The second arrow is -1 and the third also is 0 since its halfway between 1 and -1.
The result for question 2 therefore is -1
The third question is actually -1 + 1 which is 0.
Can you now interpret Sam, Tim and Hannah's solutions according to whether they pay attention to arrow length and arrow direction or not?
Teachers' Resources
We're in the early stages of developing a useful geometric model of number here.
The aim is to develop geometric analogues of number and number operations.
Ultimately, we'll want to be able to geometrically multiply the geometric analogue of 2 by the geometric analogue of 3 to yield the geometric analogue of 6.
This first idea of using a simple arrow to represent the number 1 needs a little more before it gives us a well defined geometric number.
