Peg rotation
Can you work out what kind of rotation produced this pattern of
pegs in our pegboard?
Age
7 to 11
Challenge level
Problem
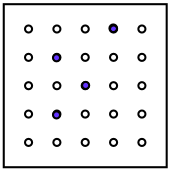
Here we have a kind of peg board. The holes go all the way through so the pegs may be seen from the top or underneath.
Image
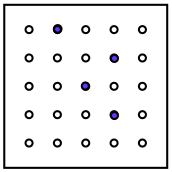
You'll see the 4 blue pegs.
Now the peg board is either:
- flipped over - north to south, OR east to west, OR north-east to south-west, OR north-west to south-east;
- or it is rotated clockwise or anticlockwise.
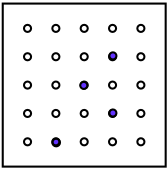
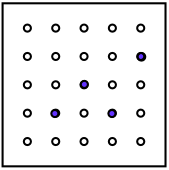
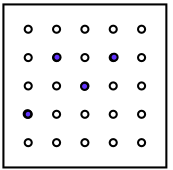
Can you find out which movements could produce the four next views?
Image
Image
Image
Image
You could make some of your own and get your friends to see if they can find how you've moved it to make your next picture.
Try now to put 4 pegs in so that when you do a move with the board it still looks the same.
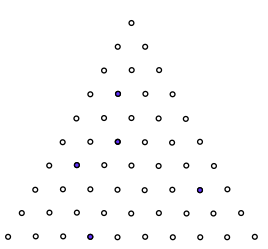
Now I've made a triangular board to do the same things.
Here's the starting view:
Image
How has it been moved to show these views?
Image
Image
Hope you've had fun exploring these.
Can you think up ways of changing what I've done so that you can explore further?
Getting Started
You might find it useful to draw a grid on an OHT or some tracing paper which you can see through.
Can you visualise the movements of each grid before physically checking?
Try matching up the dots on the original picture with the dots on the new image. It might help just to have the two pictures side-by-side.
Student Solutions
Jamie from St John's School told us:
1) It is flipped over from North to South.2) It is rotated 90 degrees clockwise.
3) It is rotated 90 degrees anti-clockwise.
4) It is flipped over from East to West.
Nik from Radley Primary School agreed with Jamie's answers, but also had some alternative ways of describing the transformations:
The first square can be flipped north-south or flipped east-west and then turned 180 degrees clockwise.The second square can be turned 90 degrees clockwise or turned 270 degrees anti-clockwise.
The third square can be turned 270 degrees clockwise or turned 90 degrees anti-clockwise.
The fourth square can be flipped east-west or flipped north-south and then turned 180 degrees clockwise.
For the triangular challenges, Callum from St. Patrick's R.C. told us:
"The triangle is turned:
1.Anti-clockwise for one turn, or clockwise for two
turns.
2.Clockwise for one turn, or anti-clockwise for two
turns."
Teachers' Resources
Peg Rotation
Here we have a kind of peg board. The holes go all the way through so the pegs may be seen from the top or underneath.
Image
You'll see the 4 blue pegs.
Now the peg board is either:
- flipped over - north to south, OR east to west, OR north-east to south-west, OR north-west to south-east;
- or it is rotated clockwise or anticlockwise.
Can you find out which movements could produce the four next views?
Image
Image
Image
Image
You could make some of your own and get your friends to see if they can find how you've moved it to make your next picture.
Try now to put 4 pegs in so that when you do a move with the board it still looks the same.
Now I've made a triangular board to do the same things.
Here's the starting view:
Image
How has it been moved to show these views?
Image
Image
Hope you've had fun exploring these.
Can you think up ways of changing what I've done so that you can explore further?
Why do this problem?
This challenging activity offers a context in which learners can apply knowledge of transformations. Although best done practically for many pupils, some may draw on visualisation skills, at least to make hypotheses before confirming practically. The triangular arrangement of dots helps to familiarise pupils with different angles.Possible approach
This activity, as well as being done in ones or twos by the pupils, could be introduced by you challenging all the class at once. The first picture could be presented on screen, and you could ask the pupils what they would have to do with it to to see the next two pictures. You could provide pairs or small groups of pupils with an acetate grid and some dry-wipe pens to create each image for testing.You may wish to download and print these sheets for pupils:
PegRotation.pdf
PegRotation2.pdf
(The first contains just the square grids, the second contains the triangular grids.)
Key questions
Which peg/s might be helpful to focus on to begin with?Have you tried flipping/rotating the original grid?