Games related to Nim
This small group of activities is taken from the Mathematical Games Archive on the NRICH site. They all have a related structure that can be used to develop the skills of strategic planning and reasoning as well as ideas of analogy.
Students at all levels of ability and age can access them. At the most basic level, they offer opportunities for practising arithmetical skills. At a higher level, they can be used to promote mathematical discussion by demanding detailed and reasoned explanations for a winning strategy, or an explanation of the mathematics that links the games.
Suggested Progression
Links
Links to the Framework
- Solve mathematical problems or puzzles, recognise simple patterns and relationships, generalise and predict.
- Understand addition and subtraction mental calculation strategies.
- Use letter symbols to represent unknown numbers or variables.
- Represent problems mathematically.
- Explain and justify methods and conclusions.
- Use logical argument to establish the truth of a statement.
- Solve increasingly demanding problems and evaluate solutions.
- Present a concise, reasoned argument, using symbols, diagrams, graphs and related explanatory text.
- Suggest extensions to problems, conjecture and generalise.
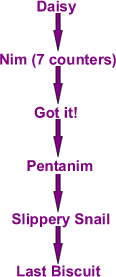
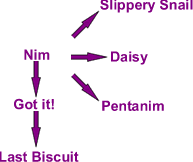
Daisy
NIM
Got It!
Play the game on line.
Start with the Got It! total of 15, using the numbers 1, 2, 3, 4 or 5.
Try to work out a winning strategy.
Does it matter who goes first?
What is the connection between NIM and Got It!?
Now change the target number, but keep the numbers 1, 2, 3, 4 and 5.
Does this alter whether it is preferable to go first or second?
Can you write a mathematical statement to link the target number to the winning player?
Try to find a generalisation for who would win for any target number, and any group of consecutive numbers from 1 to b.
Try to find a generalisation for who would win for any target number, and any group of consecutive numbers from a to b.
Slippery Snail
Pentanim
Last Biscuit
Further activities linked with these on NRICH:
