Domino magic rectangle
Domino Magic Rectangle
An ordinary set of 28 dominoes can be laid out as a 7 by 4 magic rectangle in which the spots in all the columns total 24 while the spots in all the rows total 42. Try it!
You may like to use this domino set or if you would prefer to use a computer interactivity, you might find our Dominoes Environment useful.
Printable NRICH Roadshow resources: Instructions and Gameboard
Domino Magic Square
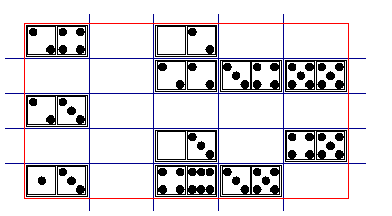
Magic squares can be made with the 25 dominoes remaining when you have put aside (0-5), (0-6) and (1-6). The total of each row, each column and each diagonal is 30. This can be done in many different ways. As a group project you might like to see how many distinct magic domino squares you can find.
Here is part of one layout to get you started.
Create your own game
Can you invent a game involving dominoes and magic squares? If you invent a good game do tell NRICH and we'll publish it on this page.
Thank you to everybody who sent in their solutions to this activity. There are a lot of different possible magic rectangles so you might be able to find one that we haven't shown here!
We received a lot of solutions from the children at Ganit Kreeda in Vicharvatika, India - thank you all for your hard work on this. Adithya sent in this explanation and picture:
First, I managed to get 24 in all columns. The rule which I used is - I noticed that only if we get the sum of the dots on the dominoes as 2 even and 2 odd or all odd or all even, we can get the sum as 24 without falling short of dominoes.
Well spotted, Adithya! There is one mistake in this magic rectangle, and it can be fixed if two dominoes are swapped. Can you see which two dominoes they are?
We also received the following magic rectangles, from Kanaa, Ruhi and Aarav respectively:
Thank you all for sending these in. It's really interesting to see the different ways you recorded your arrangements.
Milan from Sunmarke School in India sent in this picture and explanation:
We put alternately big and small dominoes for 6 columns and three rows. We then tried to put the remaining dominoes and where needed tried to swap them within the same row or the same column.
Good ideas, Milan! I wonder why alternating 'big' and 'small' dominoes is a good strategy?
Shaunak from Ganit Manthan in Vicharvatika, India sent in this solution:
You might also like to take a look at the domino game that Shaunak created. The rules are also explained in the video below.
Thank you for sharing this game with us, Shaunak - it looks like a very interesting way of creating a domino magic square!