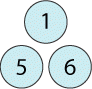
Difference
Problem

|
|||||||
Getting Started
Think about where the highest number might go.
You could use this sheet of blank pyramids to try out your ideas.
Student Solutions
Craig from Brookdale Primary gave this solution:

Perhaps you can find other ways of doing it?
Here is a solution that came in from a pupils in Stage 3 at Burke Ward Primary School, NSW Australia.
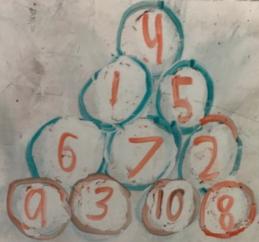
Thank you for that extra solution.
During the 2020 lockdown we had this sent in from a teacher who works at Woodmansterne Primary School, she wrote:
One of my newly-returned pupils has found another solution to the problem 'Difference' which we thought you'd like to see! She is called Melissa, and demonstrated excellent resilience with this problem.

Thank you for that, well done Melissa, and thanks to the teacher for submitting it. It's a good solution.
Teachers' Resources
Why do this problem?
Possible approach
The plenary can be used to discuss whether there are different solutions, possibly using the interactivity.
Key questions
Does thinking about odd and even numbers help you?
Possible extension
Possible support