Big and Small Numbers in Chemistry
Problem
Chemistry makes good use of numbers both small and large. Try these questions involving big and small numbers. You might need to use standard physical data not given in the questions. The questions all involve some aspect of approximation, although you might be able to give rather accurate upper and lower bounds on the actual quantities in some cases. In all cases it
is good to be clear in your own mind as to the precision of the answers given, and use an appropriate level of rounding in each case.
1) How many copper atoms are there in a one pence piece? How many electrons are there?
2) What volume of air would you have to contain to have enough argon to fill a lightbulb?
3) If you cooled all of the air from part 2 until none of the matter was in gaseous form, what volume would it occupy at standard pressure?
4) A Teflon monomer looks like this:
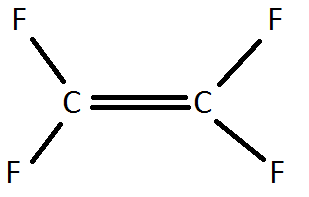
Draw a Teflon polymer. What percentage, by mass, of Teflon is carbon?
5) If the C-C bond length in Teflon is about 140pm (1pm = $10^{-12}m$), how many fluorine atoms would there be in a polymer measuring 1cm in length? How many moles of $F_{2}$ gas would contain the same number of fluorine atoms?
6) Alkali metals (group 1) react with water to produce MOH (M is the symbol used for an unknown metal) .Write a reaction for this. What gas is produced? What volume of water would you have to use in this reaction to produce 1.5 moles of gas?
7) A hydrogen atom has a radius of 120pm . Its nucleus has a radius of 1.75fm (1fm = $10^{-15}m$). What is the rest of the atom made up of? Estimate the percentage of the volume of a hydrogen atom that is empty space.
8) 25.5% of the atoms in your body are oxygen. This is equivalent to 65% of your body weight! If 9.5% of the atoms in your body are carbon, what percentage of your body weight is this? Estimate how many atoms there are in your body in total.
9) Looking at questions 7 and 8, if all of the atoms in your body were placed end-to-end, how long would the line be? Can you think of (or find out about) something that is that far away from you now?
Student Solutions
1) According to the Royal Mint, a copper coin weighs 3.56g. The relative atomic mass (RAM) is 63.5. The coin therefore contains approximately $\frac{3.56}{63.5}\times6.02\times10^{23} = 3.28\times10^{22}$ copper atoms. Assuming each atom is neutral, each atom contains 29 electrons, so there are $9.52\times10^{23}$ electrons in one coin.
2) I estimated that a lightbulb contains 100ml of air. The mole fraction of argon in dry air is 0.00934. We therefore need $\frac{100}{0.00934}ml = 1.07\times10^4ml = 10.7l$ of air.
3) Assuming the density of air is around 1.2kg/m$^3$, the mass of the air from q2 is $1.2\times10.7\times10^{-3}$kg$ = 1.3\times10^{-2}$kg. If this is cooled to a temperature where it's all liquid and it's kept at standard pressure, we can assume it has the standard density of liquid air: 870 kg/m3. It would approximately occupy $\frac{1.3\times10^{-2}}{870}m^3 = 1.5\times10^{-5}m^3 = 0.015l$.
4) The percentage mass of carbon in teflon is $100\times\frac{2\times12}{2\times12+4\times19} = 24\%$. There's an image of the polymer here.
5) The number of molecules of the monomer in a chain of length 1cm is $\frac{0.01m}{140pm\times2} = 3.5\times10^7.$ There are therefore $1.4\times10^8$ atoms of fluorine in such a chain.
One mole of $F_2$ gas contains 2 moles of F atoms. The number of moles of fluorine gas is therefore $\frac{ 2.8\times10^8}{2\times(6\times10^{23})} = 2\times10^{-16}$ moles.
6) The reaction is 2M (s) + 2H2O (l)$\rightarrow$ 2MOH (aq) + H2 (g), producing hydrogen gas. To produce 1.5 moles of gas, we'd need 3 moles of water, which weighs 54g and hence has volume 54ml.
7) The nucleus takes up a percentage volume $100\times\frac{\pi\times(1.75fm)^3}{\pi\times(120pm)^3} = 3\times10^{-13}\%$. The rest of the atom is a cloud of negatively charged electrons, which have much, much smaller mass than the nucleus.
8) Given the percentage mass of oxygen of your body is 65%, the percentage mass of carbon of your body is $65\times\frac{9.5}{25.5}\times\frac{12}{16} = 18$%. (This accounts for carbon being less abundant, and also a carbon atom is lighter than an oxygen atom.)
Suppose the average atomic mass is 10gmol$^{-1}$. Then the number of atoms in a human weighing 80kg is $\frac{8\times10^4}{10}\times6\times10^{23} \approx 5\times10^{27}$.
9)A lower bound for this distance is $5\times10^{27}\times120pm = 6\times10^{17}m$ (as hydrogen is the smallest atom). This is about 63 lightyears (the distance light travels in 63 years). There is apparently a masssive planet this distance away.
