Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Get in Line
Age 14 to 18
Challenge Level 





- Problem
- Student Solutions
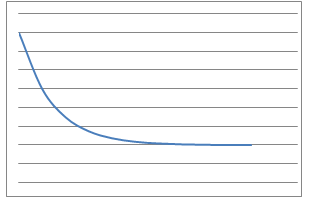
1. A cup of tea is made and the temperature measured in degrees Celsius every second. What would the temperature-time graph look like?
$$y=\frac{A}{2^t}+B$$
y = temp, t = time
2. The pendulum of a grandfather clock swings to and fro and the angle of the bob from the vertical is measured every 100th of a second. What would the angle-time graphs look like?
$$y = A \sin(Bt)$$
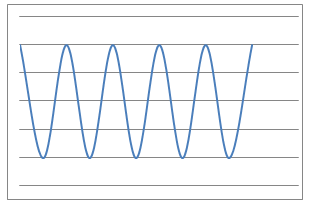
y = angle, t = time
3. I throw a tennis ball straight up into the air and catch it. The height of the ball from the ground is measured over the time of the journey using freeze-frame photography. What would the height-time graph look like?

$$y=Ax^2$$
y = height, x = time
4. I measure several objects using inches and then using metres. What would the inches-metres graph look like?
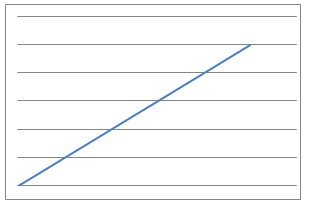
$$y = Ax$$
y = inches, x = metres
5. I jump out of a plane and the distance fallen from the plane is measured every $0.1$ second until I open my parachute. What would the distance-time graph look like whilst in freefall?

$$y = A x(x-B) + C$$
y = distance, x = time
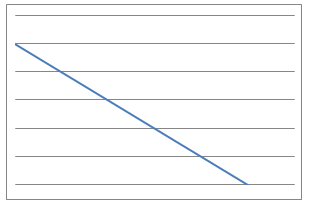
6. I drive at 70 miles an hour along the motorway and note the reading on my mileometer every 5 minutes. What would the plot of mileometer v minutes passes graph look like?
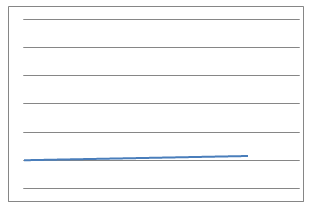
$$y = Ax+B$$
y = milometer, x = minutes
7. I bring two magnets of the same polarity together directly in a line in a sequence of steps. Starting from 1 metre, I halve the remaining distance each time and measure the force felt between the magnets. What would the force-distance graph look like?
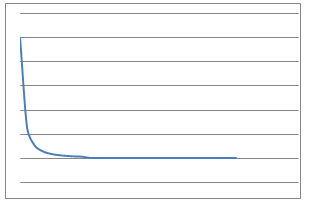
$$ y=\frac{A}{x}$$
y = distance, x = force
8. I blow up a roughly spherical balloon using a balloon pump. After each pump I measure the radius of the balloon in centimetres. What would the number-of-pumps vs radius graph look like?
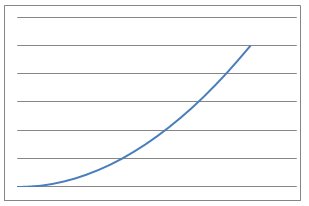
$$y=A r^3$$
y = no. of pumps, r = radius
9. I suck water through a straw out of a large beaker at a constant rate and measure the volume of liquid remaining at various times. What would the volume-time graph look like?
$$y = Ax + B$$
y = volume, x = time