Painting between the lines
In abstract and computer generated art, a real object can be
represented by a simplified set of lines. Can you create a picture
using mathematical instructions?
Problem
In abstract or computer generated art, a real object is often represented by a simplified set of shapes, lines or curves.
Take a look at the picture below:
Image

The picture could be represented in an
abstract way using straight lines:
Image
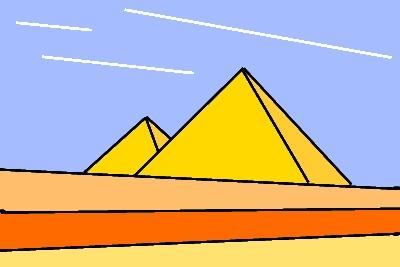
If you wanted to describe mathematically the line segments used to draw this picture, all you would need to do is decide where to put the axes and then work out the equations of all the straight lines.
Here is a set of instructions to create an abstract representation of another well known landmark (download as a Word or PDF file).
Now try this for yourself - choose an image, perhaps a photograph of a famous location, or a famous painting.
Think how it might be broken down into shapes or segments of lines.
Can you create a set of instructions for your picture?
Once you have created your instructions, give them to a friend to follow.
Can you devise a way to describe mathematically each region so that you can give instructions for colouring your image?
You may wish to use curves as well as straight lines. To explore abstract art using more complicated mathematical functions, take a look at Painting by Functions.
Have a go at creating your image using a graphing software package such as Geogebra.
Please send us any of your creations (together with your instructions).
Getting Started
You may find it useful to explore the equations of straight lines
using the interactivity in Parallel Lines.
Student Solutions
Here is the well-known landmark given by the instructions:

Image

Teachers' Resources
Why do this problem?
This problem gives
an engaging opportunity for genuine cross-curricular links between
art and mathematics whilst bringing in ideas about the equations of
straight line graphs. By considering pictures made up of curves as
well as lines there are natural extensions into transformation of
simple functions, and thinking about how to describe shaded parts
can lead into work on inequalities.
Possible approach
In working on this
problem learners will need to be fluent in expressing equations of
straight lines - Diamond Collector
offers a good exercise to practise this.
Learners could be set a
homework task before the lesson of finding an image which could be
represented in an abstract way using straight lines (or very simple
curves such as parabolas) - the photo and the abstract image used
in the problem could be given as an example.
Begin by discussing with
the class the abstract image of the pyramids. The image could be
projected onto a whiteboard and the class could decide together on
an origin and scale for the axes. Ask them to discuss in pairs how
they would write a set of mathematical instructions to create the
image (or how they would create it using graphing software), and
then share ideas with the whole class.
Key concepts to draw out
would be the use of equations of straight lines, using inequalities
to express which parts of a line to draw as line segments, using
inequalities to describe which sections to colour in each shade,
and where the class would choose to put the axes.
Now hand out this
worksheet (Word, PDF) which contains
instructions for drawing another well-known landmark. Suggest that
learners work in pairs to complete the image. One possible
extension at this point is for them to devise a way of using
inequalities to describe a way of colouring the picture.
Then give the class
plenty of time to take an image of their own and turn it into an
abstract image on squared paper with axes, perhaps working in pairs
again. Learners who have met equations of quadratic graphs could
create an image using parabolas as well as straight lines. They
could then write out a set of instructions to draw their image and
challenge another pair to recreate it without seeing the original.
Graphing software could be used to verify the equations of lines
that learners choose to use.
Another approach could be
to give everyone the same image to recreate using lines and simple
curves, and then liaise with some teachers and students of art to
choose which is the best abstract representation of the original
image.
Key questions
How can the image be
recreated using only straight lines?
Which lines have a
positive gradient? Which have a negative gradient?
Which lines are steep?
What might their equations be?
How can I move the lines
up or down the y axis?
How can I describe a line
segment rather than a whole line?
