Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Holly



The ten arcs forming the edges of this "holly leaf" are all arcs of circles of radius 1 cm. At all the spiky points the circles touch each other tangentially. All the straight lines join the centres of the circles they pass through and the four triangles at the corners have angles of 45, 45 and 90 degrees.
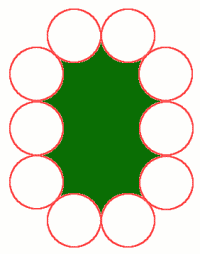
Find the length of the perimeter of the holly leaf and the area of its surface.
This is a 10-spike holly leaf. What is the perimeter of a 16-spike holly leaf of the same sort?
Examine some real holly leaves and you will find that they don't lie flat. This shape is different from a real holly leaf in so far as it does lie flat on a flat surface (or plane). See ' Giant Holly Leaf' for an extension of this problem to a more realistic holly leaf which has negative curvature.

