Pythagoras proofs
Can you make sense of these three proofs of Pythagoras' Theorem?
Problem
Pythagoras' theorem states that:
If a triangle with sides $a, b, c$ has a right-angle, and $c$ is the hypotenuse,
$a^2+b^2=c^2$
Here are three different diagrams which can be used to prove Pythagoras' Theorem.
Can you make sense of them?
Which proof do you find most "convincing"?
Which do you find easiest to understand?
Method 1:
Can you use the picture below and the proof sorter to create a proof of Pythagoras' theorem?
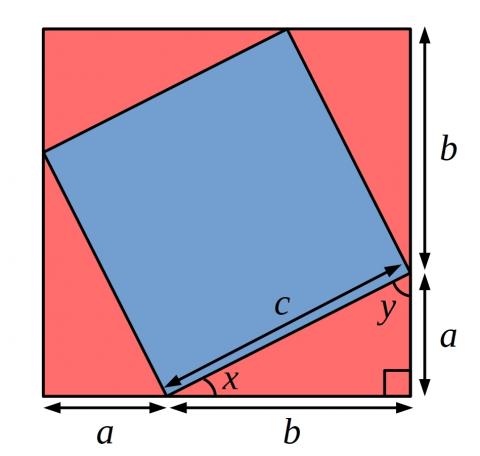
Method 2:
Can you use the picture below to come up with another proof of Pythagoras' theorem?

Method 3:
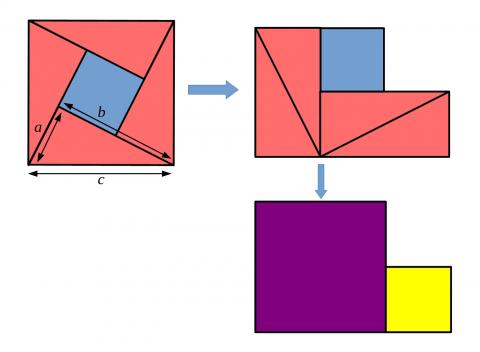
This time the four right-angled triangles have been arranged in a different way. Can you use this picture to create a third proof of Pythagoras' theorem?
Method 4: Another method of proving Pythagoras' Theorem can be found in the problem "A Matter of Scale"
You might also like to explore the problem "The Converse of Pythagoras"
You can find some videos and apps illustrating methods 2 and 3 in this Plus article "Seeing Pythagoras"
If you can find a different proof of Pythagoras' Theorem then please do let us know by emailing us.
Getting Started
What exactly are you trying to prove?
What do you know? What can you work out from what you know?
Student Solutions
Method 1
Boyi from Wellington in Malaysia, Fiona from Wellington College International Shanghai in China and Lewis from Wilson's School in the UK used the picture below and the proof sorter to create a proof of Pythagoras' theorem. Fiona wrote:

This proof is shown by making a square that has $c$ length on each side, putting 4 identical triangles around the square, forming a bigger square. We then can know that the four triangles’ area adds together to form the square in the middle, which is $c^2.$ Therefore, the area of the enclosed square = the area of large square – area of the four triangles.
The area of the large square is: $(a+b)^2=a^2+2ab+b^2,$ and the area of the four triangles is: $4\times\frac12\times ab=2ab.$
Then we can know that the area of the enclosed square is: $a^2+2ab+b^2-2ab=a^2+b^2.$ The area of the enclosed square is also given by $c^2,$ therefore $a^2+b^2=c^2.$
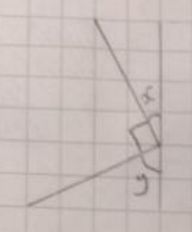
There is one piece of information that is missing from Fiona's proof. It is not clear that, starting from the small blue square, when you add four triangles, you get a square (and not some other shape). In particular, Fiona's proof is missing the fact that the 'sides' of the 'bigger square' are straight lines.
Lewis used this diagram to demonstrate this idea (along with the information that, since they are the angles in a right angled triangle, $x+y=90^\circ$):
Method 2
Boyi, Fiona and Lewis used the picture below to come up with another proof of Pythagoras' theorem. Fiona's proof is below the diagram.

First, the area of the first square is given by $(a+b)^2$ or $4\left(\frac12 ab\right) + c^2.$
The area of the second square is given by $(a+b)^2$ or $4\left(\frac12 ab\right) + a^2 + b^2.$
Since the squares have equal areas we can set them equal to another and subtract equals. $4\left(\frac12 ab\right) + a^2 + b^2 = 4\left(\frac12 ab\right) + c^2$
Subtracting equals from both sides we have $a^2+b^2=c^2,$ which proves the Pythagoras theory.
Method 3:
Some people tried to use this picture to create a third proof of Pythagoras' theorem, but wrote that the side length of the blue square was $a.$

This is Boyi's proof, which doesn't use the third diagram at all:
This is Fiona's proof, with a diagram from Lewis's proof added. Two more lengths have been drawn on to Lewis's diagram, for clarity.
The first square has an area of $c^2,$ and we can look the second shape as two squares – One small square (on the right) and one big square (on the left), shown on the last shape.

Then, this shows $a^2+b^2,$ and we can see that the two area are equal, so $a^b+b^2=c^2.$
Method 4
Another method of proving Pythagoras' Theorem can be found in the problem "A Matter of Scale".
Well done to Andrew from Island School, who demonstrated how this works.
Fiona sent in some opinions about which proof was most convincing and which was easiest to understand:
Which proof is the most "convincing"? – In my opinion, I think that second one is the most "convincing" because it uses a lot of math equations to show the proof.
Which proof is easier to understand? – I think that the last one is the most interesting and easy to understand because it basically uses shapes to illustrate The Pythagoras theorem.
Teachers' Resources
Why do this problem?
This problem shows three different approaches to Pythagoras' Theorem, and links to a fourth one (A Matter of Scale). It could be used with a group who have recently met the theorem, to provide a variety of ways of thinking about it, or with a group who are familiar with the theorem, to explore different proofs. There are great opportunities for communicating mathematical ideas and debating which method appeals the most and why!
There is also a link to a proof of the Converse of Pythagoras' theorem, which uses proof by contradiction.
Possible approach
This problem featured in an NRICH Secondary webinar in March 2022.
These printable cards for sorting may be useful: Pythagoras Proofs
Divide the class into small groups and assign each group one of the proofs to work on. Explain that once they understand how the proof works they will be asked to produce a poster or presentation to persuade others of the value of their proof.
After allowing plenty of time for exploring their proof and producing their explanation, bring groups together so that each group can present their proof to others who have worked on a different proof. Everyone should get the opportunity to see all three proofs explained. Encourage learners to be (constructively) critical of each other's proofs and to question points that aren't clear.
Finally, bring the whole class back together for a vote on which proof they thought was the clearest and most satisfying, and a discussion on the merits of each proof.
Key questions
What exactly are you trying to prove?
Possible extension
Read more about proof here.
Possible support
Tilted squares can be used to introduce Pythagoras' Theorem.
