Curve match
Which curve is which, and how would you plan a route to pass between them?
Problem
The following five functions have been plotted accurately on the chart below
Image
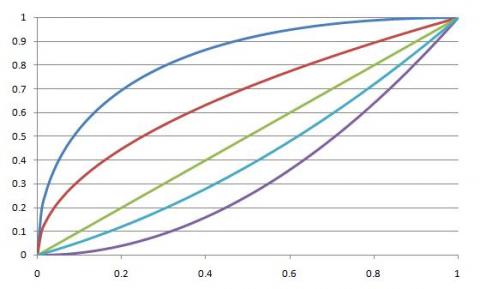
Which is the $x$-axis and which is the $y$-axis? Which curves correspond to which functions?
Imagine that you wish to plot a route between $(0, 0)$ and $(1, 1)$. Can you find a function which does this without intersecting any of the existing curves, except at the endpoints?
What other curves can you plot which only intersect the existing curves at the end points?
Getting Started
The green graph should be straightforward to identify.
A little bit of numerical detective work should help you to identify which axis is which.
A little bit of numerical detective work should help you to identify which axis is which.
Student Solutions
We really liked this solution sent in from Alex from Stoke on Trent Sixth Form College:
The horizontal axis is the $x$-axis, and the vertical axis the $y$-axis.
I used $x=0.5$ to calculate the y values and ordered them.
The curves, from top to bottom on the graph, are the functions arranged in decreasing order of $y$ value for any $x$ value between $0$ and $1$ when they are equal.
The order becomes $2\sqrt{x}-x\,,\sqrt{x}\,, x\,, (x^2+x)/2\,, x^2$
Any function of the form $x^n, n> 2$ will return smaller $y$ values than $x^2$ between $0$ and $1$; avoiding the existing curves and intersect at the end points. $x^{\frac{1}{n}}\,, n> 2$ is similar, instead returning values higher than $x^{\frac{1}{2}}$.
Functions of this form also meet the conditions. To generate a curve $c(x)$ such that $a(x) < c(x) < b(x)$ for $0 < x < 1$, and $a(0) = b(0) = c(0), a(1) = b(1) = c(1)$, the arithmetic mean could be used. $c(x) = (a(x)+b(x))/2$, or a more general $c(x) = a(x) + k(b(x)-a(x)), 0 < k < 1.$ $k = 0.5$ becomes the arithmetic mean. This formula can be used with $a(x)$ and $b(x)$ as adjacent existing curves (such as $a(x) = x, b(x) = \sqrt{x}$). The existing functions $\sqrt{x}$ and $(x^2+x)/2$ could be thought of as having been generating by using $2\sqrt{x}-x$ and $x$, and $x$ and $x^2$, respectively. $a(x) = 0, b(x) = x^2$, and $a(x) = 2\sqrt{x}-x$, $b(x) = 1$ produces $c(x)$ curves below and above existing curves.
Resulting $c(x)$ functions from using the general formulae (or the specific arithmetic mean case) can be used as the $a(x)$ or $b(x)$ functions in generating others, resulting in many different functions involving $x, \sqrt{x}$ and $x^2$ terms.
However, there are other functions in addition that meet the conditions, such as the binary logarithm. $y = \ln(x+1)/\ln(2) = \log_2(x+1)$ Between $0$ and $1$, $x < \log _2(x+1) < \sqrt{x}$, therefore it does not intersect the curves except for the end points where: $\log_2(0+1) = 0$ and $\log_2(1+1) = 1$.
Simon said
The $x$ axis is horizontal and the
$y$ axis is vertical.
Royal blue $y = 2\sqrt{x} -x$
Dark orange $y = \sqrt{x}$
Lime green $y = x$
Turquoise $y =
\frac{x^2+x}{2}$
Purple $y = x^2$
There are an infinite number of curves that can be found that
will intersect only at the two end points.
One potential form is $y = \frac{x^2+ax}{1+a}$ for $a \neq
1$
Teachers' Resources
Why do this problem?
This challenge could be used as a lesson starter to check students' ability to match curves with their equations. Finding other functions which do not intersect with the existing curves except at the endpoints can help students to develop a stronger sense of how to manipulate functions and the corresponding effect on the graph.
Possible approach
This problem lends itself to discussion as a class or in small groups. Give students a short time to consider how to identify which is the $x$ and which is the $y$ axis, then share reasoning.
Are there any of the curves they can identify straight away? The curves could be matched using some numerical work to identify key points on the graphs. Alternatively, students could consider how they could sketch the less familiar curves by transforming the functions which are more recognisable.
Considering the relationship between the functions which were chosen for the problem may lead to insight into how to create more curves with the same endpoints which do not intersect at any other point in the region. Students could use graph-plotting software to sketch these graphs and explain how they know there are no other points of intersection.
Key questions
Are there any curves which can be identified straight away?
Which curves have similar shapes to each other? How can they be distinguished?
Is there a relationship between any of the curves?
Possible extension
Come up with ways of generating curves between any pair on the grid, and prove that there are no intersections except at the end points.
Possible support
Work numerically to plot a few key points for each given function, and use these to identify the $x$ and $y$ axes and each curve.
