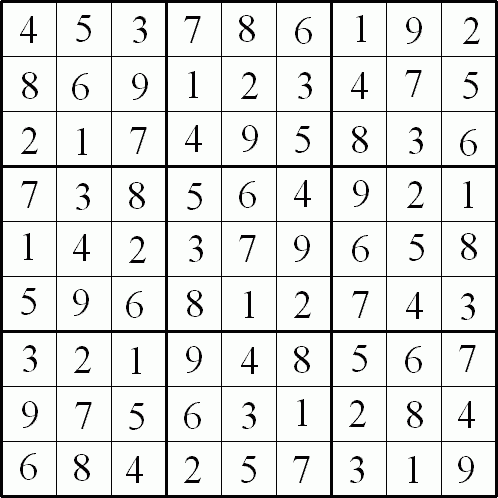
Another Quadruple Clue Sudoku
This is a variation of sudoku which contains a set of special clue-numbers. Each set of 4 small digits stands for the numbers in the four cells of the grid adjacent to this set.
Problem
By Henry Kwok
Image
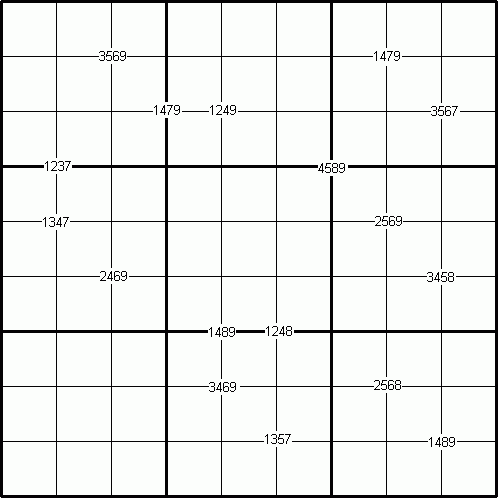
Rules of Quadruple Clue Sudoku
This is a variation of sudoku on a "standard" $9 \times 9$
grid which contains a set of special clue-numbers. These are small
numbers provided by sets of $4$ small digits.
Each set of $4$ small digits in the intersection of two grid
lines stands for the numbers in the four cells of the grid adjacent
to this set.
The remaining rules are as in a "standard" sudoku, and the
object of the puzzle is to fill in the whole $9 \times 9$ grid with
numbers $1$ through $9$ so that each row, each column, and each of
the nine $ 3\times 3$ boxes must contain all the nine different
numbers $1$ through $9$.
For example, in the puzzle, taking the two sets of adjacent
cells with small digits {$1479$} and {$3567$}, we find that they
overlap at the cell with the number $7$. The rest of the puzzle is
solved in the same way through logical deduction using the usual
sudoku techniques and by determining the numbers in the cells in
one set that overlap the cells in other sets.
Student Solutions
Image