Random inequalities
Can you build a distribution with the maximum theoretical spread?
Problem
In this problem we look at two general 'random inequalities'.
Part 1
Markov's inequality tells us that the probability that the modulus of a random variable $X$ exceeds any random positive number $a$ is given by a universal inequality as follows:
In this expression the exponent of the denominator on the right hand side is missing, although Markov showed that it is the same whole number for every possible distribution. Given this fact, experiment with the various distributions to find the missing value (??).
Part 2
Another important general statistical result is Chebyshev's inequality, which says that
Getting Started
Student Solutions
James Bell from the MacMillan academy was the first person to crack this toughnut, with this solution. He also noted that the problem as it stood only held for positive random variables -- well spotted. Well dones James!
For the first part James cleverly used random variables which only took a single value to work out that $??$ must equal $1$:
Part 1:
Consider $X$ such that $P(X=10)=1$ $E(|X|)=10$ taking $a=9$ we get left hand side equal to $1$ so the right handside must equal at least $1 =\frac{10}{9^{??}}$. If $??$ were $2$ or greater this wouldn't be satisfied.
Now consider $P(X=0.5)=1$ so $E(|X|)=0.5$. Taking $a=0.45$ we get left hand side equal to $1$ so the right handside must equal at least $1 =\frac{0.5}{0.45^{??}}$. If $??$ were $0$ or less this wouldn't be satisfied and so $??=1$.
Part 2:
Our task is to design a distribution such that the chance of $X$ being at least $2\sigma$ from the mean is maximised.
James first argues that a distribution which satisfied the inquality exactly must have a maximum value of $2\sigma$ by shifting probability around. Some more details would be needed for a fully convincing explanation, but the argument is in essence correct and shows very sophisticated statistical reasoning .
If a solution existed where $X$ could take a value greater than $2\sigma$ from the mean then we could use this to create a new distribution $Y$ by changing the values of events leading to a difference being greater than $2\sigma$ to values leading to a difference of $2\sigma$, thus making the probability of the difference being greater than $2\sigma$ zero. Of course, this would decrease $2\sigma$ for $Y$; some of the probability for $Y$ could be moved from near the mean to outside $2\sigma$ for $Y$ without moving $2\sigma$ for $Y$ as far out to where it was originally for $X$, meaning that the probability of $|Y-\mu|$ being greater than $2\sigma$ would increase above $\frac{1}{4}$. As we know this to be impossible, any such distribution must have $P(|X-\mu|> 2\sigma)=0$.
With this insight, James was able to search for a solution
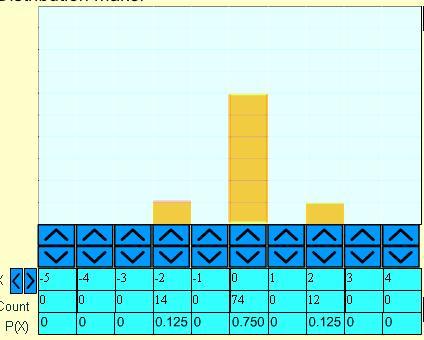
Assuming symmetry, to find a solution let us set
as together they must add to $0.25$ or $\frac{1}{4}$ the other $\frac{3}{4}$ must be distributed right on the mean in order to makle the variance as small as possible which suggests the distribution attached for $\sigma=1\,, \mu=0$, and sure enough calculating sigma from the distribution gives $\sigma=1$ as well confirming that it works.
Here is the picture:
Image
Teachers' Resources
Why do this problem?
This problem requires students to invent a distribution to satisfy certain criteria. In doing so, they will strongly reinforce their understanding of the meaning of distributions as a whole. Students will have to think creatively and really engage with the relationship between a distribution and the probabilities in order to fully solve the problem. The fact that universal order exists underlying all random processes (i.e. the inequalities) is interesting; instead of a collection of diverse results, distributions become part of a coherent whole.Possible approach
A main part of this challenge is understanding the meaning of the question. Spend time with the group exploring what they feel that question is asking and encouraging them to work through the meaning of the parts of the question. Once they have a feel for the question they might want to proceed by:
- Looking at the inequalities for some known distributions (such as the binomial or the normal)
- Investigating other simple probability distributions (such as that obtained on the roll of a die)
- Creating distributions 'randomly' using the distribution maker and investigating the inequalities for the probability distributions obtained.
Key questions
- What is the problem asking?
- How can we use the condition that (??) is a whole number?
- Are then any obvious distributions to try out?
Possible extension
As a challenge, try to create a distribution which violates the inequalities. How close can you get? Does this give you a feel for why the inequalities hold? How close to the inequalities do distributions which the class already know about (such as binomial or normal) get?
You might like to refer keen students to the central limit theorem in probability, which shows that on average distributions tend to the normal.
