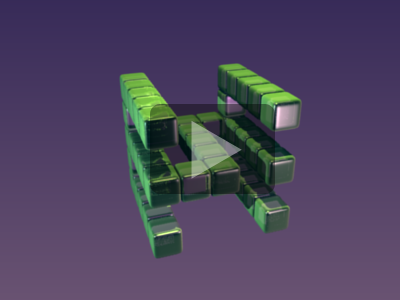
The perforated cube
Problem

Any cube may be removed so imagine some uncoloured, transparent cubes propping up anything that needs it.
If a 'perforated cube' has the three views (projections) below, what is the most and the least cubes possible to make a shape that has these three projections ? In other words what could be the most and the least cubes left from the original 125 ?
Getting Started
Student Solutions
Edison from Shatin School included some edited versions of the diagram given in the hints to support his argument:
Then you can take away blocks, checking each face projection so its unchanged.
On the far E, you can take away 4 on the top prong, 4 on the bottom prong, and the 1 back block on the middle prong. The middle of the S cannot be removed as it is needed for the S face. The on the close E you can take 4 from the middle prong, and then the back block on the top and bottom prong.
So we have removed $15$ blocks, and you cannot remove any more. So the minimum total is $41-15=26$

Well done Edison, can anyone think of any other interesting projections to aim for?
Teachers' Resources
This problem provides a rich context for visualisation and suggests many similar lines of enquiry.
For example :
-
Can all letters of the alphabet be represented using only a 5 by 5 array of cubes ?
-
Is it possible to create every combination of three letters in a 'perforated cube' ?
-
What difference does the orientation of the letters make ?
-
How many ways are there to orientate three non-symmetric letters such as F, J and P ?
-
What difference will it make to use letters that have symmetry of some kind ?
- Can every solution arrangement have either more cubes added or some removed, and still be a solution arrangement ?
- Is there a relationship between the maximum and minimum number of cubes for any solution ?
The information above is repeated on the Hint page which also contain this excellent video of one solution for E, S and H - video of an ESH solution