Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Published 2007 Revised 2018
Opening Out
In this article, Bernard Bagnall describes how to get more out of some favourite whole-class investigations found on NRICH.
The first investigation we will look at is Tea Cups . It has proved to be very popular both with pupils and with adults when working with other adults. Using squares within squares to record results, one of the most popular answers looks something like this:

and probably a fair amount of perseverance and playing around with ideas and strategies leads to the results that pupils achieve. It might have been a hard slog and then the lesson may come to an end at that point, but keep the result for looking at later.
So, later on, let's start opening it out by asking "Tell me what you notice about the result". I've found that the answers to this question usually include something about the positioning of 'same-colours'. So here we have the saucers and tracing the same colour in from the four corners we have something like this:

Continuing this idea we could link the cups in a similar way, and we'd have:
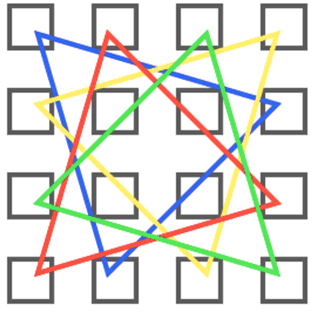
When these kinds of things [relationships] have been seen by some of the pupils it may be very useful to illustrate them in other ways - it can help the pupils who think differently. I've just drawn them here for your illustration, but you could use pegboards or nail [plastic] boards. I've sometimes found it good to 'go large' and have the 16 positions marked on the floor so that the whole layout is a couple of metres across and then I've used string to replace the lines [thick coloured wool would have been better]. Things like the middle bits show up really well and quite often lead to others noticing shapes and relationships. So in the first one the shape in the middle is an octagon:

The second a square:
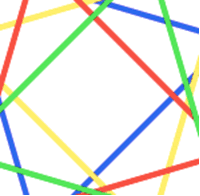
Using computers and isolating parts of the arrangement (as I have done above) will no doubt also reveal things.
So, what have we done?
1. Taken a result of an investigation.
2. Answered the question, "Tell me what you notice".
3. Taken some of those answers and illustrated them in different ways and again asked, "Tell me what you notice".
I have some thoughts about this kind of investigation. You may have done the investigation first, yourself, so as to feel more comfortable with it. This is like going on a trip to the local woodland area and making the journey yourself first to "check it out" before taking your pupils. You may notice all kinds of things when you go on your own. You are probably using many of your senses. Now, when the pupils are with you it's a temptation for you to lead their thoughts along the same path that yours have gone. But we really need to allow a lot of space for them to know that it's good for them to use their own senses and notice new things for themselves. Also it is good for them to discover for themselves the same things that you noticed. Thirty lots of senses should be able to pick up more! You never know beforehand but one child may detect a funny smell and then someone else is aware that it may be a fox! So the adventure goes on. Can we think about the maths investigation lessons in the same way?
Let's look at another investigation on NRICH, this time it's Sending and Receiving Cards. Well I've found that most children get to the solution by multiplying the number of people by one less than that number. That could be the end of it, but we can look for more patterns in the answers, just by asking "What do you see?".
When written out systematically, the results will throw up patterns such as the units going 2, 6, 2, 0, 0. [When such a pattern shows up it's worth asking the more advanced pupils WHY?] A lot of number pattern investigations are greatly improved - particularly from the children's point of view - when the patterns are explored as digital roots. Digital roots are formed by taking any number, say 243, and, ignoring place value, add up the digits until you only have a single digit - which is called its digital root! Many pupils have come across this in the nine times table with all the answers adding to 9. (You can read more about digital roots in this article.)
So 243 becomes 2 + 4 + 3 which is 9. 853 becomes 8 + 5 + 3 which is 16, which in turn becomes 1 + 6 which is 7. Although not directly linked to the original question, looking at digital roots is a very good way of opening up investigations in looking for patterns.
| Number of people | Cards |
| 2 | 2 |
| 3 | 6 |
| 4 | 12 |
| 5 | 20 |
| 6 | 30 |
| 7 | 42 |
| 8 | 56 |
| 9 | 72 |
| 10 | 90 |
| 11 | 110 |
| 12 | 132 |
| 13 | 156 |
| 14 | 182 |
| 15 | 210 |
| 16 | 240 |
| 17 | 272 |
| 18 | 306 |
| 19 | 342 |
| Number of people | Cards | Total cards |
| 2 | 2 | 2 |
| 3 | 6 | 8 |
| 4 | 12 | 20 |
| 5 | 20 | 40 |
| 6 | 30 | 70 |
| 7 | 42 | 112 |
| 8 | 56 | 168 |
| 9 | 72 | 240 |
| 10 | 90 | 330 |
| 11 | 110 | 440 |
| 12 | 132 | 572 |
| 13 | 156 | 728 |
| 14 | 182 | 910 |
Now we can open the question out and see where our imagination takes us. 4's and 7's - what else could we try? What borders of rectangles can we make?
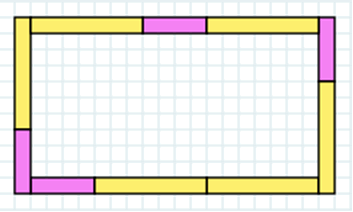
It's really a matter of having a grasp that the doing and exploring for the child is as - if not more - important than just getting an answer to a question. For this to work well the pupils need to have been shown in every way that they are allowed 'to think', not simply 'to remember'. So much value has to have been put on to the speaking and listening of the teacher - as well as the pupil.
Asking, "Tell me what you notice about 4, 6, 6 and 8?" may provoke answers such as:
1. "They're all even" - so maybe the response then is "Could we do the same kind of thing with all odd, OR with a mixture?". For some children you could ask, "What effects do you notice when using evens only, as apposed to a mixture of odds and evens?"
Such things are possible when teachers are confident enough to take little risks and to let the children do so too. Enjoy!!
This article also appears in Primary Mathematics, a journal published by The Mathematical Association.
Here is a PDF version of this article.

