Secret number
Annie and Ben are playing a game with a calculator. What was Annie's secret number?
Problem
This is a game for two players with a simple calculator.
Annie and Ben are playing. Annie puts her secret number into the calculator without showing Ben.
Annie then asks Ben, "What do you want to add?"
Ben tells Annie the number he wants to add: "I want to add four."
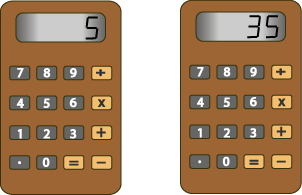
Annie presses the 'add' button and then the four button. The calculator now shows '4'. Annie gives the calculator to Ben.

Ben presses the 'equals' button and the calculator gives the answer '10'.

What was Annie's secret number?
How do you know?
You could play this with a friend. If you work out your friend's secret number correctly, it is your turn to put in a secret number of your own. You could score a point for every one you get right.
A multiplication version of the game might go like this:
Charlie puts in a secret number and asks Dana, "What do you want to multiply it by?"
Dana replies, "Multiply it by 5."
Charlie puts in 'times' and '5' and hands the calculator to Dana.
When Dana presses the 'equals' button the calculator shows '35'.


Dana now has to work out Charlie's secret number. What was it?
How do you know?
Try playing this version with your friend too!
Getting Started
Annie added $4$ to her number and it made $10$. What number added to $4$ makes $10$?
How could you check your answer?
When Charlie "timesed" his number by $5$, it came to $35$. What number multiplied by $5$ makes $35$?
How could you check your answer this time?
You could use a number line or a multiplication square to help you.
Student Solutions
We had a few solutions sent in, here is the one from Samiya from Boxgrove Primary School
The answer is 6
because 10 take away 4 equals 6.
Or we can say 4 plus 6 equals 10
Kaiand and Jase from Carrillo Elementary in America wrote;
Her secret number is six. I know this because she never pressed the "=" sign. She only put the + and the 4 and it showed the four. Then when he pressed the "=" sign, it became 10 because 6+4=10.
Abhishek, Xylia,Christopher, Alex, Grace, Seby and Connor from Pakuranga Heights Primary School in New Zealandsent in these two calculations.
4+6=10 5x7=35
Holly from Hermitage School sent a particularly well-explained one:
You know that the number on the addition question is 6 because of two things.
1. I know that 6 + 4 = 10, and 4 was the choosen number, so 6 is what you add on to make 10.
2. You can subtract 4 from 10 and see what the number is. That number is then the secret number, 10 - 4 = 6.
You know that the number on the multiplication question is 7 because of 2 things.
1. I know my 5 times tables and I went through them, {5x1=5,2x5=10,3x5=15,4x5=20,5x5=25,6x5=30,7x5=35}. When I got to 35 I figured out what you times it by.
2. Another way to do it is to do division. 35 divided by 5 is 7. Also if you find division hard you can do 5 jars and 35 sweets.
I think Holly means that you can think of division as sharing, for example sharing out 35 sweets between 5 jars.
Benjy from St Mary Redcliffe Primary looked at the first addition game more generally. He said:
If I ask to add one to the secret number then I can get the number from the answer by taking away one.
If I ask to add one hunded to the secret number then I can get the secret number from the answer by taking away one hundred.
Well done, Benjy - you have got the idea. Charoo from Manor Farm Junior School summed it up by saying:
You know the operation and you know what other number they're using so you do the inverse!
That is indeed one way of doing it, as Holly has shown. Thank you again to all of you who contacted us.
Teachers' Resources
Why do this problem?
This game is a good one to play with young children once they are familiar with the basic number operations. They will like the idea of their number being "secret", and of course being able to work out someone else's "secret" number! Looking for a ''secret'' number is the basis for algebra and solving unknowns in equations. So as well as enjoying what they are doing, your class will be engaging with some important mathematical ideas.
Possible approach
A good way to start might be for you either to enter your own secret number and invite the class to suggest what to add, or perhaps ask two children to come to the front to demonstrate. This activity will create a great opportunity for rich discussion amongst the class about how they can work out the secret number. You could ask the children to think for themselves first, then share their ideas with a partner and finally with the whole group. (Think-pair-share.)
Key questions
Possible extension
The best extension for this is for children to play the game with a partner as they are invited to do in the original problem. For some, there need be no limit to the numbers or operations involved. However, it would be a good idea to get all "secret" numbers and working out recorded!
Possible support
Use a calculator openly with the children so that they can see exactly what is happening. When they understand the mechanism of the game then start using a "secret" number with very simple numbers. Learners could also use a number line or multiplication square to help.