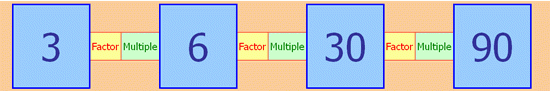
Factor-Multiple Chains

Can you see how these factor-multiple chains work? Find the chain which contains the smallest possible numbers. How about the largest possible numbers?

Age

7 to 11

Challenge level

Primary

Number - Multiplication, Division and Ratio

Properties of Numbers: Multiples, Factors, Primes, Square and Cube Numbers

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

Here is an example of a factor-multiple chain of four numbers:

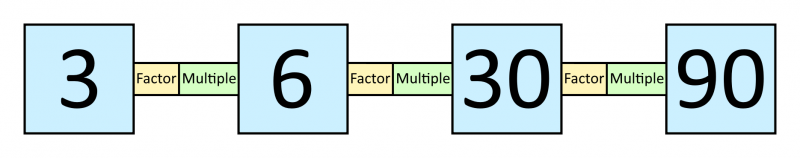
Can you see how it works? Perhaps you could make some statements about some of the numbers in the chain using the words "factor" and "multiple".

In these chains, each blue number can range from $2$ up to $100$ and must be a whole number.

You may like to experiment with this spreadsheet which allows you to enter numbers in each box. Perhaps you can make some more chains for yourself.

What are the smallest blue numbers that will make a complete chain?

What are the largest blue numbers that will make a complete chain?

What numbers cannot appear in any chain?

What is the biggest difference possible between two adjacent blue numbers?

What is the largest and the smallest possible range of a complete chain? (The range is the difference between the largest and smallest values.)

Student Solutions

We had quite a few solutions sent in for this challenge. Many just approached the first two questions and those who tried all five sometimes misread - so be careful! For example the third question says "What numbers cannot appear in any chain?" and many answered by saying what could not start a chain.

First from Chloe and Holly at North Molton:

The solution to Factor Multiple Chains is to find the smallest chain of the factors and multiples is 2, 4, 8, 16 and then the biggest is 5, 25, 50,100.

Then from Haipei at Moorside:

The way to work it out is to find out the pattern linked between the numbers stated to work out the largest and smallest possible combinations.

2, 4, 8 and 16 is the lowest possible combination because 2 is a factor of 4, 8 and 16; and the highest would be 5, 25, 50 and 100 and 5 is a factor of both 25, 50 and 100.

We received three contributions from Grimsargh St Michael. They were from Klara, Olivia and Isabelle offering 2, 4, 8, 16 as the lowest and either 10, 20, 40, 80 or 7, 21, 42, 84 for the largest.

Karim and Prasidhee from Middleton Primary School sent in their excellently explained solution as a Word document: AailaandOthers.doc

They also summarised their work:

Question 1: 2, 4, 8,16 We started from the smallest number and kept on multiplying by 2.

Question 2: 12, 24, 48, 96 We experimented with different combinations until we found the correct chain.

Question 3: You times a number by 4 and if it's above 100 the it can't be possible (that's the answer to the question) this is true but leaves out many others.

Question 4: 2-100 they're the smallest and the highest possible number as 2 times 50 = 100

Question 5: 2, 24, 48, 96 Yet again we experimented with the different chains until we found the correct chain.

Jaeyen St Mary and All Saints sent in the following:

What are the smallest blue numbers that will make a complete chain? 2, 4, 8, 16.

What are the largest blue numbers that will make a complete chain? 12, 24, 48, 96.

What numbers cannot appear in any chain? Any odd numbers after 12 can't appear in the chain because you can't divide an odd number by 2 but 3, 5, 7, 9, 11 are allowed, but only to start the chain. I think you may be able to find others as well!

What is the biggest difference possible between two adjacent blue numbers? 48.

What is the largest and the smallest possible range of a complete chain? (The range is the difference between the largest and smallest values.) Biggest: 12, 24, 48, 96. Smallest: 2, 4, 8, 16.

Mitchell also from St Mary and All Saints sent in this next one and I've added some comments:

What are the smallest blue numbers that will make a complete chain? 2, 4, 8, 16

What are the largest blue numbers that will make a complete chain? 12, 24, 48, 96

What numbers cannot appear in any chain? Any odd numbers after 12 cannot be used because you can't divide odd numbers by 2. This is because an odd number times an odd number equals an odd number. A even number times an even number equals an even number. And an odd number multiplied by an even number is an even number. This is because even beats odd. For example, if you do 11x3 it equals 33. If you do 2x4 it equals 8. And if you do 3x6 it equals 18. In this problem you need to multiply by 2, 4 or 8.This may need further thinking about! Therefore, any odd numbers after 12 can't be used. again I think you may find more.

What is the biggest difference possible between two adjacent blue numbers? 48

What is the largest and the smallest possible range of a complete chain?

(The range is the difference between the largest and smallest values.)

Finally, Aaila and Hamna from Vale View Primary School sent in this extremely systematic solution: AailaandHamna.doc

Well done! You all worked well on this challenge, look out for the next batch of live problems!

Teachers' Resources

Factor-multiple Chains

Here is an example of a factor-multiple chain of four numbers:

Why do this problem?

This problem offers opportunities to reinforce pupils' understanding of factors and multiples. They will become confident in using this vocabulary. It also gives them the chance to justify their solutions and to be creative by making their own chains.

Possible approach

You may wish to show this spreadsheet to the whole class to introduce the problem. It is an interactive chain, which allows you to enter numbers in each box, giving feedback as to when factors and multiples occur. As you make different chains, ask the children to explain what is happening so that everyone fully understands the environment.

Encourage pupils to work on the different questions, ideally in pairs so that they have somone with whom to discuss their ideas. They could work on paper or mini-whiteboards, and access to calculators might be useful. You might like the class to be at computers so they can manipulate the spreadsheet themselves and check their solutions. You may have to discuss what is meant by 'largest' and 'smallest', and come to an agreement. You could ask two questions, for example 'What are the largest blue numbers that will make a complete chain?' and 'What is the largest possible first number in a chain?'.

When sharing solutions, encourage learners to justify their answers - how do they know that their chain contains the smallest/largest numbers etc.? Some children will be using trial and improvement, some will have developed a system for trying numbers in turn, and others may have been able to combine these with their knowledge of number properties.

Key questions

Can any number start the chain? Why/why not?

Can any number be at the end of a chain? Why/why not?

What can you say about the numbers in the second and third positions in a chain?

How will you keep track of what you have tried?

Possible extension

Children could investigate what would happen if the chain was made up of more than four numbers, or made up from a different range of numbers.

Possible support

Enabling learners to manipulate the spreadsheet for themselves will help them access this problem and you can focus on justifying the solutions rather than pupils worrying about obtaining them. There is still a great deal of mathematical thinking taking place as children interact with the spreadsheet.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners